1
$\begingroup$

Flow work and energy for continuous fluid flow is calculated as $Pv$ 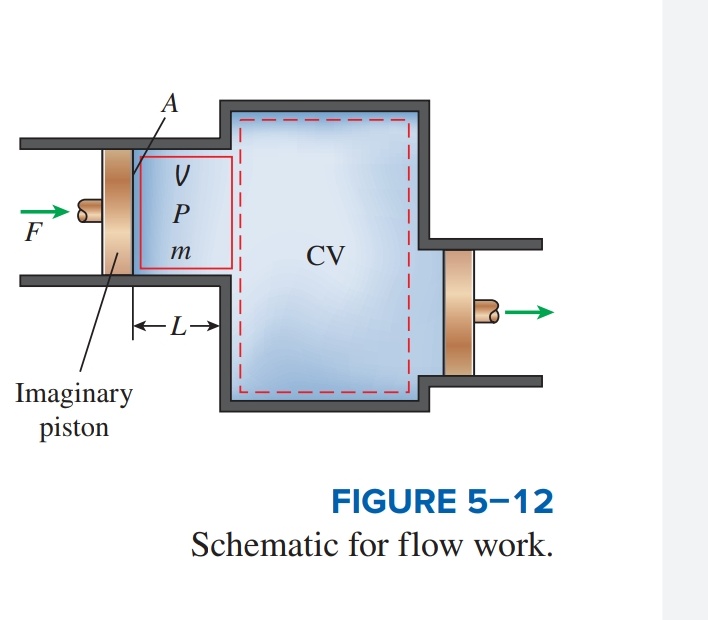enter image description here

This is explained in terms of the rest of the fluid pushing the back of a fluid element in consideration, which is described in terms of a hypothetical piston.

In a solved example involving air flowing uniformly and steadily, my textbook essentially uses the ideal gas law to solve for the rate of energy transport by mass. They write, $h=C_pT$ and $E_{rate}=\frac{dm}{dt}(h+ke)$ since they have consolidated $u+Pv = h$ & neglected gravitational PE, and then they plug in values.

What I don't get is, these calculations are done for a "static" gas. The fact that it's flowing here doesn't seem to be accommodated for. Wouldn't the pressure $P$ used in calculating the flow energy have to include the additional force due to the liquid flowing?

EDIT: Here's how flow energy is defined and explained by my text

enter image description here

And also energy transport: enter image description here

Improve this question
$\endgroup$
4
  • $\begingroup$ What is your definition of flow energy? $\endgroup$
    – Chet Miller
    Commented Jan 20, 2023 at 12:52
  • $\begingroup$ @ChetMiller I'll include this in my post $\endgroup$
    – xasthor
    Commented Jan 20, 2023 at 12:53
  • $\begingroup$ You are aware that the calculation includes $E_{in}-E_{out}$, right? $\endgroup$
    – Chet Miller
    Commented Jan 20, 2023 at 12:59
  • $\begingroup$ @ChetMiller For energy transport by mass? I've just added the section on that to the post for reference too. Edited my post to clarify what I meant by E $\endgroup$
    – xasthor
    Commented Jan 20, 2023 at 13:03

1 Answer 1

Reset to default
0
$\begingroup$

E includes two types of things: 1. Flow of internal energy, kinetic energy, and potential energy entering or exiting the control volume. 2. work to push fluid into or out of the control volume. 2. is lumped together with internal energy from 1 to give the enthalpy term of E.

Improve this answer
$\endgroup$
6
  • $\begingroup$ I get this. My question is when they're calculating h = $C_pT$, at say T= 77°C, and this = Pv + u, the pressure here would be for a static gas. In our case, the gas is flowing, so I feel like that isn't being accounted for with this. $\endgroup$
    – xasthor
    Commented Jan 20, 2023 at 13:36
  • $\begingroup$ P is the force per unit area exerted by the moving fluid on the adjacent moving fluid. To a moving observer,, the interface is static.. You're not referring to viscous contributions to the compressive stress tensor, are you? $\endgroup$
    – Chet Miller
    Commented Jan 20, 2023 at 14:09
  • $\begingroup$ No I'm not referring to the latter. So, the fact that the fluid is moving has no effect? I would imagine that in a moving fluid, the molecules would have an additional velocity component in the direction of the flow, and that would contribute to increased pressure in that direction $\endgroup$
    – xasthor
    Commented Jan 20, 2023 at 14:12
  • $\begingroup$ No. Not as measured by an observer moving at the interface velocity. P is what we call the static pressure. $\endgroup$
    – Chet Miller
    Commented Jan 20, 2023 at 14:20
  • $\begingroup$ What about if the fluid was accelerating? In this case the $P$ in the $Pv$ term for flow energy wouldn't be that calculated by an equation of state right? $\endgroup$
    – xasthor
    Commented Jan 20, 2023 at 14:28

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.