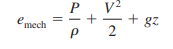I'm having a hard time understanding what the difference between flow energy and kinetic energy in a fluid that moves. My book gives me the following equation for mechanical energy of flowing fluid:
Now the two last terms is easy to understand for me, but I don't see the necessity for the first term. Surely all the energy tied to the fluids movement is contained in the kinetic energy of the fluid?
If possible I would really appreciate an intuitive answer.
You can consider pressure as energy density. Note:
$$P=\frac{F}{A}=\frac{Fd}{Ad}=\frac{W}{V}$$
When flow encounters a restriction, the pressure, and thus pressure energy, drops. This may seem counter intuitive until you realize that at the same time the flow velocity and thus kinetic energy increases at the restriction.
For a given elevation, neglecting friction losses, conservation of energy requires any increase in kinetic energy in the flow equal any decrease in pressure energy in the flow due to the flow work done per unit volume pushing the fluid through the restriction.
Hope this helps.
Consider a small moving mass $dm$ crossing a fixed boundary $L$. Work ${p \over \rho} dm$ is required to push the mass across the boundary. ${p \over \rho}$ is the work per unit mass. This is the Lagrangian viewpoint, following a fixed mass.
Consider mass flowing into a fixed region. This is the Eulerian viewpoint, following a fixed region, and the system is called an open thermodynamic system. From this viewpoint, ${p \over \rho}$ is called flow energy.
This is why in the first law of thermodynamics for mass entering/leaving an open system, The enthalpy, $h$ of the flowing masses is considered, where $h = u + {p \over \rho}$, $u$ being the internal energy of the flowing fluid.
Your relationship for mechanical energy is used in the Bernoulli equation, which is an approximation of the first law for an incompressible fluid with negligible changes in internal energy. Sometimes the Bernoulli equation includes terms for work done (e.g. by a pump) and the "head" loss due to friction to simply account for change in internal energy. The Bernoulli equation is not true in general, for example where there are large changes in the temperature of the fluid.
See a good book on thermodynamics such as Obert, Thermodynamics. For a detailed development see Transport Phenomena by Bird, Stewart, and Lightfoot.
This term is defined for a flowing fluid. Your perspective is somewhat as though you are standing inside the fluid and wanting to consider all forces (kinetic, potential, and pressure) on the fluid contributing to energy density. You are able to account for the kinetic and potential energy terms for that fluid, but you wonder about the pressure term. Stand instead inside a static control volume, allow the fluid to flow through it, and consider the energy balance.
The energy balance equation (conservation of energy equation) over the static control volume is stated using internal energy $\tilde{U}$, kinetic energy $\tilde{E}_K$, and potential energy $\tilde{E}_p$, each here expressed per unit mass. Within the defined control volume, we write terms for flow in/out, change with time, and heat + work as
$$\iint_{CS} \left( \tilde{e} + \frac{p}{\rho}\right) \rho \left(\vec{v}\bullet\vec{n}\right) dA + \frac{\partial}{\partial t} \iiint_{CV} \rho\ \tilde{e}\ dV = \dot{q} - \dot{w}$$
with
$$\tilde{e} \equiv \tilde{U} + \frac{v^2}{2} + gz$$
The first integral is over the control surface (CS). It accounts for energy flow into and out of the system through its boundary. The second integral is over the control volume (CV). It accounts for energy change inside the volume as a function of time (the derivative).
A closed system would not consider the first term because no mass flows into or out from the control surface. Notice then that we have no consideration for the $p/\rho$ term. Heat or work flow change either the internal, kinetic, or potential energy of the control volume with time.
For an open system, the mass that flows into or out from the control volume carries energy density with it. The first energy density is the internal + kinetic + potential as for any control volume. We include an additional energy density term for potential to do $pV$ work as the fluid packet moves through the control volume. We translate $pV$ work into an energy density using
$$\frac{pV}{m} = p\tilde{V} = \frac{p}{\rho}$$
This is not work to ''push'' fluid into or out of the control volume. This is a capacity for the fluid packet to expand or contract as the it passes through the control volume. If the term $p/\rho$ is the same at the exit as the entrance, the fluid packet has neither expanded nor contracted as it passes through the control volume.
Using your definition, we obtain
$$\tilde{e} = \tilde{U} + \tilde{e}_{mech}$$
Finally, consider a steady state, adiabatic process. The time derivative and heat flow terms are zero. Allow the fluid to have the same entering and exit velocities and heights. We obtain
$$\iint_{CS} \left(\frac{p}{\rho}\right) \rho \left(\vec{v}\bullet\vec{n}\right) dA = - \dot{w}$$
The static control volume will expand or contract according to the change in the $p/\rho$ term going into and out of the control volume.
