What does the $\Delta x $ in Heisenberg uncertainty principle actually mean?

Question

In the textbook ''Concepts of Modern Physics -Arthur Beiser'' in chapter 3 section 3.7 where the book talks about the uncertainty princple

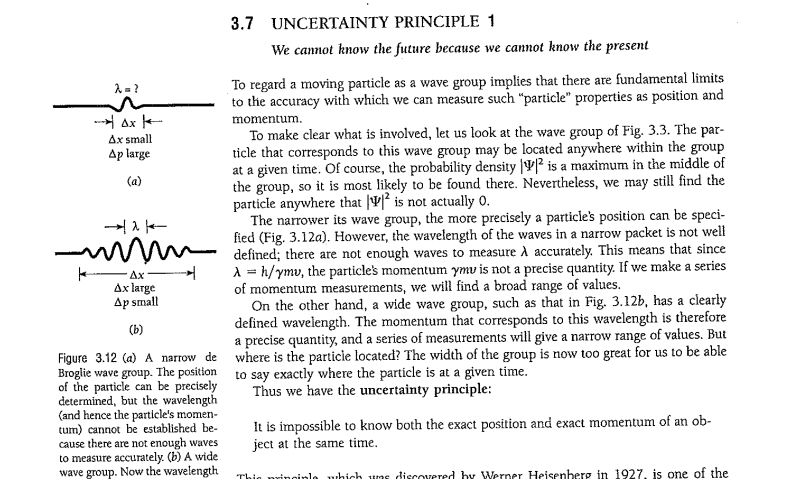
While illustrating the physics behind the uncertainty principle ''I will put an image from the book'' he began talking about the $\Delta x$ and defined it as the width of wave group associated with the particle,, and this is logical as the width of wave group increase the less precise we know it's position

but while deriving the famous formula which is :

\begin{gather*} \Delta x\Delta p\ge \frac{h}{4\pi} \end{gather*}

he said that $\Delta x $ is the standard deviation of the ${\Psi}(x)$

this is what my confusion about ,,is the $\Delta x $ the width of wave group represented by ${\Psi}(x)$ or the standard deviation ${\Psi}(x)$? and why we began by talking about it by the width of wave group of the wave function ${\Psi}(x)$ and then we said it represent the standard deviation of ${\Psi}(x)$? and what does mean by saying that the $\Delta x$ is the uncertainty what do mean by uncertainty?

Answer 1

Formally, $\Delta x$ here means the standard deviation in $x$, which can be computed given the wavefunction $\Psi(x)$ as (I'll assume we're in one spatial dimension) \begin{equation} \Delta x^2 = \langle x^2\rangle - \langle x \rangle^2 = \left[\int dx \Psi^\star x^2 \Psi \right] - \left[\int dx \Psi^\star x \Psi\right]^2 \end{equation}

However, intuitively, given a wavepacket, $\Delta x$ indeed corresponds to the spatial width of the wavepacket. It is not too difficult to check this analytically for a Gaussian wavepacket \begin{equation} \Psi(x) = \frac{1}{\left(2\pi \sigma^2\right)^{1/4}}e^{-(x-x_0)^2/(4\sigma^2)} e^{i k x} \end{equation} using the formula for $\Delta x^2$ given above.