Deriving time dilation from a moving photon clock [closed]

Question

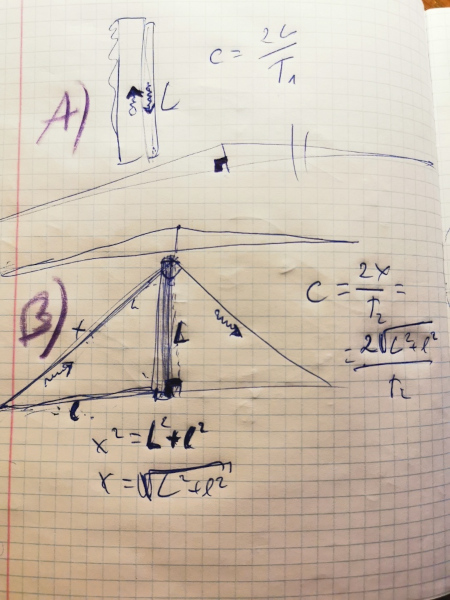
This is my attempt of simple derivation of time dilation from a moving photon clock. Consider this schematics:

A) is photon clock as seen from a photon clock reference frame (or as by observer outside when photon clock would be stationary)

B) is when photon clock moves, and is seen by observer outside of photon clock reference frame.

According to A) in one clock tick (1 period) photon moves distance $2L$ (height of clock), so photon speed is :

$$ c=\frac {2L}{T_1} \tag {1},$$ where $T_1$ is tick duration in photon clock reference frame.

According to B) photon travels per 1 tick distance $2x$, so by outside observer photon speed is : $$ c= \frac {2x}{T_2}, \tag {2}$$ Where $T_2$ is time duration of 1 tick as is seen from outside reference frame.

Now because light speed is the same in all reference frames, we can equate :

$$ \frac {2L}{T_1} = \frac {2x}{T_2} \tag 3 $$

According to Pythagoras theorem, $$ x= \sqrt{L^2+l^2} \tag 4 $$

Substituting (4) into (3), and expressing tick duration ratios, we get :

$$ \frac {T_2}{T_1} = \frac {\sqrt{L^2+l^2}}{L} \tag 5$$

Multiplying in RHS numerator and denominator by $1/L$ we get :

$$ \frac {T_2}{T_1} = \sqrt {1 + \frac {l^2}{L^2}} \tag 6$$

Acknowledging that $l$ is distance traveled of photon clock moving forward with speed $v$ per half of proper tick $T_1/2$, and similarly - $L$ is distance traveled by photon in own reference frame per same half of proper tick. So we can re-write (6) into :

$$ \frac {T_2}{T_1} = \sqrt {1 + \frac {(v~T_1/2)^2}{(c~T_1/2)^2}} \tag 7 $$

After simplification (7) becomes,

$$ \frac {T_2}{T_1} = \sqrt {1 + \frac {v^2}{c^2}} \tag 8 $$

What I've got is "something a bit like" a Lorentz factor. From the wiki is seems that there's an alternative forms of Lorentz factor like : $$ \gamma ={\sqrt {1+\left({\frac {p}{m_{0}c}}\right)^{2}}} \tag 9,$$

but I'm not sure if equation (8) can be reduced to (9) or vise-versa. So it's more probably that I've made an error somewhere in derivation or in concepts/ schematics.

Question is,- Does (8) equation valid and if not - Where I have made an error and How to derive correct Lorentz factor for time dilation from a moving photon clock ?

Answer 1

The mistake I see is in (7), you put in $T_1$ when it should be $T_2$, because

$$v=2l/T_2$$

Your equation (7) should be

$$\frac {T_2}{T_1} = \sqrt {1 + \frac {(v~T_2/2)^2}{(c~T_1/2)^2}} $$

If you then solve for $$T_2/T_1$$ you should get the correct Lorentz factor.

Answer 2

Lorentz Factor was long before using it by Einstein there for other proposes.

there are 2 questionable approaches to deriving Lorentz transformation from 1905 and 1916 by Einstein

following I'd show a better and more comprehensible approach. If we assume t′ and x′ as linear functions of t and x , we can write: t'=at+bx,(1) x'=ct+dx.(2) or Δt'=aΔt+bΔx,(3) and Δx'=cΔt+dΔx.(4) from (2) if x′=0 , x=−ct/d , so −c/d=v , and if x=0 , x′=ct , and from (1) t′=at , so x′=ct′/a , and c/a=−v , so from the definition of relative velocity we get d=a ,and c=−av , x′=ax−avt(6) t′=at+bx(5) and now if two snapshots from 1-meter-rods in two inertial system are equal, so from (6) at t=0 if x′=1x=1/a and from (5) at (t′=0 if x=1 ), at=−b and from (6) x′=a+bv and acc. to the relativity x=x′ , this results b=(1/a−a)/v , and we get the general transformation equations without gamma. x′=a(x−vt)(7) t′=at−[(a−1/a)/v]x(8) If a=1 you get Galileo Transformation, and if x=ct , x′=ct′ then you get the LT. but time dilation and length contraction can be found in general form too, and these are not specific properties of STR.

Atomic clocks example is an obvious trick. In 1905 in "On the Electrodynamic of Moving Bodies" Einstein doesn't speak of the real slowing of clocks in the moving system. He has said only if the time definition was correct, then the time at the moving system would be shorter than the observer's, seen from the observer's point of view.