Twin Paradox - Why am I missing 0.2 years?

Question

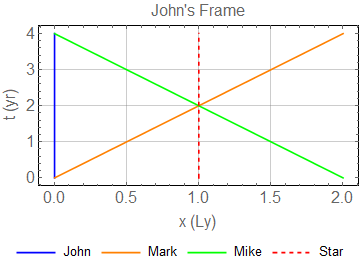
The situation: we have Earth, a star and an exoplanet, all in one line. The star is between the Earth and the exoplanet and 1 light year away from each.

At the start of this thought experiment, Mark and John are on Earth, while Mike is on the exoplanet. Mark gets in his spaceship and moves to the star at 0.5c. At the same time, Mike gets in his spaceship and also moves to the star at 0.5c, such that Mark and Mike come at the star simultaneously. Mark records the time it took him to get to the star, and communicates it to Mike when they pass each other. Mike travels on to Earth, records the time it took him to get from the star to Earth, and adds the duration Mark gave him.

From John's perspective, Mark's trip to the star took $$\frac{1\;light\;year}{0.5c}=2\;years$$ and Mike trip from the star to Earth also took 2 years.

Of course, due to time dilation, John expects Mark and Mike to age $$2*\sqrt{1-\frac{0.5c^2}{c^2}}=2\sqrt{\frac{3}{4}}\;years$$ during these trips, for a total of $$4\sqrt{\frac{3}{4}}\;years$$ So far so good.

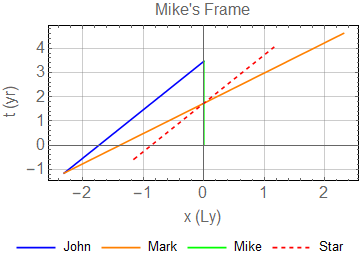
Now let's look at things from Mike's perspective. From his perspective, the star moves towards him at 0.5c and Mark moves towards him at $$\frac{0.5c + 0.5c}{1 + \frac{0.5c\;*\;0.5c}{c^2}} = 0.8c$$

Which means that from Mike's perspective, Mark closes in on the star with 0.3c. So in order form Mark and Mike to arrive at the star at the same time, Mike has to leave later than Mark. Call the time Mike waits t1, and the time he takes to get to the star t2. Note that during t1, Mark closes in on the star with 0.5c (since the star is standing still), and during t2, with only 0.3c. Also, during t1, Mike perceives the distance between Mark and the star to be 1 (no length contraction yet).

Therefore, the distance between Mark and the star after t1 has passed equals 1 - 0.5 * t1 light years. And this remaining distance, length contracted, must be equal to 0.3 * t2:

$$0.3 * t2 = (1 - 0.5 * t1) * \sqrt{\frac{3}{4}}$$

t2 is also simply the time it takes Mike to get to the star:

$$t2 = \frac{\frac{3}{4}}{0.5c} = 2\sqrt{\frac{3}{4}}\;years$$

Which means

$$t1 = 0.8\;years$$

In other words, Mike waits 0.8 years, flies to the star, meets Mark exactly at the star and flies to Earth. Mark has aged t2 during his trip; Mike ages t2 during his trip from the star to Earth. Adding these 2 numbers together gives

$$2\sqrt{\frac{3}{4}} + 2\sqrt{\frac{3}{4}} = 4\sqrt{\frac{3}{4}}\;years$$

In complete agreement with John!

But how much has John aged, according to Mike?

During t1, John was stationary according to Mike and therefore aged t1. During t2 + t2, John was moving at 0.5c, so aged

$$(2\sqrt{\frac{3}{4}} + 2\sqrt{\frac{3}{4}}) * \sqrt{\frac{3}{4}} = 4 * \frac{3}{4} = 3\;years$$

So in total, John aged

$$0.8 + 3 = 3.8\;years$$

from Mike's perspective?! That can't be correct. The thing is, no matter how I do the calculations, I come out at 3.8 years. Where are those 0.2 years I am missing?

Answer 1

At the same time, Mike gets in his spaceship and also moves to the star at 0.5c, such that Mark and Mike come at the star simultaneously.

Be careful here. There is no universal notion of "at the same time" for events which occur at different points - see the relativity of simultaneity. Specifically, Mark and Mike's departures may be simultaneous according to John, but then they won't be simultaneous according to Mike or Mark.

As is often the case, the problem is made easier through the use of a spacetime diagram.

Recall that the proper time along a segment of a straight worldline between the points $(t_1,x_1)$ and $(t_2,x_2)$ is given by $\Delta \tau = \sqrt{\Delta t^2 - \Delta x^2/c^2}$. In John's frame, the proper time along the first segment of Mark's worldline is $\sqrt{2^2 - 1^2} = \sqrt{3}$ yr, and the same is true along the second segment of Mike's worldline, and so the number Mike reports to John is $2\sqrt{3}$ years, as you say.

In Mike's frame, Mark started the journey early, at time $t_{Mike}=-2/\sqrt{3}$ yr, from the point $x_{Mike} = -4/\sqrt{3}$ Ly. He traveled until intersecting Mike's worldline at $t_{Mike}=6/\sqrt{3}$ yr and $x_{Mike}=0$. Therefore, the proper time along John's worldline is $\Delta \tau = \sqrt{(8/\sqrt{3})^2-(4/\sqrt{3})^2} = 4$ yr, as expected.

---

I suspect the problem enters into your analysis when you talk about the various observers waiting before they start to move. This implies that they are not inertial - their worldlines will not be straight. Instead, they will have little "kinks" in them which occur in different places depending on whose frame you're in. Put differently, "Mike's perspective" therefore cannot be encapsulated in a single (inertial) frame (or spacetime diagram).

Answer 2

This question already has an excellent answer, but I figured I'd post my own corrected calculations too.

My main mistake (as pointed out by J. Murray in the accepted answer) is having Mike wait from his perspective. That makes his trip nonlinear.

If we assume both Mark and Mike were already in motion and arrive at the star simultaneously from both John's and Mike's perspective, we can see that from Mike's perspective, Mark takes more time to arrive at the star than Mike does. That's because Mark's speed is 0.8c, and the star's speed is 0.5c (according to Mike). Therefore the star closes in on Mike with 0.5c, but Mark closes in on the star with 0.8c - 0.5c = 0.3c.

So from Mike's perspective, Mark takes $$\frac{\sqrt{\frac{3}{4}}}{0.3c} = \frac{5\sqrt{3}}{3}\; years$$ to get to the star.

In that time, John aged $$\frac{5\sqrt{3}}{3} * \sqrt{\frac{3}{4}} = 2.5\;years$$ As shown before, Mike takes $$2\sqrt{\frac{3}{4}}\; years$$ to get from the star to John. In this time John ages $$2\sqrt{\frac{3}{4}} * \sqrt{\frac{3}{4}} = 1.5 \; years$$ So in total, John aged 2.5 + 1.5 = 4 years from Mike's perspective.