1
$\begingroup$

I've recently done an experiment where I analyzed a mass moving in circular motion around a point with a spring attached to the mass on one end and to the point around which the mass moves on the pother end. This system was set on top of an air table to reduce friction as much as possible and it was recorded using a camara set on top of the table.

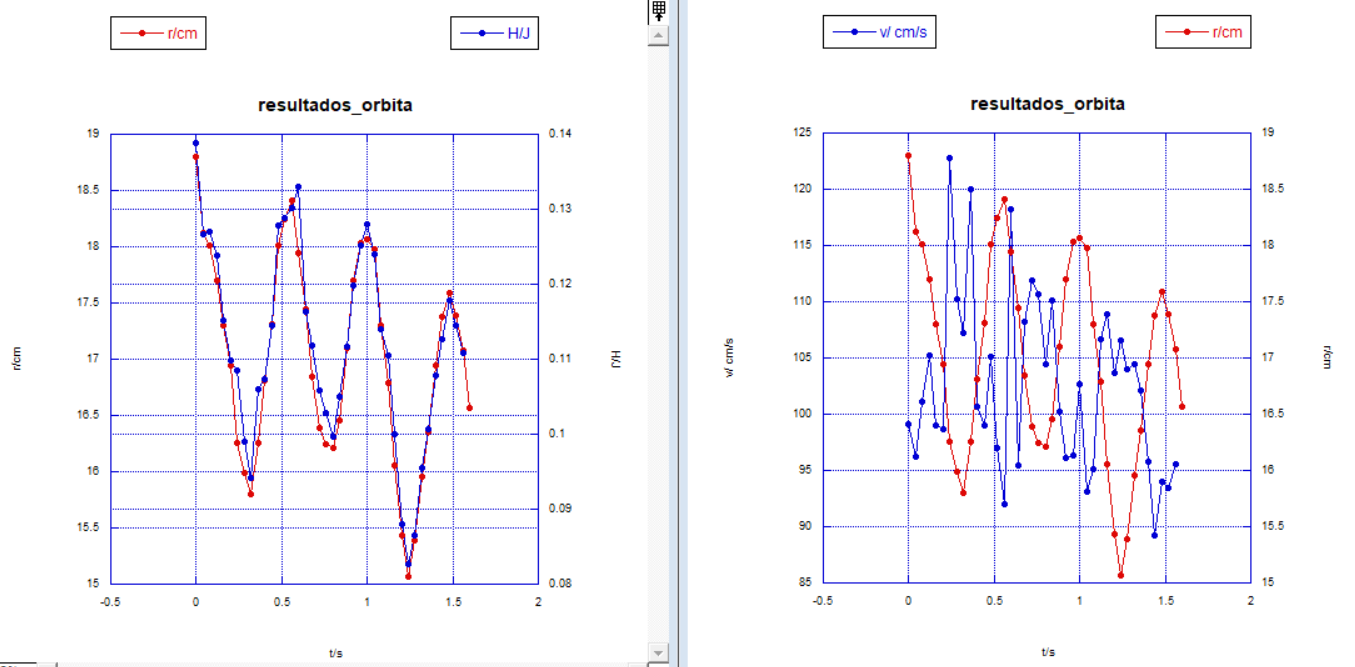
After analyzing the video using the program Tracker. Then, using Kaleidagraph I got the polar coordinates as well as the time derivatives of the radius and the angle. With this data, I got the hamiltonian of the system and it doesn't remain constant.

According to the theory, as the holonomic constraints are stationary in this system then the hamiltonian should be exactly equal to the mechanical energy which should remain constant. Instead, what I get is some oscilating value which decreases.

The fact that it oscilates downwards makes sense as this could be attributed to a friction force we are neglecting. However, it doesn't make sense that the energy of the system oscilates. In fact, this oscilation correlates with the oscilation of the radius (the distance between the point the mass orbits and the mass). This correlation makes me think that there could be some reason for why the energy doesn't remain constant but I still can't explain why this happens.

The graph that correlates r and H on the left and r and v on the right

One think I've thought of while representing some of the variables is that the velocity of the system could have something to do with the change in the hamiltonian not from a physics point of view but more on a technical way. As when the velocity is larger the camara couldn't capture the image properly and thus could create some error.

Other than this last interpretation I have no idea why the energy would change and why this change would be correlated to the change in r.

If anyone had some insights on why this happens I would appreciate the help.

Sorry for any bad grammar or spelling, english is not my first language.

Improve this question
$\endgroup$
6
  • $\begingroup$ $r(t)$ must be periodic, if not the Hamiltonian is not conserved $\endgroup$
    – Eli
    Commented Oct 19, 2022 at 16:30
  • $\begingroup$ this is your Hamiltonian $~\frac 12\,m \left( \left( r \left( t \right) \right) ^{2} \left( {\frac { d}{dt}}\varphi \left( t \right) \right) ^{2}+ \left( {\frac {d}{dt}} r \left( t \right) \right) ^{2} \right) +\frac 12\,k \left( r \left( t \right) \right) ^{2} $ $\endgroup$
    – Eli
    Commented Oct 19, 2022 at 16:39
  • $\begingroup$ @Eli I don't really understand the question. My $r$ in theory should be as close to a circle and thus be constant over time. However, as we couldnt get the mass to move only giving it a tangential initial velocity, the radius changes over time. Also, shouldn't the term on the right be $\frac{1}{2}k(r(t)-l_0)^2$ $\endgroup$
    – Mikel Solaguren
    Commented Oct 19, 2022 at 19:47
  • $\begingroup$ r is not a constant. if r is a constant the sprint force is zero. r(t) must be periodic otherwise the energy (Hamiltonian) is not conserved. you can add a preload to the spring ($~l_0\ne 0~)$ but this term dose not change the Hamiltonian. $\endgroup$
    – Eli
    Commented Oct 19, 2022 at 19:56
  • $\begingroup$ OP mentions Hamiltonian, but it actually seems to be energy. $\endgroup$
    – Qmechanic ♦
    Commented Oct 19, 2022 at 20:09

2 Answers 2

Reset to default
1
$\begingroup$

However, it doesn't make sense that the energy of the system oscilates.

This just means that there is some potential that is being neglected in your analysis. As energy goes into that potential it leaves the parts of the system that you are analyzing and as energy leaves that neglected potential then it goes back into the analyzed parts.

In fact, this oscilation correlates with the oscilation of the radius (the distance between the point the mass orbits and the mass).

Either your spring constant is wrong or there is some other elastic part of the system that is not included in the analysis. You can just use an "effective" spring constant and adjust it until the oscillation is minimized. That will give you an idea of how large the un-measured effect is and you can have a "reality check" to see if it is reasonable that the errors may be that large.

Improve this answer
$\endgroup$
2
  • $\begingroup$ One question, if i used an ideal spring constant and added the term $U_i=\frac{1}{2}k(r-l_0)^2$ to the total energy, this would leave me with something like $E=\mathcal{H}+W_F+U_i$. Now, as the hamiltonian increases energy as the redius gets larger wouldn't the "hidden potential" $U_i$ has to take energy as the radius decreases. This would mean that I've overestimated y $k$ right? $\endgroup$
    – Mikel Solaguren
    Commented Oct 20, 2022 at 10:29
  • $\begingroup$ @MikelSolaguren I think you could be right. You may have overestimated k. It is also possible that the spring is significantly non-ideal so it needs a term that is proportional to r $\endgroup$
    – Dale
    Commented Oct 20, 2022 at 11:50
1
$\begingroup$

Any deviation from the assumptions produces (or may produce) deviation in the results, from the expected ones. To list some of them:

  • "imperfect" boundary condition and constraints
  • friction
  • wrong measurements of the mass
  • wrong measurements of the constitutive law of the spring and its parameters (if the behaviour of the spring is approximately linear, this means the elastic constant of the spring)
  • uncertainty in the position measurement
  • uncertainty in the velocity measurement

As a methodological observation, when you plot your experimental measurements, you need to add the uncertainty bar over the measured quantities, and you can compute them with uncertainty propagation (e.g. by means of RSS) from the parameters, as independent variables, to the results, as the dependent variables.

Anyway, given the good correlation of the variation of the Hamiltonian with the distance $r$, it seems likely that you're overestimating the stiffness of the spring.

Just a question, maybe a stupid one: did you evaluate the Hamiltonian using the magnitude of the velocity, didn't you?

Improve this answer
$\endgroup$
4
  • $\begingroup$ I don't really understand your question. To evaluate the Hamiltonian I used an expression $\mathcal{H}=f(r, \dot{r}, \dot{\theta}, m, k, l_0)$, $\dot{r}$ and $\dot{\theta}$ beng the numerical derivatives of the experimental values $r$ and $\theta$. But for the kinetic energy term I use $\dot{r}^2+r^2\dot{\theta}$ which is equal to $v^2$ $\endgroup$
    – Mikel Solaguren
    Commented Oct 19, 2022 at 14:43
  • $\begingroup$ $\dot{\theta}^2$ in your expression here. Anyway, I wanted to be sure you didn't miss some term there $\endgroup$
    – basics
    Commented Oct 19, 2022 at 14:51
  • $\begingroup$ Anyway, what's the mass of your spring, and what's the mass of your mass? $\endgroup$
    – basics
    Commented Oct 19, 2022 at 14:54
  • $\begingroup$ I didn't meassure the mass of the spring but the mass of th mass is 35g. The thing with this experiment in particular that is different from what we usually do is that we were given all the measurements by the professor instead of taking them ourselves. This includes the resting length and the constant of the spring as well as the mass $\endgroup$
    – Mikel Solaguren
    Commented Oct 19, 2022 at 15:02

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.