Determining Brillouin Zone for a crystal with multiple atoms

Question

The Brillouin Zone (BZ) refers to a region of reciprocal space corresponding to the primitive cell. That is, a Brillouin Zone is a subset of the reciprocal space which contains all the information necessary to describe the crystal.

In the case of a crystal which only has a single type of atom, the procedure for determining the corresponding BZ is simple:

1. Take the lattice in the real space and convert it to the recirpocal space

2. Choose any one lattice site as the origin and draw lines connecting it to all of its nearest neighbours

3. Draw the perpendicular bisectors of these connecting lines. The area bounded by the bisectors is the first Brillouin Zone

Wikipedia has a very clear illustration of the process.

---

It is further possible to find the Irreducible part of the Brillouin Zone (IBZ), which is the smallest possible subset of the BZ after reducing it along its symmetries in the same ways as the symmetries present in the point group associated with the lattice.

---

The Problem:

The above formulation is clear and well-known. However, I am not very sure about what to do if the crystal has more than one atom. There can be several possibilities:

1. We could treat the question as purely concerning the lattice and treat all the atoms as if they were identical. Then follow the earlier process.
2. We could treat the crystal as having a motif consisting of groups of the different atoms. Then, try to obtain a resulting lattice and carry out the standard process on it.
3. Connect nearest neighbours using sets of either only identical or only dissimilar atoms and continue the earlier process.

---

Unfortunately, none of these options seems obviously correct. It feels like there should be a clear answer based on the underlying theory, but I have had no luck finding it so far.

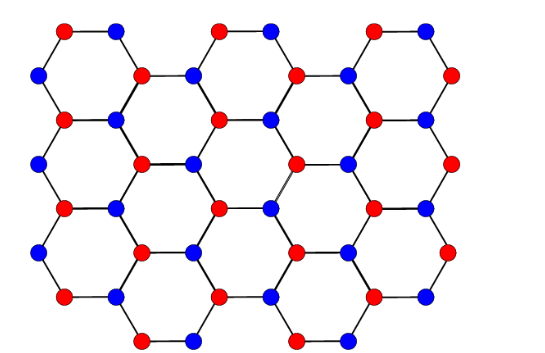
What is the correct way to find the Brillouin Zone/ Irreducible Brillouin Zone for a crystal cconsisting of more than one type of atom? (such as the one shown below)

Answer 1

Lattice and crystal structure are two different things.

A crystal structure is a convolution of a (Bravais) lattice with a basis (an atom or a group of atom).

A lattice is a collection of geometrical points (not atoms) that have the same geometrical properties. Your picture represents a structure with two different kinds of atoms (blue and red), not a lattice. The orientation of the blue points around a red point is different that of red points around a blue point. In this case the lattice point are located at the centre of the hexagons and the basis is constituted of 1 blue atom plus 1 red atom (1/3 of each of the 3 blue atoms at vertexes + 1/3 of each of the 3 red atoms at vertexes).

So your reciprocal lattice is obatined by the direct lattice, independently on the associated basis.

Answer 2

Option 2 is essentially the correct approach. Just as in the your Bravais lattice example, you begin by writing down the lattice vectors $\mathbf{a}_i$ of the real-space lattice. The lattice vectors specify the distance you have to go for the motif (in the case of your honeycomb lattice it consists of two sites, one blue and one red) to repeat itself. Then determine the reciprocal space lattice vectors $\mathbf{b}_i$, which specify the periodicity of the lattice in $\mathbf{k}$-space and thus the size of the Brillouin zone. In the case of the honeycomb lattice, you'll find a hexagonal reciprocal lattice, for which you can then determine the first Brillouin zone following the approach you describe.