Relationship between net torque and net force in constrained systems

Question

If the movement of the rigid body is "constrained", let's say a ladder leaning against a wall, would net torque $= 0$ always imply net force $= 0$?

In most physics questions, torque and translational forces are two separate entities usually independent of one another. But for the example of a leaning ladder, I can't really imagine the case where $\sum F \neq 0$ but $\sum \tau = 0$ (or vice versa) as a non-zero net force would imply that the ladder has to rotate, as the 2 ends are constrained to move along the walls. Is there anything wrong with this line of reasoning?

Answer 1

No, since you can have loading combinations that produce no motion.

Specifically for the ladder problem, consider the kinematics.

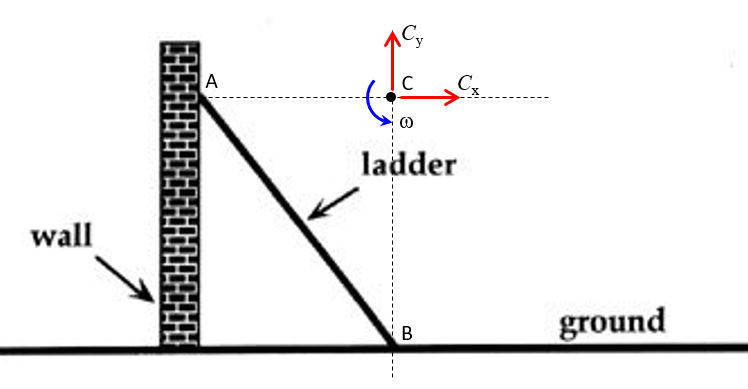
Points A and B are only allowed to slide, which means the center of rotation of the ladder is point C.

As a result, any force that goes through point C will not produce any motion. For example, the normal forces at A and B, marked above as $C_x$ and $C_y$ are such forces.

The net torque about C will indicate if there is rotational equilibrium here, and it might be net zero or not.

If it is not, that means the center of mass is accelerating (since motion occurs) which also means that net force is not zero, since $\sum F = m a$.

---

To find if a force will produce motion or not, consider the kinematics first. Find the power $P = \vec{F} \cdot \vec{v}_A + \vec{\tau}_A \cdot \vec{\omega}$ on an arbitrary point A, and if it is zero, then the force is a constraint force and adds no power to the system.

Take point C above, then kinematics state that $\vec{v}_C = 0$ but $\vec{\omega } \neq 0$. This means that any non-zero arbitrary force $\vec{F} \neq 0$ that produces net torque about C of zero $\vec{\tau}_C = 0$ is going to be a constraint force.

$$ \require{cancel} P = \vec{F} \cdot \cancel{ \vec{v}_C } + \cancel{\vec{\tau}_C} \cdot \vec{\omega} = 0 $$

Answer 2

If the movement of the rigid body is "constrained", let's say a ladder leaning against a wall, would net torque =0 always imply net force =0?

Not always, no. Many different sorts of constraints are possible, and with some you could have cases where you get a translational acceleration without a rotational acceleration.

However, in this specific case the constraints are such that there is no translation without rotation. So your reasoning is correct for this specific case, but not in general.