Conservation of linear momentum in a rotating moon

Question

Imagine a car at the surface of a moon that accelerates forward. As the car pushes on the ground to move, the moon starts to rotate in the opposite direction. However, at any given instant the car has some linear momentum, in the direction tangent to the moon surface at where it is, but the moon does not have any linear momentum due to rotation. In the image below it can be seen that for any particle on the moon there is another one diametrically opposite with opposite linear momentum (represented in purple), which means that the rotation does not contribute to the total linear momentum.

The only way for the linear momentum to be conserved is if the center of mass of the moon has some linear momentum opposite to that of the car. However, is this possible? If the car exerts a force on the moon only tangent to its surface, how can its center of mass accelerate?

Answer 1

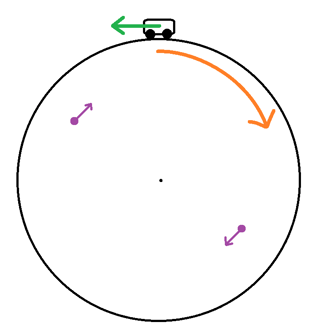
"...but the moon does not have any linear momentum due to rotation"

This is not correct. Why?

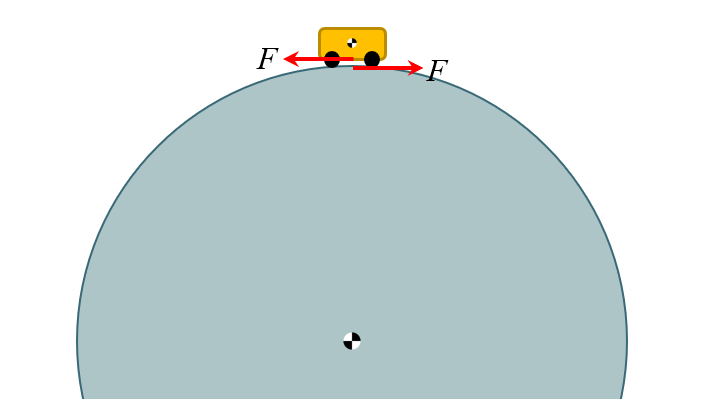
At the moment a tractive force is applied through the wheels, and an equal and opposite force is applied on the moon. Newton's 3rd law still applies.

This force accelerates the center of mass of the car and the moon. Both acquire linear momentum.

In fact, total linear momentum is conserved here.

When you consider the details, you will see the car will orbit around the common center of mass (blue cross below) while at the same time the planet will also orbit around the center of mass at a smaller distance.

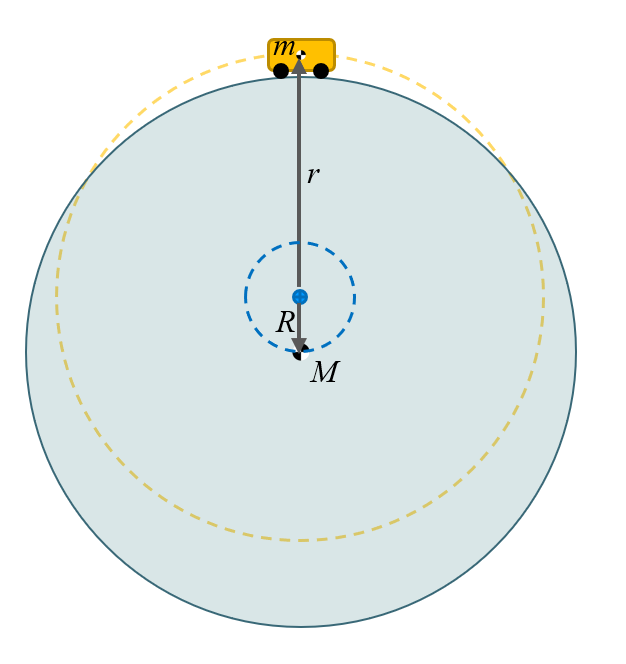
_{Above $R$ is shown as a negative value.}

If the car of mass $m$ is orbiting at distance $r$, and the planet of mass $M$ is orbiting at a distance $R$ then you can say that

$$ m\, r + M\, R = 0$$

This combined center of mass remains fixed (at the origin) and is an internal reference frame as no external forces are applied to the system.

As the car accelerates going faster around its orbit, the planet must counter accelerate also increasing its orbital speed also. Both objects must remain diametrically opposed with respect to the common center of mass in order to maintain the above $m\,r + M R = 0$ relationship.

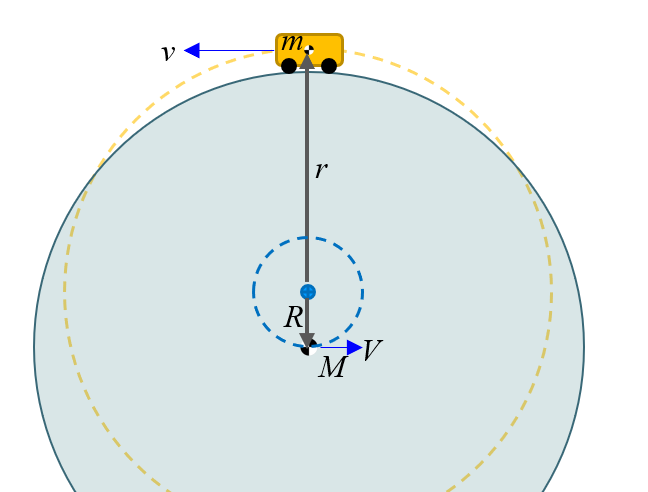
If at some time the car has velocity $v$, then the planet must have velocity $V$ in the opposite sense such that

$$ m v + M V = 0 $$

which is interpreted as the total momentum remains constant (at zero).

In terms of accelerations, if the acceleration of the car is $a = \frac{F}{m}$ and the acceleration of the planet is $A = \frac{-F}{M}$ then the above is

$$ \begin{gathered} m a + M A = 0 \\ m \frac{F}{m} + M \frac{-F}{M} =0 \\ F - F = 0 \end{gathered}$$

Which is exactly Newton's 3rd law.

You might decide to do a more rigorous analysis based on the following free body diagram

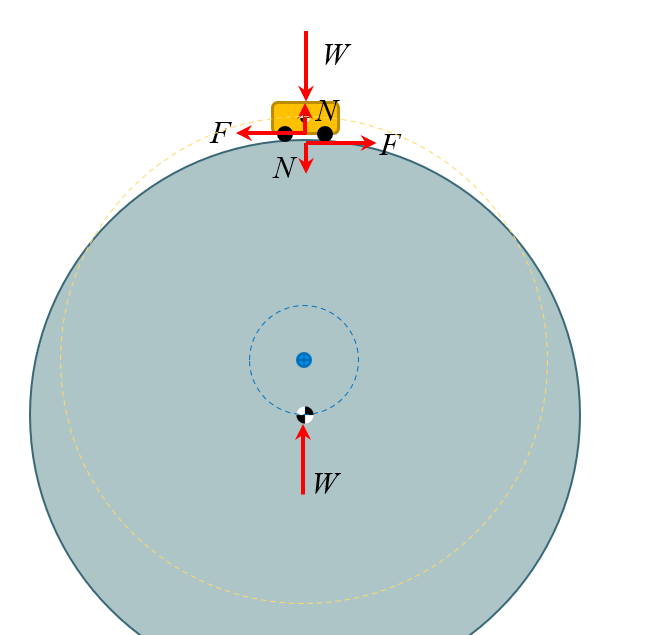
but remember that both objects orbit, and thus both have centripetal acceleration equalling $\frac{v^2}{r}$ and $\frac{V^2}{R}$ respectively. Then you can do the balance of forces in the x and y directions to see for yourself how the system behaves.

Answer 2

The centre of mass of the moon is irrelevant except for calculating the force of gravity. It is the total center of mass, car+moon, that is important in calculating kinematic variables for a system of two bodies. The following simple argument may help.

For the car to move in the direction shown with the green arrow in your plot, the ground is pushed by a frictional force in the opposite direction. As incremental (v a vector) momentum of car is $mv$, the moon is pushed back by incremental -2 $mv$ , for conservation of momentum , this should equal $Mv^{'}$, M the mass of the moon and $v^{'}$ its velocity from momentum conservation. The mass of the moon is practically infinite with respect to the mass of the car, so the velocity of the moon would be very small, so nothing really moves except the car.

If the moon had the same mass as the moving object, this would result in a rotation of the moon about the center of mass.