Movement of a rod submitted to a shifted torque

Question

Suppose I have a rod of mass m initially at rest, for which a torque (F1, F2) is applied at the right side during a brief time $\Delta t$, as in the schema below. The rod is not fixed to anything and not submitted to gravity or any other forces.

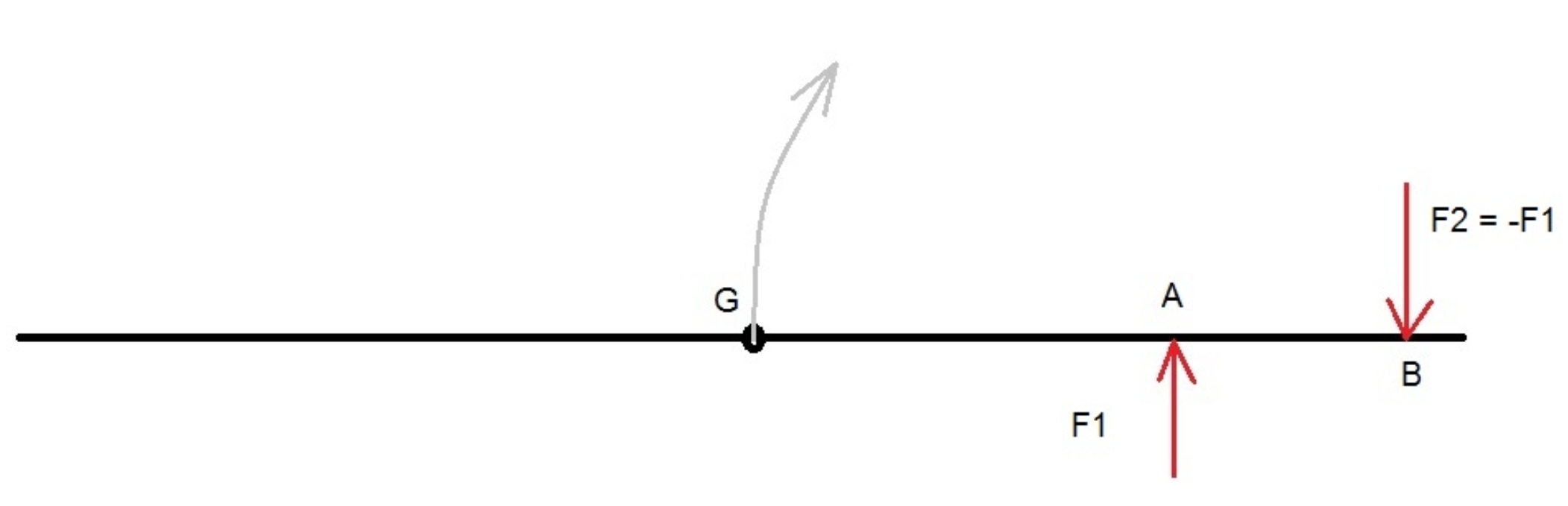Intuitively, I would say that the center of mass G would follow a circular trajectory, as pictured in gray in the schema. But this seems to contradict what I learnt when I was young, that the acceleration of the center of mass stems from the sum of the applied exterior forces (which is zero here). Some help to understand where I am wrong would be welcome.

Answer 1

I wish to see how will the rod move in a finite amount of time, so I assumed there are two opposite charges at the two positions $A$ and $B$ with a static uniform electric field along the vertical direction filling all space. This allows one to match the initial forces exactly with the question and also to solve for the finite-time behavior.

The kinetic energy is $T=1/2 m (\dot{x}_c+\dot{y}_c)+ 1/2 I \dot{\theta}^2$, where $I$ is the moment of inertia around the center of mass and $\theta$ is the angle the rod makes with $x$ axis. The potential energy is $V=-k y_A+k y_B=k(l_B-l_A) \sin\theta$, where $l_B$ is the distance of B to the center of mass, and similarly for $l_A$. Now the Lagrangian is $$L=T-V.$$ Using the Euler–Lagrange equation $\frac{d}{dt}\frac{\partial L}{\partial \dot{q_\alpha}}-\frac{\partial L}{\partial q_\alpha}=0$, one can get the equations for $x_c$, $y_c$, $\theta$: $$\frac{d ^2 x_c}{d t^2}=0$$ $$\frac{d ^2 y_c}{d t^2}=0$$ $$I \frac{d ^2 \theta}{d t^2}=-k(l_B-l_A) \cos\theta.$$ From the first two one can see that if the center of mass is initially at rest, it will remain so. From the third one can solve for $\theta(t)$, which can be done numerically. The bottom line is, the rod rotates with its center of mass being at rest the whole time.

I confess that even after deriving and solving these equations I still find it counter-intuitive.

Answer 2

Your intuition is wrong. The position of the center of mass doesn't change, and you've already explained why, i.e. for the second law of Dynamics.

The rods have an angular acceleration, given by the equation of motion for rotation of rigid bodies, whose component in the out-of-plane direction reads

$I_G \dot{\omega} = M_G^{ext} = -F d$,

having assumed counter-clockwise rotations as positive, $I_G$ the inertia around the out-of-plane axis, $\omega$ the component of the angular velocity in the same direction, $F$ the force magnitude and $d$ the distance between the action lines of the couple of forces.

Putting together the results from the equations of motion for translation and rotation, you can assert that body starts rotating around its center of mass, whose position in space is constant.