Shankar Monopole belonging to the class $-1$ of $\pi_3(\mathbb{R}P^3)$

Question

I am having a lot of trouble trying to understand how the classes of homotopy groups relate to point-defects in physics (and how they can be used/represent in general). This is a problem from Nakahara's textbook on geometry and topology in physics, but is NOT homework; I just want to learn homotopy theory in physics.

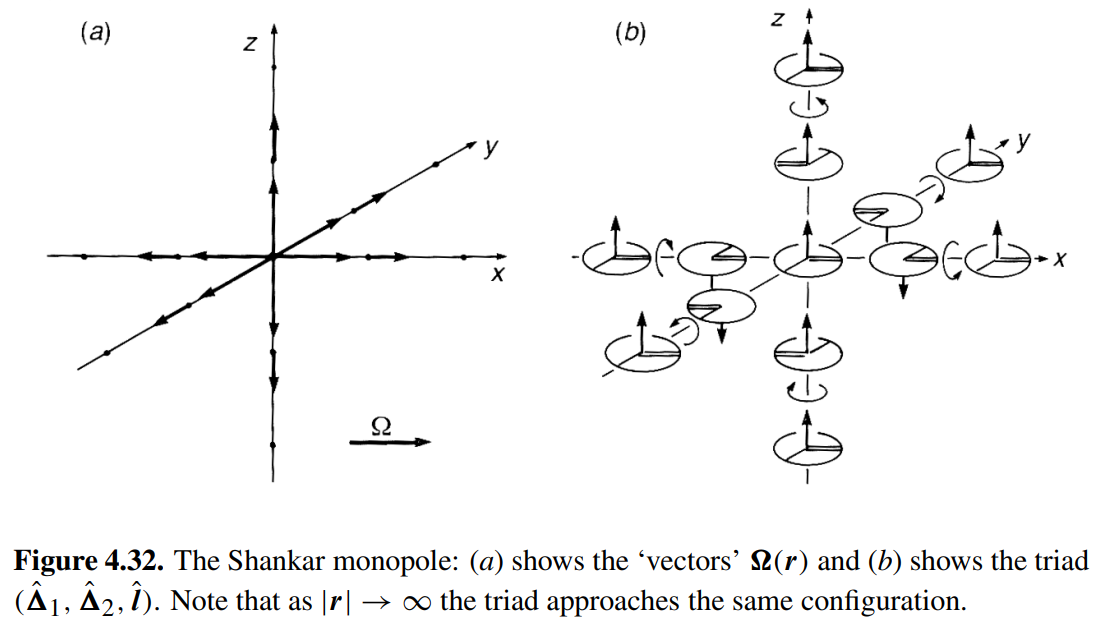
In Nakahara's text, he shows how the Shankar monopole comes about by stating, "[since we have] compactified $\mathbb{R}^3$ to $S^3$... the texture is classified by the third homotopy group of the real projective plane, $\pi_3(\mathbb{R}P^3)\cong\mathbb{Z}$." Now, I know that the real-projective plane is homeomorphic to a sphere, so finding the spheres homotopy group is how to proceed, namely in this case, $\pi_3(\mathbb{R}P^3)=\pi_3(S^3)=\pi_3(SO(3))$. Now, by the last equality, we can take a vector $\Omega(r) = \theta n$ where $n$ is an axis in $S^3$. Since $\Omega(r)$ traces out a disc, $D^3$, there are antipodal point that are the same rotation (this is how the relation between the $S^n$ and $\mathbb{R}P^n$ works (as far as I can understand). But, physically, Shankar decided to write the vector as $\Omega(r) = \frac{\mathbb{r}}{r}\cdot f(r)$ where $f(r)$ can take values of $2\pi$ for $r=0$ or $0$ for $r\rightarrow \infty$.

The trouble of understanding for me though comes from the next part in Nakahara's text where he says that, "as we scan the whole space, $\Omega(r)$ sweeps $SO(3)$ twice [as I described above], and this texture corresponds to class $1$ of $\pi_3(SO(3)\cong\mathbb{Z}$."

Now, how does this relate to class $1$ of $\pi_3(SO(3))$?? I understand that if $\pi_3(SO(3))\cong\mathbb{Z}$ then there are an infinite number of homotopy classes since $\mathbb{Z}$ is infinite. From a group perspective, this means there is one generator that creates the group of homotopy classes, but how this relates back to defining a vector $v(r)$ and then describing (and better yet drawing) a texture that relates to $-1$ of the same group, is where I am stuck.

I may be reading to fast over definitions and am not fully comprehending what they mean (this could completely be the case), or missing the mark entirely. Anything helps, thanks. (Also not sure if this should be put on the math stack exchange or not).

For clarity, the drawing/sketch I mentioned is the following image from page 167, figure 4.32 of Nakahara's text:

Answer 1

The answer might be a little late, but I'm also going through Nakahara at the moment:)

So I think, you might have missed a point of defining the negative elements of $\mathbb{Z}$; see Example 4.13 for this (in Nakahara 2004 Ed.). So the idea is that positive elements correspond to the mappings where the orientation of the surface does not change. Obviously, the minimum angle you need to map the zero point to by the texture having the same orientation is the point $2\pi$; any other angle multiple to this also won't change the orientation. Those multiples correspond to positive elements of $\mathbb{Z}$. Whereas the negative elements correspond to multiples of $\pi$ since they change the orientation. This answers your second question: the texture, that relates to $-1$, is the one with $f(0)=\pi$ instead of $2\pi$.

Hope that clarified some things (and I hope my understanding is correct; please correct me if I'm wrong).

EDIT:

See the answer in another OP's post here, as mentioned in the comment. As the OP has mentioned there, the answer to the second question is $\Omega = \Omega(x,-y,z)$.