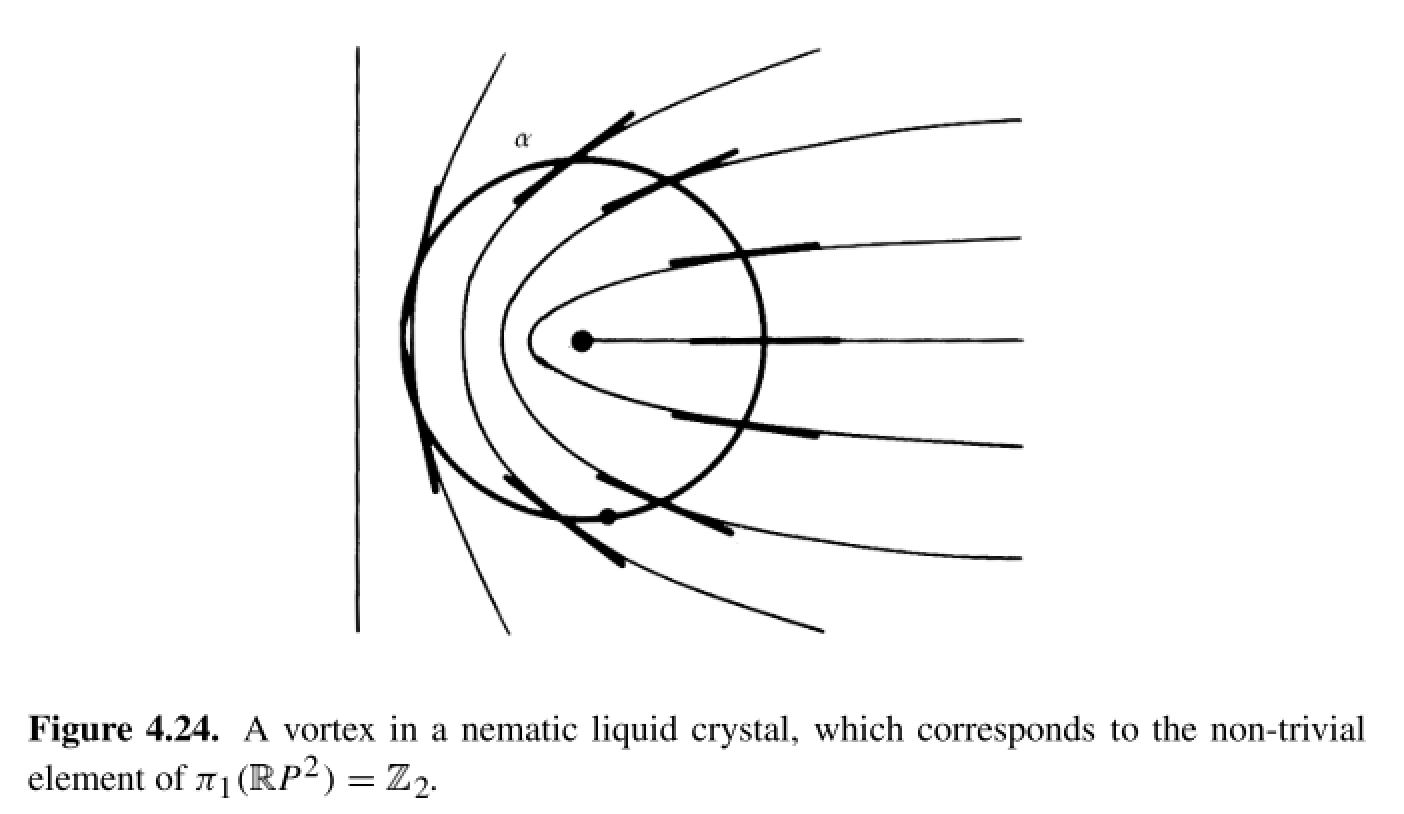
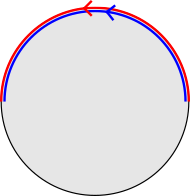
In Nakahara section 4.9, "Defects in nematic liquid crystals", it is discussed that the order parameter for a nematic should be the real projective plane $\mathbb{R}P^2$, which has fundamental group $\pi_1(\mathbb{R}P^2) \cong \mathbb{Z}_2 = \{0,1\}$. The real projective plane's fundamental group is generated by two homotopy classes, one being a trivial loop and the other a non-trivial loop which winds around the space. As discussed earlier in the text, the fact that the fundamental group is isomorphic to $\mathbb{Z}_2$ is equivalent to the fact that winding around the non-trivial loop twice creates a trivial loop, as demonstrated in this represetation, where antipodal points of the disk's boundary are identified:
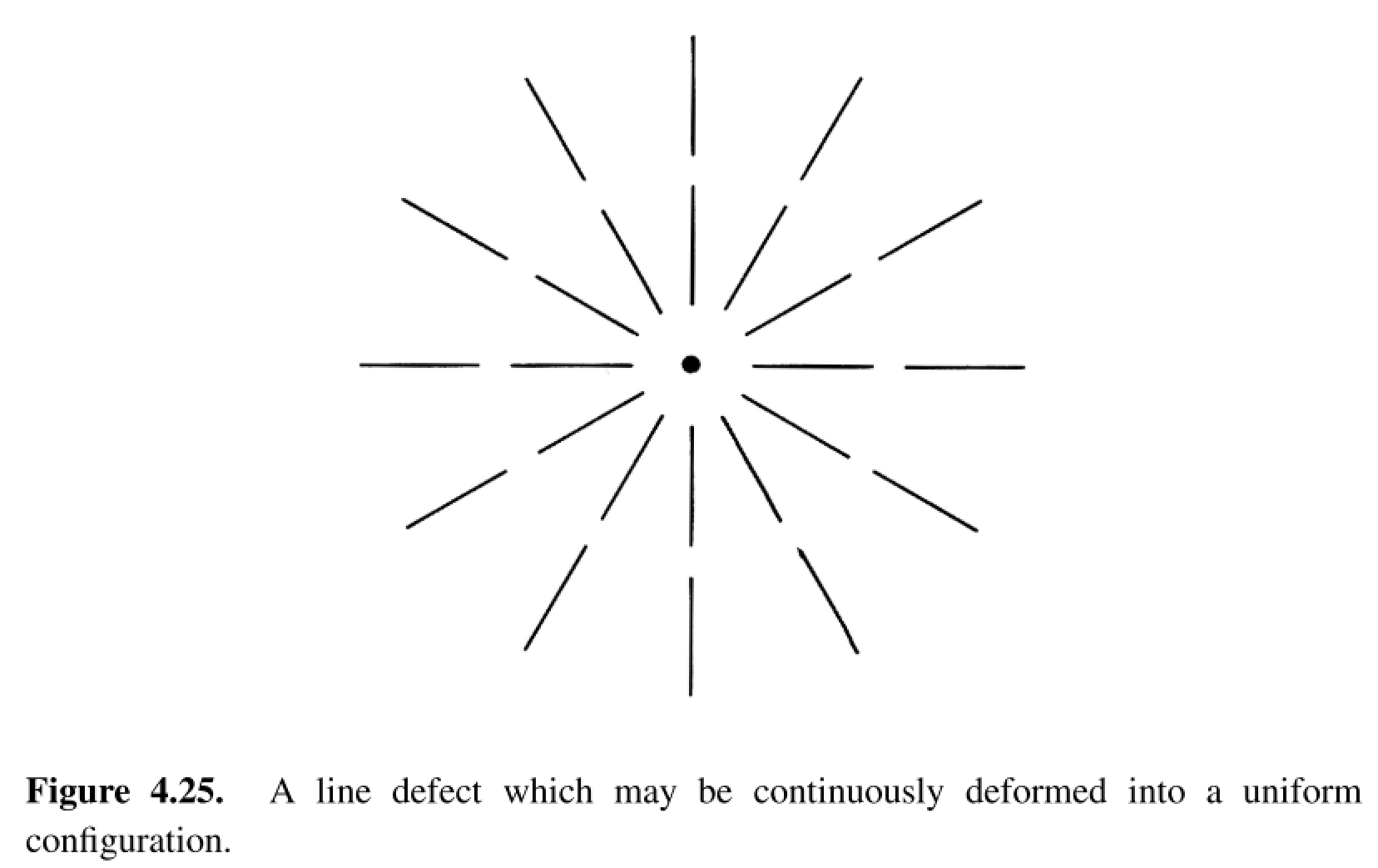
Correspondingly, it is then stated that there are two types of line defects in a nematic, one of which can be deformed to a uniform configuration and one which cannot. These are shown in the following two figures,
However, I am having trouble visualizing how the configuration in 4.25 can be deformed to a uniform configuration. I was trying to visualize it in the same way as in Figure 4.16: take a circle $S^1$ surrounding the line defect (like the curve $\alpha$ in 4.24) and think of how it maps into a curve on $\mathbb{R}P^2$ ala Figure 4.16, but have thus far I am still very confused as to how to visualize the deformation in 4.16 in terms of the configuration shown in 4.25.
Can anyone explain how the deformation should be done and how it relates to the deformation in 4.16? And for that matter, how the configuration in 4.24 maps into $\mathbb{R}P^2$?