Recently I have taken an interest in mathematical physics and as my background is mostly in math itself, I have quite a lot of catching up to do regarding my knowledge of physics. One phrase that I have heard (and read) time and time again is:
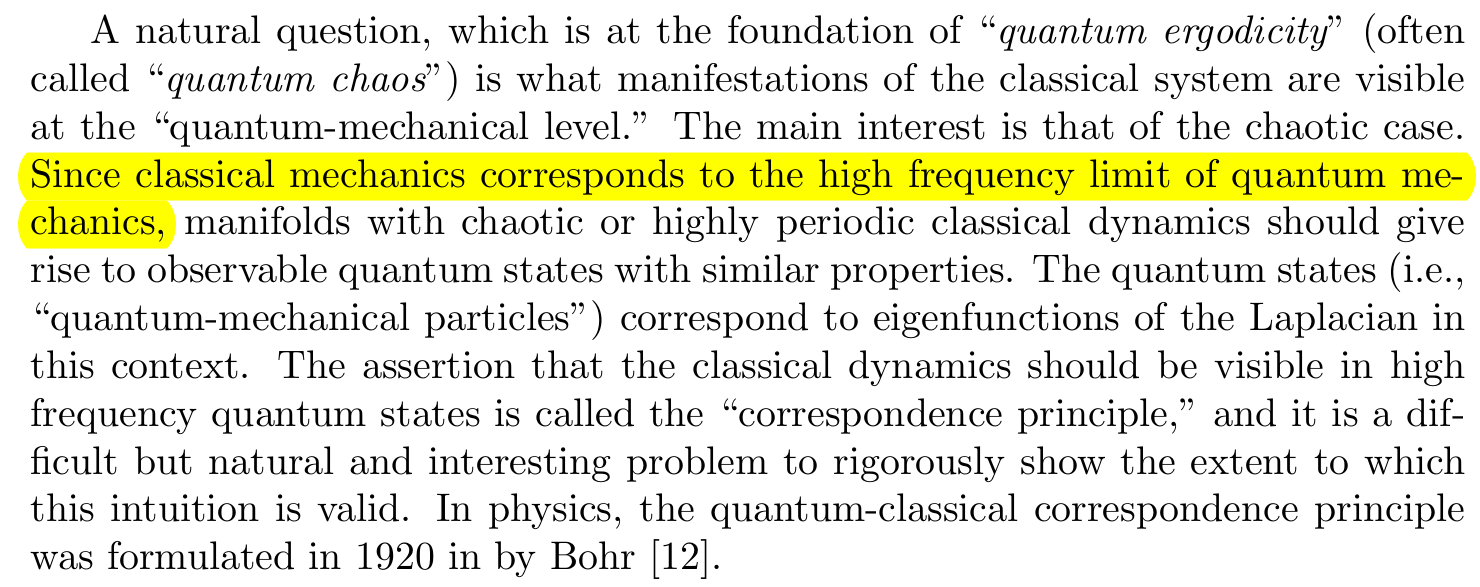
"Classical mechanics corresponds to the high frequency limit of quantum mechanics".
Unfortunately I have not managed to find a source which would discuss (and if necessary spoon-feed) the ins and outs of this phrase to me.
I understand that the phrase is discussing the limit as Planck's constant approaches zero, as $E = hf$ and my current knowledge regarding Planck's constant is that it represents discrepancy between the classical and quantum interpretations. My question is that are there some other analogies/"way" to think about the phrase in addition to
"Let us let the discrepancy approach zero. As frequency can be expressed as $f = \frac{E}{h}$, this is equal to letting $f\to \infty$".
Edit: The first reference that comes to my mind is from the chapter 6.2 of the Hangzhou Lectures on Eigenfunctions of the Laplacian by Christopher D. Sogge. I double checked other articles that I have been reading and it seems that no other has used that exact wording. Rather the high frequency limit has been of central interest in many articles, but the authors have not said (at least explicitly) what I claimed. One such article which discusses QUE and therefore the high freq. limits of the eigenfunctions of the Laplacian is Recent Progress On The Quantum Unique Ergodicity Conjecture" by Peter Sarnak.