Does positive pressure due to fast-moving particles create an attractive gravitational force in general relativity despite not having more true mass?

Question

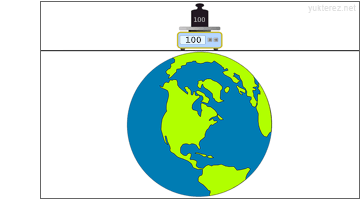
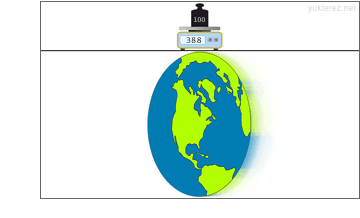
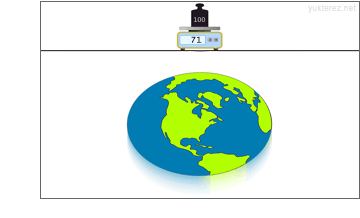
Matthew O'Dowd of PBS Spacetime explained, in his old video on dark energy and the cosmological constant, that even if a region of space has the same total energy and mass density as another, it will have a stronger, inward gravitational pull than the second region if its particles are moving more rapidly, due to the positive pressure of general relativity...

And yet Don Lincoln of Fermilab (among others) has repeatedly explained that particles moving at relativistic speeds do NOT have any additional true, rest mass/energy, only increased relativistic mass...

I can see where they might be agreeing with each other, in that a region of space with the same rest mass and energy density as another, but more rapidly moving particles, might ultimately exert more gravitational pull despite not having more 'true' energy density or mass, but...

I have heard (and read) multiple times that a massive particle moving at relativistic speeds does NOT have any increased gravitational effect....

Otherwise one of these cosmic rays (massive particles) moving at very nearly the speed of light would, according to Lorentz's gamma factor in special relativity, possess nearly infinite relativistic mass, and therefore exert as much gravitational pull as the Earth!

What am I missing here?

Do rapidly moving massive particles create extra 'positive pressure' in General Relativity, leading to a bit more inward-pulling gravity, just not as much as they would according to Lorentz and Einstein's original Special Relativity theory?

Answer 1

Kurt Hikes asked: "Do rapidly moving massive particles create extra 'positive pressure' in General Relativity, leading to a bit more inward-pulling gravity, just not as much as they would according to Lorentz and Einstein's original Special Relativity theory?"

If the earth in the following example would rest below the fixed scale, that would show 100kg for the weighted 100kg test mass:

but when the earth flies by with v=0.7c the scale with v=0 would show 388kg for the 100kg test mass at the closest approach:

If the earth's motion is not transverse, but radial, the fixed scale shows 140kg (γ), which is less than in the transverse case:

If we don't go by the closest approach, but by the same distance from center to center, we get even less, namely 71 kg (1/γ):

so it depends on the vector and how you measure the distances, but you can't just plug in the relativistic mass intead of M, you have to apply the full GR (click on the images to see how it is done in cartesian Schwarzschild coordinates, where the force required to keep a test particle with constant local velocity on a straight path from accelerating is calculated).

The whole thing is time symmetric, so you get the same result for an approaching or a receding mass at the same distance.

However, if you have a box full of particles moving in all directions like in the famous example of the box full of hot gas (which is heavier than a box of cold gas), the combined mass of the box in the rest frame of the box itself averages out to the sum of the particles' relativistic masses.