Let $U\subseteq \mathbb{R}^3$ be open and $f:U\to\mathbb{R}^3$ be a radially symmetric central force, that is, a force field such that $$f(p) = -g(r)u_r$$ where $r=|p|$ and $u_r$ is the unit vector pointing to $p$ for some integrable (this will be important later) function $g:\mathbb{R}_{\ge 0}\to \mathbb{R}$. I want to show that $f$ is a conservative force, and in particular that $$f=-\nabla (G\circ r)$$ where $G' = g$. Put differently, I wish to show that $$\int_Cf \cdot dr = (G\circ r) (t_0) - (G\circ r) (t_1)$$ for some bijective parametrization $r:[t_0,t_1]\to C$ of the smooth curve $C\subseteq U$.
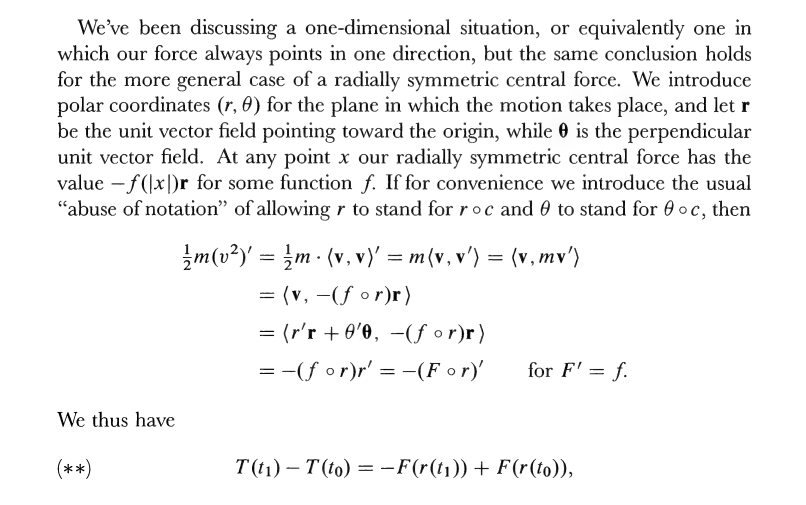
My interest in the above came after seeing a sort of argument for the above regarding planar curves. In case it is of any help, I include it here. It is from Spivak's Physics for Mathematicians: