Yes there is. Total energy is, in polar coordinates in the plane where the movement occurs:
$$E=\frac{1}{2}\,mv^2-G\frac{Mm}{r}=\frac{1}{2}\,m(\dot{r}^2+(r\dot{\theta})^2)-G\frac{Mm}{r}$$
A short rewrite using $C=\lVert\vec{L}\rVert/m$, the constant from Kepler's second law, yields:
$$E=\frac{1}{2}\,m\dot{r}^2+\underbrace{\frac{mC^2}{2r^2}-G\frac{Mm}{r}}_{=V_\text{eff}(r)}$$
The second and third terms form what's called the effective potential energy $V_\text{eff}$. Notice you necessarily have $V_\text{eff}(r)\leqslant E$, which can easily be interpreted on a graph:
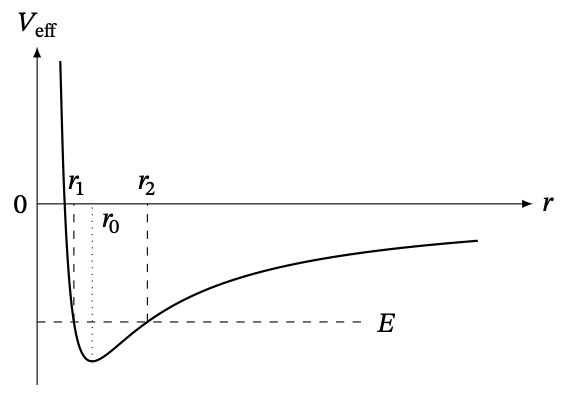
In this example, $E<0$. Since $V_\text{eff}$ must remain below $E$, the only part of the plot that is physically accessible is $r\in[r_1,r_2]$. In other words, $r$ cannot go to infinity, it's a bound state. This is the elliptic case.
On the other hand, if $E>0$, $r$ cannot go to zero, but it can go to infinity. The movement isn't bounded, it's the hyperbolic case.
The special case $E=0$ is the parabolic one ($r$ can go to infinity, but then kinetic energy is zero).
The special case where $E$ is exactly at the bottom of the potential well is the circular movement (only one allowed value for $r$, here $r_0$).
In other words, the sign of $E$ will tell you exactly in which case you are. $E$ can be rewritten as:
$$E=\frac{1}{2}\,mv_0^2-G\frac{Mm}{r_0}
\quad
\begin{cases}
>0 & \text{hyperbolic}\\
=0 & \text{parabolic}\\
<0 & \text{elliptic}
\end{cases}
$$
which should answer your question.

