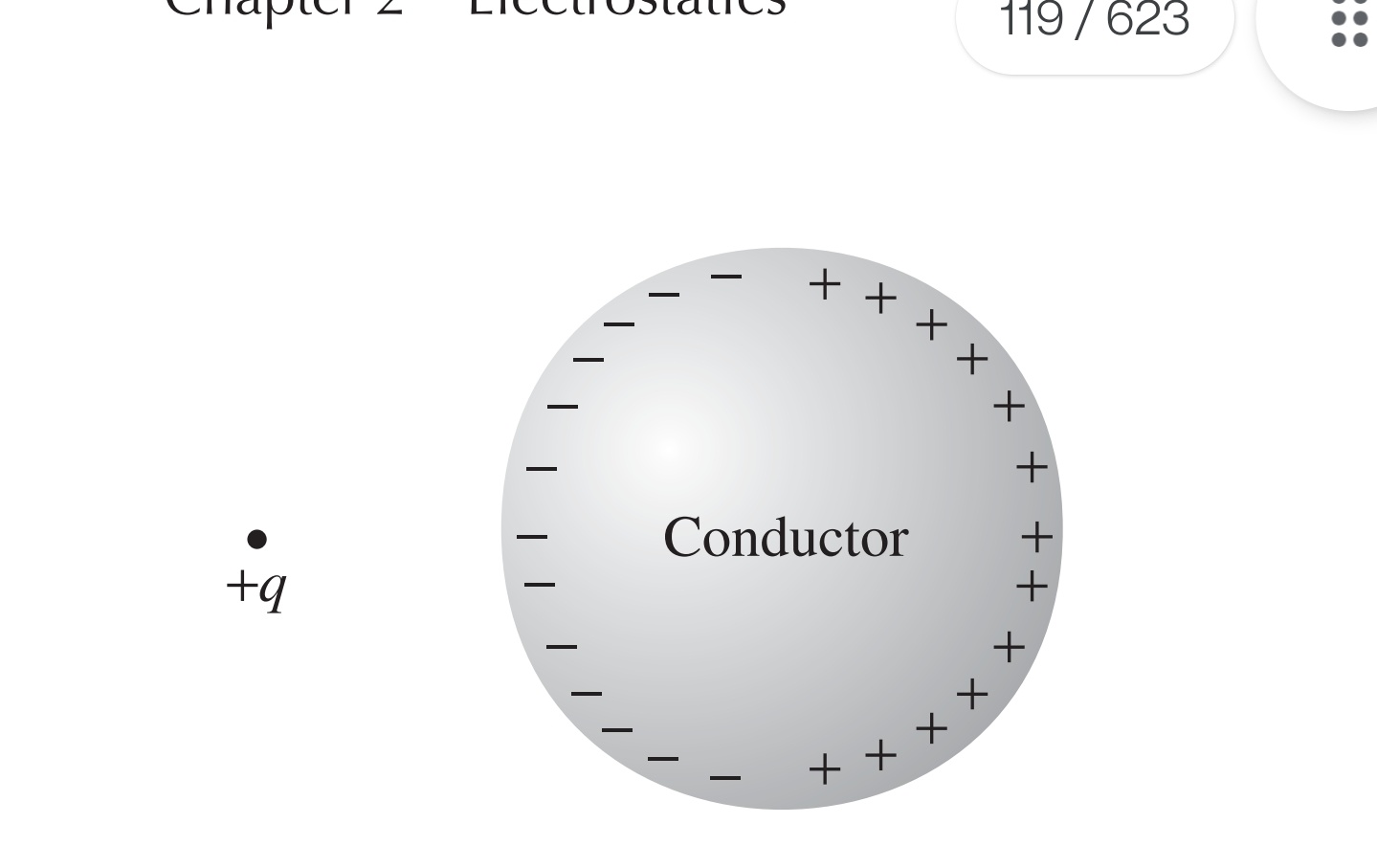
how can positive charges move to the far side? How can they move at all? I thought that positive metal ions don't move but only the electrons move (and since it's a conductor, I am assuming it consists of positively metal ions and free electrons).
The positively charged atomic nuclei do NOT move from one side of the sphere to the other. What happens is that some of the negatively charged electrons leave that side, leaving a net positive charge behind.
In electrolyte solutions and plasmas, positive ions do move, but in solids, the nuclei are essentially fixed in their position relative to their neighbors.
Regarding the same picture and charge distribution, I was also wondering if it is correct that a solid metal sphere can never have a volume charge, but only a surface charge, since the electric field must be zero inside a conductor always right?
That is essentially correct. A minor correction is that if there is a current in a conductor, there is an electric field $\vec{E}$ which is proportional to the current density $\vec{J}$ times the volume electrical resistivity of the conductor $\rho$.
$$\rho\vec{J}=\vec{E}$$
This is known as the "microscopic" version of Ohm's law.
Since the resistivity of most metals is quite small, the electric field will be small unless the current density is very large. The small electric field due a flow of current in a conductor is often ignored in practice.
However, even though there may be a small electric field within the body of a conductor in which there is an electric current, it is still true that charges do not accumulate in the interior of a conductor with uniform current density and uniform resistivity.
This can be shown by taking the divergence of the microscopic Ohm's law. If $\rho$ and $\vec{J}$ are uniform, then $\nabla \cdot \rho \vec{J} = 0$. Therefore $\nabla \cdot \vec{E} = 0$. Therefore by Gauss's Law, the charge density in that area of uniform current density $\vec{J}$ and uniform resistivity $\rho$ must also be $0$.
[Note that in this answer, I used $\rho$ as the symbol for resistivity. In the context of Gauss's Law, the symbol $\rho$ is often used for a different purpose, i.e. as the symbol for charge density. Please don't be confused by this.]