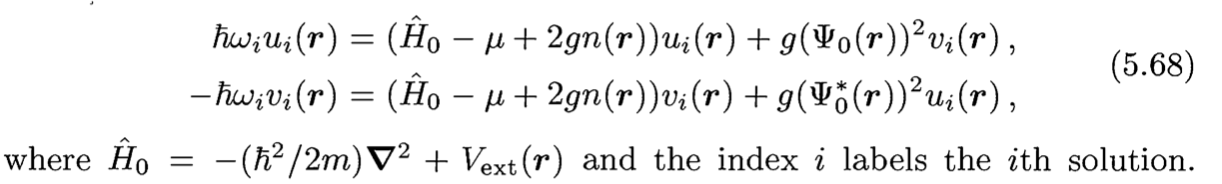0
$\begingroup$

Can someone please share how you would go on to solve the Bogoliubov-de Gennes equation for a Bose-Einstein condensate with a potential. In particular, how would you deal with the diagonalization when an operator (Laplacian in the Hamiltonian) is included? I would like to know the eigenvalues $\omega_i$ for the case of a trapped Bose gas (1D is ok, and for simplicty a step potential can be taken).

enter image description here

Improve this question
$\endgroup$
12
  • $\begingroup$ Or can I use a Fourier transform? But here there is NO translational invariance $\endgroup$
    – MrQ
    Commented Feb 14, 2022 at 11:20
  • $\begingroup$ As an extra, maybe one could provide a basic pseudocode for solving this. $\endgroup$
    – MrQ
    Commented Feb 22, 2022 at 19:39
  • $\begingroup$ Related question is stackoverflow.com/questions/71226231/… $\endgroup$
    – MrQ
    Commented Feb 22, 2022 at 20:22
  • $\begingroup$ If you are dealing with a solid, oftentimes there is discrete translational invariance of the potential (e.g., translations along the crystal lattice vectors). If you are dealing with "jellium" then there is translational invariance. $\endgroup$
    – hft
    Commented Feb 22, 2022 at 21:20
  • $\begingroup$ pseudocode for numerical solution of the Laplace equation or what? $\endgroup$
    – hft
    Commented Feb 22, 2022 at 21:21

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.