Physics of two orbiting masses connected with cable when the cable is cut

Question

The Problem:

Figure 1 - connected masses

In a 2D world without gravity or friction:

Lets say we have two masses, m1 and m2. They are both connected with some massless cable and the whole system is rotating about their center of mass cm with some angular velocity ω0. (See figure 1)

Q: When the cable is cut what is the resulting linear and angular velocity of each mass? (See figure 2)

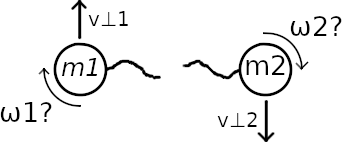Figure 2 - Cut cable for connected masses

My attempt:

Linear velocity is simple enough. I can use the equation for tangential velocity: v_⊥ = ω*r, where ω is the angular velocity of the system and r is the distance between the mass and center of mass of the system before separation.

v1_⊥ = ω0*r1  # Linear velocity of m1
v2_⊥ = ω0*r2  # Linear velocity of m2

Angular velocity is the one I'm struggling with. The angular momentum of the system must be conserved.

• L_before = L_after
• L_before = L_m1 + L_m2
• Using the formula for angular momentum L=I*ω
• I_m1&m2*ω0 = I_m1*ω1 + I_m2*ω2
• Using the formula for moment of inertia I=m*r^2
• (m₁*r₁² + m₂*r₂²)*ω0 = m₁*r₁²*ω1 + m₂*r₂²*ω2
• And this is where I get stuck.

The closest I could find to my problem is this post on Physics StackExchange: Will two bodies initially connected to and revolving around each other, start spinning when disconnected?

Answer 1

When the string is cut, both masses wil keep on rotating with angular velocity $\omega_0$. Before the cut they both rotate with this angular velocity around their central axis and there is no torque changing that. The angular momentum due to the rope is turned to linear momentum.

Answer 2

Just to add more details on Felicia's great answer:

The angular momentum of a particle can be given by the cross product of the particle's position with its momentum.

$L = r \times p$

Note that this means a rock flying in a straight line has angular momentum, and the amount of angular momentum it has depends on where you put the origin of your coordinate system. Angular momentum is conserved over time in all coordinate systems, but the total amount in two different systems might not match. One way of looking at this is to think of when people get shot in action movies. The bullet hits them in the shoulder, and their body twists around from the angular momentum. But if it hit them straight on in the middle of the chest this would not happen.

You have found out how to calculate the velocities of the two masses after the string is cut

$v_1$ = (0, $\omega_0 r_1$) and $v_2$ = (0, $-\omega_0 r_2$)

The positions of the two partciles are also quite easy. They are both travelling up or down the page (y-diretion) so the $x$ component of position is constant (at $-r_1$ and $+r_2$ for the two masses respectively). The angular momentum afterwards comes out as... exactly the same formula you wrote for the angular momentum beforehand (with the $\omega_0$ multiplying $r^2$).