6
$\begingroup$

Two extended bodies are connected with a string and revolve around each other (that is, around the center of mass of this system). No gravity, no external forces.

The string is cut, and they start to depart from each other. Will they spin around their own centers of mass?

For a (representative) particular case when one of the bodies is a dumbbell (two point masses connected by a light rigid rod),

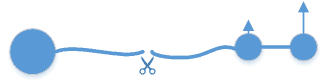
radial

the answer is trivial - each point mass will start its tangential movement with a different velocity, so the pair will be revolving.

On the other hand, for the following configuration,

co-radial

there seems to be no rotation.

Is there a general approach?

Improve this question
$\endgroup$
1
  • 2
    $\begingroup$ In the second case there will be rotation about the center of mass of the two balls. That’s because the tangent velocity at the location of ball 1 is not parallel to the tangent velocity at location of ball 2. $\endgroup$
    – Al Brown
    Commented Aug 22, 2021 at 16:52

2 Answers 2

Reset to default
7
$\begingroup$

The bodies are spinning before the string is cut, once per revolution. Since neither the string nor the cutting of the string apply torque to the bodies, there will be no change of rotational speed for the bodies, so they will keep spinning.

To think of this another way: at the moment the string is cut, the part of the body connected to the string is moving slower than the part of the body directly opposite due to the circular motion. Cutting the string does not change the speed of any part of the body, so the difference in velocity in different parts of the body will result in an overall rotation.

In your second illustration, the two balls have different velocities, as shown with the arrows below:

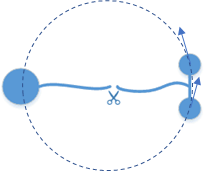
Illustration of a spinning system with one ball to the left and two balls in line with the instantaneous motion. Arrows show these two balls have different velocities due to their separation.

These different velocities would result in an overall rotation in the two-ball system after the string is cut.

Improve this answer
$\endgroup$
10
  • $\begingroup$ What if the string was initially connected to some principal axis so that the objects did not rotate before the string was cut? $\endgroup$
    – Mauricio
    Commented Aug 22, 2021 at 15:05
  • $\begingroup$ @Mauricio Are you thinking of something like the string being connected to the axis of a toy top? $\endgroup$
    – Mark H
    Commented Aug 22, 2021 at 15:13
  • $\begingroup$ Yeah, I guess the result in that case is different and should be acknowledged. $\endgroup$
    – Mauricio
    Commented Aug 22, 2021 at 15:14
  • 1
    $\begingroup$ @Mauricio Not necessarily. If the string is only connected at one point, then the top will rotate until its center of mass is aligned with the string. Even if the connection point is at the center of mass, then the top will rotate if the axis isn't a 1D line. I could imagine a multipoint connection rigging with slip rings that would allow for spinning without the top rotating, but that goes rather far beyond the generic situation described by the OP. In any case, cutting the string still doesn't change the rotational motion of the bodies about their own centers of mass. $\endgroup$
    – Mark H
    Commented Aug 22, 2021 at 15:26
  • $\begingroup$ Indeed, the bodies are spinning before the string is cut. But I am not sure that this spinning is not because they are connected by the string. In your terms, this is exactly my question - does the string apply torque to the bodies? $\endgroup$
    – uk-ny
    Commented Aug 22, 2021 at 15:30
2
$\begingroup$

Take a ball of radius $r$ connected to a thin center-post by string of length $R$. The post accelerates up to and stays at a high final rotation speed $\Omega$. Obviously the ball revolves at $\Omega$. Now imagine case 2 where a thin, light ball-bearing is connected to the string and the ball rotates within it.

Lookin down along the axis of rotation, draw an arrow ⬆️ on the top of the ball. In case 2 it always points the same way, and in case 1 it rotates once per revolution.

At the same angular velocity $\Omega$ of the system: $$ v_1=v_2 =v= \Omega (R+r) $$ $$ \omega_1 = \Omega ~,~ \omega2 = 0$$

By conservation of angular and linear momentum, after the cut: $$v_{1,f}=v= \Omega (R+r) ~,~ \omega_{1,f}= \Omega$$

$$v_{2,f}=v= \Omega (R+r) ~,~ \omega_{2,f}= 0$$

Yes it spins as it travels away no matter what shape it is.

In your lower pic the tangent velocities are not parallel for the two balls.

By the way, the kinetic energy in case 1 is higher: $$E_1= \frac{1}{2} m v^2 + \frac{1}{2} I_{ball} \omega^2$$ where $I_{ball}$ is the moment of inertia of a sphere around around its axis. The second term is gone for case 2: $$ E_2= \frac{1}{2} m v^2= \frac{1}{2} I_{mass} \Omega^2$$ where $I_{mass}$ is the moment of inertia of a point-mass in revolution, $m(R+r)^2$ in this case. We also just derived the parallel axis theorem where $E_1= \frac{1}{2} I_{tot} \Omega^2$ and $I_{tot}=I_{mass}+I_{ball}$.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.