2
$\begingroup$

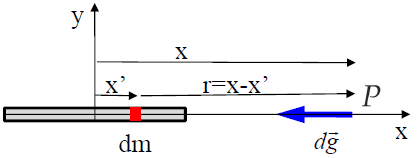
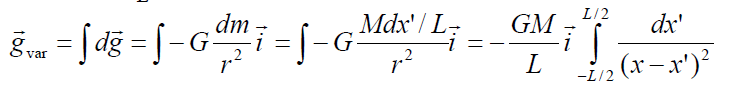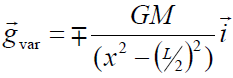enter image description here

I just calculated the gravitational field on the $x$-axis created by a thin rod of mass $M$ and length $L$ by applying the formula:

enter image description here

I got this:

enter image description here

However there are some issues that I don't get. If I plug $ x = \pm L/2$ on the equation, gravitational field goes to infinity. Why? What's its physical meaning?

Another thing that bothers me is the fact that when I plug $x=0$ in the equation the result is $\vec{g}(x=0)= \frac{4GM}{L^2}\vec{i}$, but shouldn't it be 0 since $x=0$ is in the middle of the rod and by simmetry it looks like the net field right there is 0?

Improve this question
$\endgroup$
2
  • $\begingroup$ I believe your problems are occurring since you are considering an infinitely thin rod, aka 1d, of you did an area or volume integral for the center it would be 0 $\endgroup$
    – jensen paull
    Commented Jan 24, 2022 at 23:38
  • $\begingroup$ Double check the expression you have for $\vec g$. $\endgroup$
    – joseph h
    Commented Jan 25, 2022 at 1:27

1 Answer 1

Reset to default
1
$\begingroup$

careful -- you wrote down your $d\vec g$ as though it only points in the $+\hat i$ direction when that's not the case for $x<0$. you need to split up your integral. one interval goes from $-L/2$ to $0$ and the other from $0$ to $L/2$.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ That worked! I first tried to compute the integral your way but I got that it diverged to infinity... Later I realized that I had to take Cauchy principal value in order to get the correct result and I got 0, so everything went right in the end :). Thanks a lot!! $\endgroup$
    – Javieer Picazo
    Commented Jan 25, 2022 at 15:34

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.