0
$\begingroup$

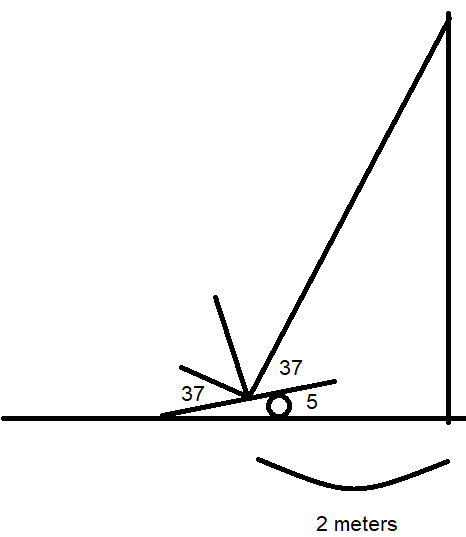
Here's the question directly from the book:

Sunlight enters a room at an angle of 32$\circ$ above the horizontal and reflects from a small mirror lying flat on the floor. The reflected light forms a spot on a wall that is 2.0 m behind the mirror, as shown in Figure 16.31. If you place a pencil under the edge of the mirror nearer the wall, tilting it upward by 5.0$\circ$, how much higher on the wall ($\Delta$y) will the spot of light appear?

Figure from book

I first found the initial height without putting the pencil under the mirror $$h_1=(2m)(\tan(32\circ))$$

Then, I found the second height after the pencil has been put under the mirror not to scale

We find that the angle of incidence is $32+5=37$

not to scale too

Then I added $37+5=42$ to get the angle opposite the wall and took and did some trig $$h_2=(2m)\tan(42\circ)$$

Finally, $$\Delta y=h_2-h_1$$

After checking my answer on Quizlet I saw a different answer due to calculating $h_2$ differently. However I noticed in the diagram that part of the mirror after rotation is underground meaning whoever wrote the answer interpreted the question differently. Just to check, is my solution or Quizlet's solution correct or is the question ambiguous?

Improve this question
$\endgroup$
2
  • $\begingroup$ I just checked the book's appendix. The answer is 0.55 which agrees with my answer. I'm not sure if I should delete this. I spent my time writing it instead of preparing for my test. $\endgroup$
    – LostPositron
    Commented Jan 2, 2022 at 22:46
  • $\begingroup$ Correct. $\Delta h = h_2 - h_1 = h\ tan \theta_2 - h\ tan \theta_1 = 2\times [tan(42) - tan(32)] = 0.55\ m$ $\endgroup$
    – joseph h
    Commented Jan 2, 2022 at 23:11

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Browse other questions tagged or ask your own question.