1
$\begingroup$

...

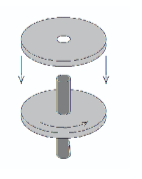
A disk, with rotational inertia $J$, rotates with angular velocity $w_0$ around a vertical axis, which has negligible rotational inertia. A second disk, initially at rest and with $2J$ inertia, is suddenly coupled to the system, as illustrated in the figure. The friction between the two discs causes them to rotate at the same speed. What is the final angular velocity of this system?

Comment: Ok, I know that I can use the conservation of angular momentum in this case:

$J w_0=3Jw$

$w=\frac{w_0}{3}$

But why using conservation of energy we get a different answer?

$\frac{1}{2}J w_0^2=\frac{1}{2}(3J)w^2$

$w=\frac{w_0}{\sqrt{3}}$

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

Energy is not conserved here due to friction. Same case when you have inelastic collision. There energy is not conserved, but linear momentum is.

Here angular momentum is conserved because no external moment is acting on this system. When you place your disk on top of the rotating one, they act on each other due to friction with forces of opposite orientation, hence changing individual angular momenta of disks, while total angular momentum stays constant.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ The idea of inelastic collision was a good point. Like a bus colliding with a car in rest results in a bus+car moving together, we have here a rotating disk (bus) colliding with another disk in rest (car) which results in a two-disk rotating together. In these two cases, conservation of energy is not true because of inelastic collision. Thank you, now it is clear for me! $\endgroup$
    – Guilherme Correa Teixeira
    Commented Nov 24, 2021 at 13:42

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.