We know that like charges repel each other. But my professor claimed that two electrons can attract each other as well. What he said was that due to screening an electron travelling at some speed won't repel another electron, but that they will, in some cases, attract each other due to weak phonon exchange. What does that mean? What is phonon exchange? Do two electrons really attract each other?
3 Answers
Just as two oppositely pointed bar magnets (magnetic dipoles) attract, two electrons embedded within cancelling positive atomic charges can form transient electrical dipoles that attract. The catch is that to create such dipoles, there must be some form of "wobble" in the way that negative and positive charges are around the electrons.
A Cooper pair is a quantum version of just such a wobble-initiated dipole attraction. The effect that creates the wobbles is a quantized version of ordinary sound, also called a phonon. The synchronized way in which the charges wobble at each end of the Cooper pair is described in quantum terms as a "phonon exchange." Like two bar magnets rotating end over end in exact synchronization, this coordinated wobble dance enables the dipoles formed by the electrons to stay attached indefinitely.
Balancing all of this is the fact that these are conduction electrons, meaning they are part of "gas" of electrons that moves relatively freely within the neutralizing positive charge of the metal. If any pair of electrons gets too close to each other within such a gas, the neutralizing charges of the atomic lattice cannot move with them, and you start to develop a local negative charge in that region.
That charge buildup keeps the electrons in Cooper pairs from getting too close to each other, despite the attraction created by their phonon-coordinated charge wobble. This repulsion effect keeps the electrons in a Cooper pair surprisingly far from each, in the order of hundreds of atomic diameters apart. It is also why Cooper pairs are so delicate, since a mere dipole bond over those distances doesn't have much oomph. It can easily be broken by random vibrations (heat) that upsets the wobble dance.
The real magic in all of this, however, stems from a quantum effect that has no classical physics analogy of which I am aware. The two electrons -- or to be more precise, the two quasiparticle dipole pairs they form -- have half-integer spins, which makes them into mutually repulsive fermions. Once bonded, these half spins cancel each other, giving the overall Cooper pair spin of zero. Whole-integer spin makes Cooper pairs into a composite bosons, and such bosons can join together in a fully coordinated fashion that is quite impossible for fermions. All of the most remarkable properties of superconductors stem from that last seemingly minor point in the overall formation of Cooper pairs.
Addendum: @Danu pointed out this excellent illustration by Dr Ronald Griessen at Vrije Universiteit. It shows how rapid motion electron motion through an atomic lattice creates a lagging charge displacement, and thus a charge dipole. (Dr Griessen has some excellent online physics notes at that site, BTW.)
Picture the box on the right as a (not to scale!) snapshot of a phonon-synchronized oscillation going on continuously between two electron sites, and you will have a pretty solid classical-analogy lock on how exchanging phonons (or in classical analogy, vibrating synchronously) can bind two electrons together:
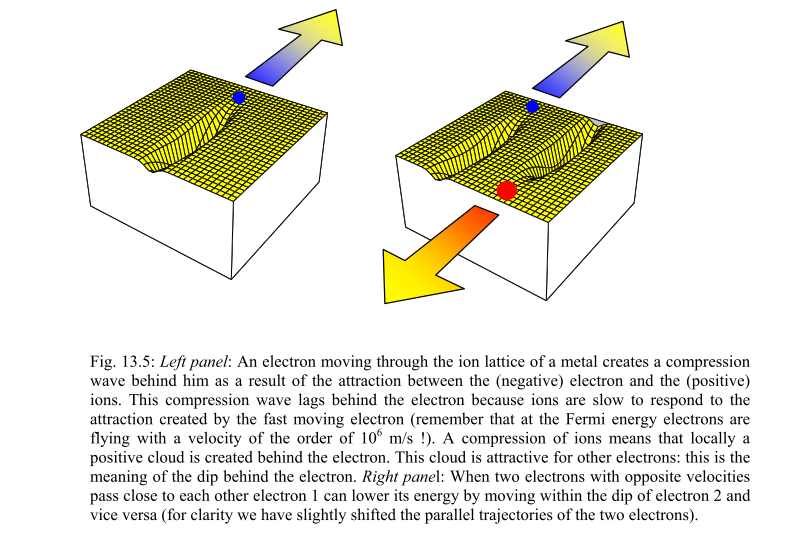
-
1$\begingroup$ This description gives somewhat a false message that the 2 electrons are coupled together in the real space, but from my understanding it is the wavefunction of the 2 electrons that is coupled rather than the physical coupling of the 2 electrons. The 2 electrons of the Cooper pair can be spatially separated as well. $\endgroup$ Commented Nov 26, 2017 at 4:24
-
$\begingroup$ @Draco_1125 Well, he does mention that it's a quantum effect to which there is no real classical analog. I agree that the semi-classical picture is somewhat wrong. Humans seem to have a psychological need to "locate things" in space and time, which simply doesn't apply in quantum mechanics. That is probably where all these attempts at illustrations come from. Are they physically correct? No. $\endgroup$ Commented Aug 13 at 19:47
From the Wiki article Cooper pair:
In condensed matter physics, a Cooper pair or BCS pair is two electrons (or other fermions) that are bound together at low temperatures in a certain manner first described in 1956 by American physicist Leon Cooper. Cooper showed that an arbitrarily small attraction between electrons in a metal can cause a paired state of electrons to have a lower energy than the Fermi energy, which implies that the pair is bound. In conventional superconductors, this attraction is due to the electron–phonon interaction. The Cooper pair state is responsible for superconductivity, as described in the BCS theory developed by John Bardeen, Leon Cooper, and John Schrieffer for which they shared the 1972 Nobel Prize.
Although Cooper pairing is a quantum effect, the reason for the pairing can be seen from a simplified classical explanation. An electron in a metal normally behaves as a free particle. The electron is repelled from other electrons due to their negative charge, but it also attracts the positive ions that make up the rigid lattice of the metal. This attraction distorts the ion lattice, moving the ions slightly toward the electron, increasing the positive charge density of the lattice in the vicinity. This positive charge can attract other electrons. At long distances this attraction between electrons due to the displaced ions can overcome the electrons' repulsion due to their negative charge, and cause them to pair up. The rigorous quantum mechanical explanation shows that the effect is due to electron–phonon interactions.
-
$\begingroup$ I have been reading this article since few hours and something is making me confused. I dont know how can an electron distort the lattice site even though the positive ion has much greater charge than a single electron? $\endgroup$ Commented Jun 12, 2013 at 1:48
-
1$\begingroup$ @A4KASH, I think it may be the case that you're failing to distinguish between the case of "not distorting the lattice at all" and the case of "minutely distorting the lattice". The next sentence after the quote of the article I provide is: The energy of the pairing interaction is quite weak, of the order of 10−3eV, and thermal energy can easily break the pairs. So only at low temperatures are a significant number of the electrons in a metal in Cooper pairs. $\endgroup$ Commented Jun 12, 2013 at 1:53
-
2$\begingroup$ @AlfredCentauri The most important point of the Alfred Centauri's answer is obviously that the second paragraph of the Wikipedia description is a simplified classical explanation of the Cooper pairing. The Cooper pairing is better explained (to my mind) as an instability of the Fermi sea caused by electron-phonon coupling, as the first paragraph of his quotation tells you. You need a quantum mechanical treatment to understand the Cooper pairing. By the way, neither a phonon nor an electron is a classical object :-) $\endgroup$ Commented Jun 12, 2013 at 18:01
-
2$\begingroup$ I feel like this answer is a little too much of a case of link-only... Could you perhaps expand a little? $\endgroup$– DanuCommented Oct 25, 2014 at 18:55
-
$\begingroup$ @Danu, I understand your concern, however, my interests are elsewhere for now. I will consider expanding a little in time. $\endgroup$ Commented Oct 26, 2014 at 2:27
We consider a piece of metal and zoom into its structure. If we abstract all the mathematics of the quantum mechanics of this situation (which of course takes away the majority of the truth behind this phenomenon but allows a more intuitive understanding), we can consider the system as a simplified arrangement of a typical metallic lattice consisting of layers of vibrating metal ions that have "donated" their Valence (highest energy) electrons to a sea or pool of delocalised electrons. Passing a current through a metal at room temperature leads to collisions between the moving electrons and the vibrating ions, resulting in kinetic energy transfer to these ions, increasing the speed and amplitude of their vibration - this manifests itself as an increase in temperature of the metal and therefore energy loss by heating. This concept is known as power dissipation due to resistance, from useful electrical energy to thermal energy.
Now, let us cool this piece of metal close to absolute zero. Temperature is a measure of the average kinetic energy of the particles in an object - at absolute zero there is no kinetic energy whatsoever, and hence we can say that the metal ions in the cooled metallic lattice will show negligible vibration. An electron moving through this structure in between the layers of the metal ions will attract the positively charged ions via the standard Coulomb interaction (very simply the attraction between positive and negative charges). Since the positive metal ions move closer to this electron and increase their density at its location, they cause a local positive charge buildup, albeit distorted, to form around the region where the electron is situated in the metal - this is known as a phonon.
Another electron, which may be 100-1000 atoms away from the first electron, will feel an attraction towards this local positive charge disturbance, causing it to weakly bind to the first electron. The two electrons have now attracted each other through the phonon interaction which occurs via the lattice of metal ions!
In fact, the electrons have now formed something called a Cooper pair, which can very easily be broken by even so much as a small vibration - hence the requirement for extremely low temperatures for most superconducting materials. Many such Cooper pairs can form throughout the supercooled metal.
Note that an electron is a fermion - it has a half-integer spin (either 1/2 or -1/2) and so must obey the Pauli Exclusion Principle, which says that no two fermions can obey the same quantum state (that is why we cannot walk through walls!). An interesting quantum phenomenon that occurs with Cooper pairs is that the spins of the electrons in a Cooper pair combine to give an integer spin (0 or 1), characteristic of bosons. The Cooper pair therefore acts as one combined entity in a bound state, as a boson, and so does not obey the Pauli Exclusion Principle. As a result of this, the various Cooper pairs are able to "clump" together in the lowest energy ground state and form one entity called a Bose-Einstein condensate by occupying the same quantum state.
This condensate consisting of the Cooper pairs can now flow through the metal indefinitely without any resistance - superconductivity explained! Even if there is one collision, the network of Cooper pairs can recombine; alternatively we can say that since the Cooper pairs are all in their ground state, no more energy can be taken away from them, giving zero resistance in the metal. This is BCS theory simplified. However, it is not yet able to explain the mechanisms behind how room-temperature superconductors work.
Hope this was helpful.
-
$\begingroup$ (+1) Suppose there are 2 pairs of cooper electrons. Each pair is moving along in one direction with nice correlated momentum. Could you elaborate why would these 2 independent pairs clump together to form a BE-condensate instead of simply traveling each their own independent path through the lattice? $\endgroup$– JamesCommented Aug 14 at 3:27
-
$\begingroup$ Thank you for your question. Firstly, we should note that the picture I have painted in the comment above is a simplification to aid intuition - the true nature of Cooper pairs is described by the abstract mathematics of quantum mechanics; I have used the word "clump" as a visual tool to symbolise the pairs occupying the same quantum state. $\endgroup$ Commented Aug 14 at 17:44
-
$\begingroup$ Essentially, after the Cooper pairs form, they all collapse into the lowest energy state - the ground state - which is possible with entities resembling bosons (but NOT fermions - this is why the two electrons occupying an orbital in an atom MUST have opposite spins). A condensate is simply a collection of particles occupying the same quantum state - I am not familiar with the description of a condensate in terms of the maths, but I think the main concept to remember here is that since the Cooper pairs all occupy the same quantum state, they behave as one entity - the BE Condensate. $\endgroup$ Commented Aug 14 at 17:47
-
1$\begingroup$ Not sure if that answers your question, but hope this helps! If you find out anything more about your question in your own research, feel free to comment it. $\endgroup$ Commented Aug 14 at 17:48
