2
$\begingroup$

Recently a found a paper on the thermoelectric effect:

https://williamsgj.people.cofc.edu/Thermoelectric%20Effect.pdf

When I started with Chapter 5 "Irreversible Thermodynamics" I struggle totally with concepts of "Entropy Flow". My question is general and not related to thermoelectricity yet. I just want to understand the concepts the author uses to derive some features.

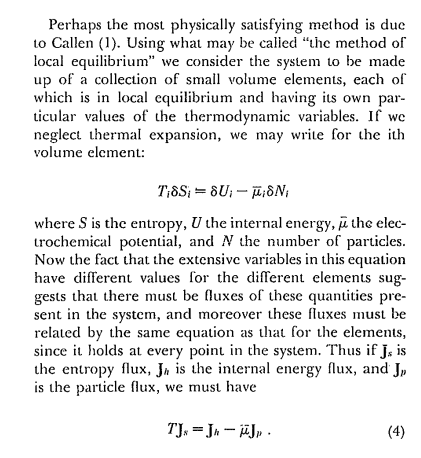
On page 6 a system is split into a set of subsystems, each in local equilibrium. Then the author writes

$$T_i \delta S_i = \delta U_i - \mu_i \delta N_i$$

I would understand this as a relation describing equilibrium states which are close together.

Next the author presents an equation where I cannot follow in detail:

$$T J_s = J_h - \mu J_p$$

Where $J_s$, $J_h$ and $J_p$ denotes the entropy flux, internal energy flux and particle flux.

Unfortunately I cannot follow what is meant with "Entropy-Flux". Entropy is a state variable of a system or a sub-system, how can there be a "flux"?

A few lines after that, he defines heat flux and relates it to entropy flux:

$$J_Q = T J_s$$

Of course I know, that for reversible processes $dQ_{rev}= T dS$, but this would imply that the processes behind are all reversible. In general $$\delta Q \le T dS$$

so why can we relate heat flux directly to entropy flux. There are processes possible, where dQ=0 but the entropy of a system increases anyway - for instance expansion of a gas into a bigger volume after opening a valve.

enter image description here enter image description here

When the chapter is obviously about "irreversible thermodynamics", why do we assume reversible processes from the beginning? Isn't this a discrepancy?

Unfortunately I'm completely lost with those concepts. I'm aware that the topic is complex - if there is not an easy answer possible, where can I read more about it? My textbook of thermodynamics doesn't cover such things and just deals with equilibrium thermodynamics.

Improve this question
$\endgroup$
5
  • 1
    $\begingroup$ mass is a state variable, I do not think you have a problem with its flux. $\endgroup$
    – hyportnex
    Commented Nov 16, 2021 at 21:40
  • $\begingroup$ Very nice question. I also had exactly.the same remark about the inequality becoming an equality even though this is irrevetsible thermodynamics and seems contradixtory. As far as i rmember i found the solution in the boook by de groot and mazur in the first pages of Non equilibrium thermodynamics. $\endgroup$
    – untreated_paramediensis_karnik
    Commented Nov 17, 2021 at 12:24
  • $\begingroup$ As far as i remember it had to do with the definition of S, which had to be the total entropy rather than a subset of it, but i forgot the details. If you find tbe solution pkease write an answer, id uovote it, ublike the current naswer whixh doesnt deal with your question. $\endgroup$
    – untreated_paramediensis_karnik
    Commented Nov 17, 2021 at 12:33
  • $\begingroup$ Related: physics.stackexchange.com/q/672039/226902 physics.stackexchange.com/q/526818/226902 $\endgroup$
    – Quillo
    Commented Mar 14, 2023 at 0:38
  • $\begingroup$ Regarding entropy flow: it is heat exchanged through a boundary physics.stackexchange.com/a/253312/226902 $\endgroup$
    – Quillo
    Commented Mar 14, 2023 at 0:53

1 Answer 1

Reset to default
2
$\begingroup$

There are two ways that the entropy of a closed system can change:

  1. By heat flow across the boundary between the system and its surroundings at the boundary temperature $T_B$. This part of the entropy change is given by $\int{\frac{dQ}{T_B}}$, where the integral is carried out along the process path from initial state to final state. This contribution to the entropy change is present in both reversible and irreversible processes; moreover, in a reversible process, there are no temperature variations within the system, so that $T_B=T$ along the process path where T is the (uniform) system temperature.

  2. Entropy generation within the system as a result of irreversibility within the the system. This part of the entropy change, denoted $\sigma$ is always positive, unless the process is reversible, in which case it is equal to zero.

So, based on this, the total entropy change of a closed system experiencing an irreversible process is $$\Delta S=\int{\frac{dQ}{T_B}}+\sigma$$And, for a reversible process, $$\Delta S=\int{\frac{dQ}{T}}$$

Improve this answer
$\endgroup$
13
  • 1
    $\begingroup$ You say: $\Delta S=\int{\frac{dQ}{T_B}}+\sigma$. But then I would get by integrating over flux quantities $\int J_S= \int J_Q+ \frac{d \sigma}{dt}$. In the paper I see $\int J_S= \int J_Q$. Does it mean only reversible processes are considered there? I cannot find this explicitly stated. Do you see my "Problem" now? $\endgroup$
    – MichaelW
    Commented Nov 17, 2021 at 12:24
  • 2
    $\begingroup$ Answer to the first comment: T is not necessarily spatially uniform in an irreversible process, so, for such a case, value of T within the system ( at what location) do you mean? $\endgroup$
    – Chet Miller
    Commented Nov 17, 2021 at 12:31
  • 2
    $\begingroup$ A finite rate chemical reaction carried out adiabatically and at constant volume is an example of an irreversible process with uniform temperature. Of course, in this case, there is no heat flow at the boundary, but the entropy of the system increases. $\endgroup$
    – Chet Miller
    Commented Nov 17, 2021 at 15:47
  • 1
    $\begingroup$ The post you submitted before my most recent post is correct. A reversible process is one consisting of a continuous sequence of thermodynamic equilibrium states. $\endgroup$
    – Chet Miller
    Commented Nov 17, 2021 at 15:52
  • 1
    $\begingroup$ It's basically the definition of a reversible process. $\endgroup$
    – Chet Miller
    Commented Nov 17, 2021 at 17:27

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.