I need help with a concrete problem that needs solving through good application of physics principles. Here's the problem:
I would like to build a filtering system for my bestway pool, the pool has only one inlet and one outlet for filtering water, diameter of each 32 mm.
I also have a system that can heat the water by passing the filtered water through some 1/2 inch pipes (that are buried under concrete, so I can't replace them) to a heat exchanger to the heat pump.
And I have a 10 M3/h filtering pump. My problem is that the pipes to the heating are too small for the 10M3/h pump, this causes a pressure build up in the sand filter, which is plastic, breaking it rather quickly.
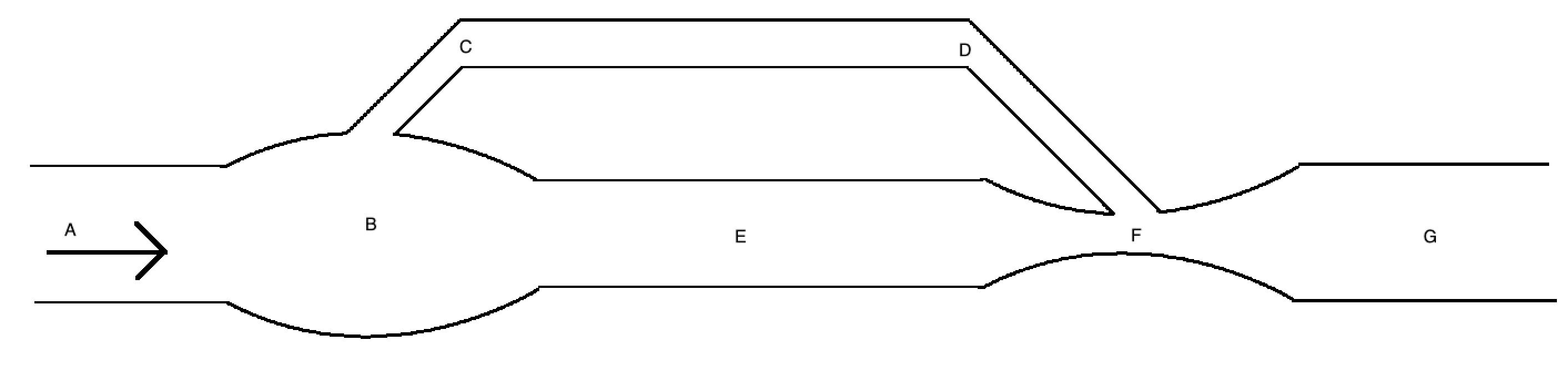
I would like to modify the system, so that the 10m3/h pump is only used for actual filtering, while the heating is done using a smaller pump (2.5 m3/h). The pool still has only one intake and one outtake for water, so I will need at some point to have some sort of T split. to split the two flows, and rejoin them after heating/filtering. like shown here: 
Because the two pumps are very different in their hydraulic characteristics, I'm worried that I won't be able to run both pumps at the same time, i.e. the powerful one will make the small one useless.
I'm looking for a solution to this:
Venturi said that if I have a pipe, and reduce it's diameter in the shrinkage I'll get lower pressure. I imagine the opposite will be true too: if I enlarge the pipe, I'll get a higher pressure.
So if
- pressure at A = pressure at B
- pipe diameter at A = diameter at B
- main pipe diameter at C < diameter at A
- main pipe diameter at D > pipe diameter at B
then
- pressure at C < pressure at A
- pressure at D > pressure at B
- pressure at C < pressure at D
I could then join these two pieces together as in the figure:
because there's a higher pressure at B (pipe C) than there's at F (pipe D), then there must be a flow in the lateral pipe C-D. even when there's no pump in this pipe, but only one located at E.
now, I'm not too sure there can be real flow without a pump on C-D but at least I hope I might be able to have a smaller pump on C-D running the water heating system.
I'm planning to 3d print the parts for this, so I have the chance to calibrate the sizes of all the pipes.
If this principle is sound, how would I calculate the diameters of the pipes and how would I shape the T junctions to make it work?
I got this idea from this: https://www.thingiverse.com/thing:4920000