How does the weight to break a bar depend on the dimensions?

Question

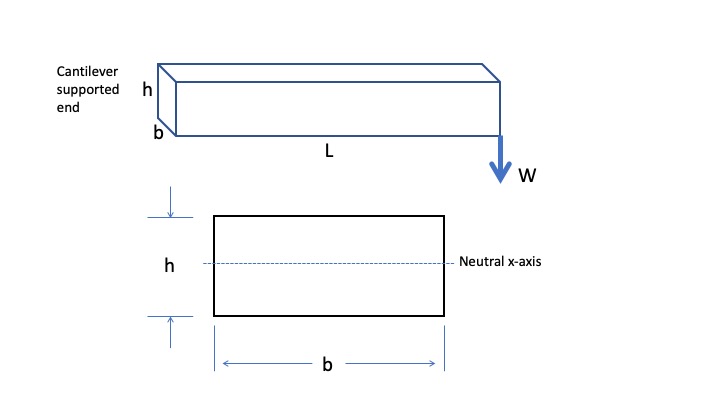
Imagine a horizontal bar of glass, say, 10 cm long, 1 cm large and 1 mm thick. At one end it is maintained stably, e.g. by a screw clamp, and at the other extremity, some increasing weight is added (e.g. by suspending an appropriate container which is filled with water) until the threshold where the bar breaks. I am wondering how this threshold depends as a function of the bar's length, width and thickness. Obviously, it should be proportional to the width (think of placing several identical bars with weights besides each other, it should not make a difference whether they are glued together or not), but what about thickness (naively, I would expect that to be linear again) and about length (inversely proportional? or a different negative power?)

[And by curiosity again: at which point will it break when increasing the weight slowly?]

If we use some other (not elastic) material instead of glass, e.g. brass, hard plastic, dry wood or ice, would the formula for the threshold be the same, with just some different constant depending on the material (and of course of the gravity)?

A similar question, maybe easier to conceive but more tricky to calculate (?): what if the bar is fixed at both ends, and the weight is applied exactly in the middle?

Answer 1

In general, for a given shape, material strength is proportional to cross-sectional area, and material stresses are proportional to the cross-product of force and the length of the lever, so we would expect your rod to support a max weight

$F_{max} \propto \frac{A}{Lsin\theta}$

Where $\theta$ is the angle between the weight vector and the length vector between where the force is applied and where the rod is supported. Note for a given shape. If we started experimenting, we would find that a hollow rod could support almost as much weight as a solid rod with twice the cross-sectional area, while a rod that was flattened out into a thin film would break with barely any weight applied, and so on.

A more detailed approximation would want to consider at least bending moment, buckling critical load, fracture strength, and tensile strength, and is the sort of thing for which you'll want a textbook, not an answer in a forum post. As you would expect, this is a subject of great importance to mechanical and structural engineers.

Answer 2

I am wondering how this threshold depends as a function of the bar's length, width and thickness.

It appears you are describing a cantilever supported beam made of glass. For such a support the maximum bending and shear stress, and thus the stresses associated with the threshold of bending or shearing failure, occurs at the fixed (supported) end of the beam.

Assuming a solid rectangular bar of height $h$, base $b$, and length $L$ with a weight $W$ hanging vertically from the free end (see figures below), maximum normal stress $\sigma_{x}$ due to bending and maximum shear stress due to the vertical load $W$, are:

$$\sigma_{x}= \pm\frac{6WL}{bh^2}$$

$$\tau_{xy}=\frac{3W}{2bh}$$

Derivations can be found in any mechanics of materials textbook.

Hope this helps.