I'm trying to clarify in my mind the use of Airy functions as matching functions across a turning point in the WKB approach.
Quoting from Section 3.1 of Migdal and Krainov, Approximation methods in Quantum mechanics, 1969, W.A. Benjamin, Inc.:
... such a procedure is legitimate only if we know that the quasiclassical approximation is valid at distances from the turning point small in comparison with the distance at which the potential departs appreciably from linearity.
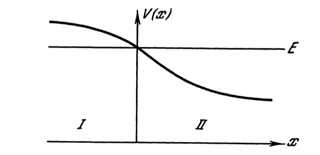
Migdal and Krainov then proceed to provide an estimate of the boundaries of this region. Near the turning point (conveniently taken at $x=0$) it is assumed that $V'(0)<0$ as per figure. Working with $m=\hbar=1$, they introduce $k(x)=\alpha \sqrt{x}$ with $\alpha =\sqrt{-2V'(0)}$, and estimate
$\alpha\sim \sqrt{\vert V'(0)\vert}\sim \sqrt{V(0)/\ell}\sim > k_0/\sqrt{\ell}$,
where
$\ell$ is the distance over which the potential $V(x)$ changes appreciably,
and $k_0=\sqrt{2E}$.
(I believe there is a factor of $\sqrt{2}$ missing in writing $\alpha$, and it's put back in the definition of $k_0$ but that's probably a typo and doesn't affect the argument since this is an order of magnitude estimate).
They then show that
... we can always choose a value $x_1$ such that $$ \frac{\ell}{(k_0\ell)^{3/2}}\ll x_1 \ll \ell\, .$$ At distances of order $x_1$, the quasiclassical approximation is already valid, which at the same time we can still take the potential to be linear.
My question is on the estimate $k_0\sim \sqrt{2E}$: in other words, it appears the authors have taken $V'(0)=\Delta V/\ell \approx E/\ell$. Simple dimensional analysis shows that $V'(0)$ must be the ratio of some energy divided by $\ell$, and the only energy scale is $E$, but it seems taking $\Delta V(0)\approx E$ is quite drastic, so that $k_0<\sqrt{2E}$, and that therefore $1/(k_0)^{3/2} >1/(2E)^{3/2}$.
Is there a better way to justify this estimate of $k_0$, or an alternate estimate of $k_0$? Alternatively, is there a better bound on the size of the region where WKB is applicable and the potential does not depart too much from the linear approximation? For instance if $\Delta V=10$ while $E=100$ (in arbitrary units), then using $k_0\sim \sqrt{2E}$ instead of the "better" $k'_0\sim\sqrt{2\Delta V}$ leads an error of size $(k_0/k_0')^{3/2}\approx 30 $, which considerably changes the bound on the left of $x_1$.