Equivalence of Maxwell and electric field operator in the Coulomb gauge (minimal and polar coupling)

Question

In the field theory literature, we find the interaction Hamiltonian coupling a point particle with charge $e$ and mass $m$ to the electromagnetic field to be

$$ \hat{H}_\text{int}(t) = - \frac{e}{m} \hat{p}^i(t) \hat{A}_i(t,\boldsymbol{x}) \tag{1}, $$ wherein $\hat{p}^i(t)$ is the particle's $i$th momentum operator, and $\hat{A}_i(t,\boldsymbol{x})$ is the Maxwell field's $i$th field component in the Coulomb (transverse) gauge.

However, outside of field theory, e.g., in atomic physics, we find the interaction Hamiltonian to be

$$ \hat{H}_\text{int}(t) = - e \hat{x}^i \hat{E}_i(t,\boldsymbol{x}) \tag{2}, $$ wherein $\hat{x}^i$ is the particle's $i$th position operator, and $\hat{E}_i(t,\boldsymbol{x})$ is the $i$th component of the electric field operator. Often the first two factors are defined as the electric dipole operator, $\hat{d}^i=-e\hat{p}^i$.

In Quantum Optics by Mandel & Wolf, the interaction Hamiltonian in eq. (1) is referred to as minimal coupling while the interaction Hamiltonian in eq. (2) is referred to as polar coupling. Furthermore, Mandel & Wolf claim that there exists a unitary transformation

$$ \hat{U} = \exp\left\{ -ie\hat{x}^i\hat{A}(t,\boldsymbol{x}) \right\} \tag{3} $$

connecting these two couplings. In Photons and Atoms - Introduction to Quantumelectrodynamics by Cohen-Tannoudji (p. 253), we find some more insights between the difference of the minimal and polar couplings. In particular, he claims that both descriptions are equivalent and correspond to different gauge conditions.

Questions

1. Is my understanding correct that the polar and minimal formalisms are equivalent?
2. Are there any limitations to this equivalence, for instance, second-order coupling $\hat{A}(t,\boldsymbol{x})^2$?
3. It appears to me that the "fundamental" fields are different in the minimal and polar description, Maxwell field, $\hat{A}$, vs electric field, $\hat{E}$. However, we usually say that the Maxwell field (or vector potential) is unphysical as we can change it by a gauge transformation. How do we resolve this contradiction?

Update 1 (11.11.21)

Addressing the feedback from ACuriousMind

1. What about the Cohen-Tannoudji argument you already seem to have found is insufficient for you?

Good point, the explanation requires quite some context, which might qualify for a separate question (I was hoping to avoid that).

In photodetection theory, we derive a relation between the photocurrent of a photodetector, e.g., a phototube or photodiode, from the atom-light interaction. Most authors, e.g., Cohen-Tannoudji et. al. in Atom—Photon Interactions: Basic Process and Applications, use the polar coupling interaction, eq. (2). Mandel & Wolf use the minimal coupling interaction, eq. (1).

In both cases, they find that the mean photocurrent is proportional to an intensity operator. However, depending on the field, the intensity operator is either given by the Maxwell or electric field, i.e.,

$$ \begin{align} \hat{I}_A(t) &= \hat{A}^{(-)}(t) \hat{A}^{(+)}(t) & \hat{I}_E(t) &= \hat{E}^{(-)}(t) \hat{E}^{(+)}(t) . \tag{4} \end{align} $$

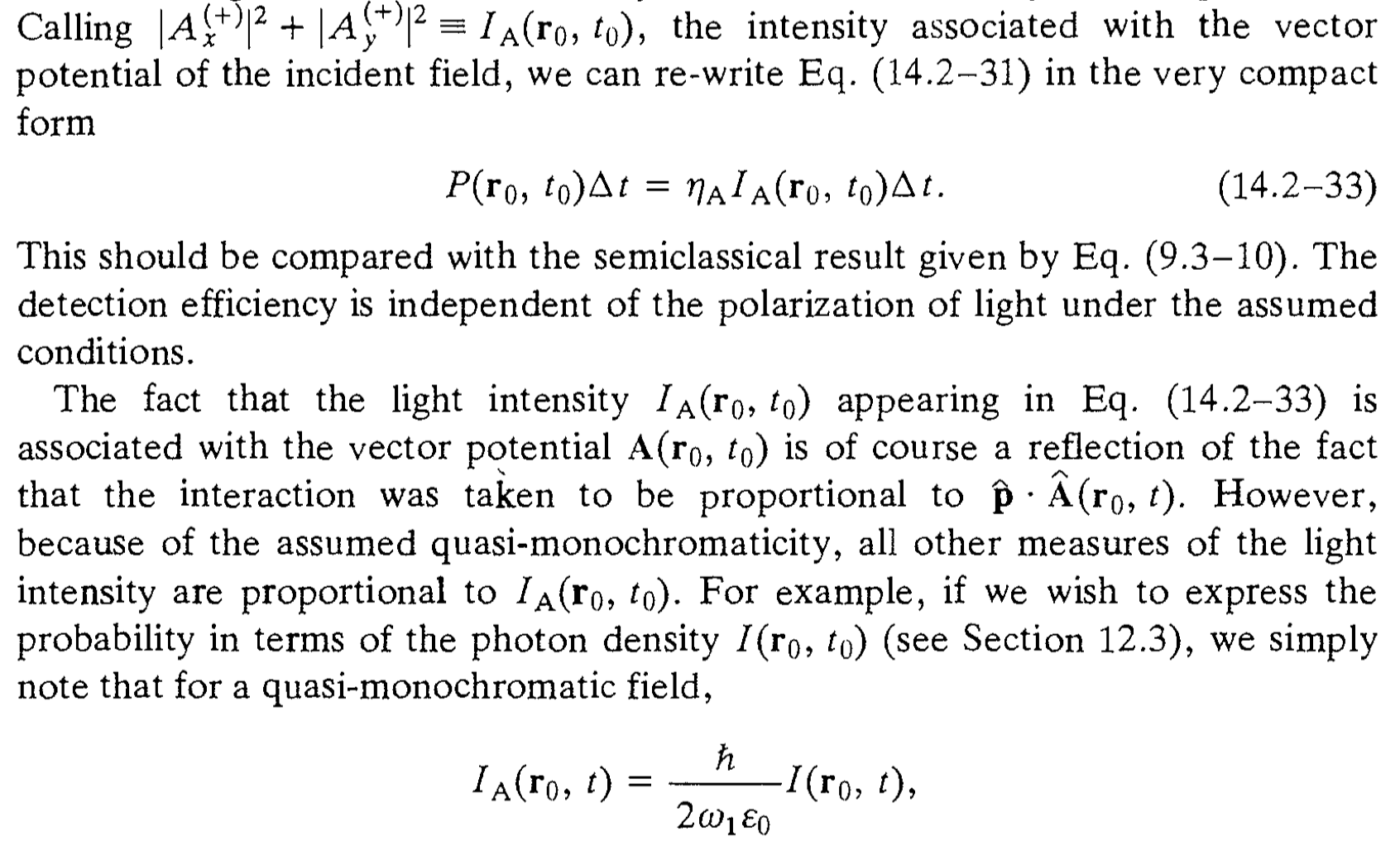
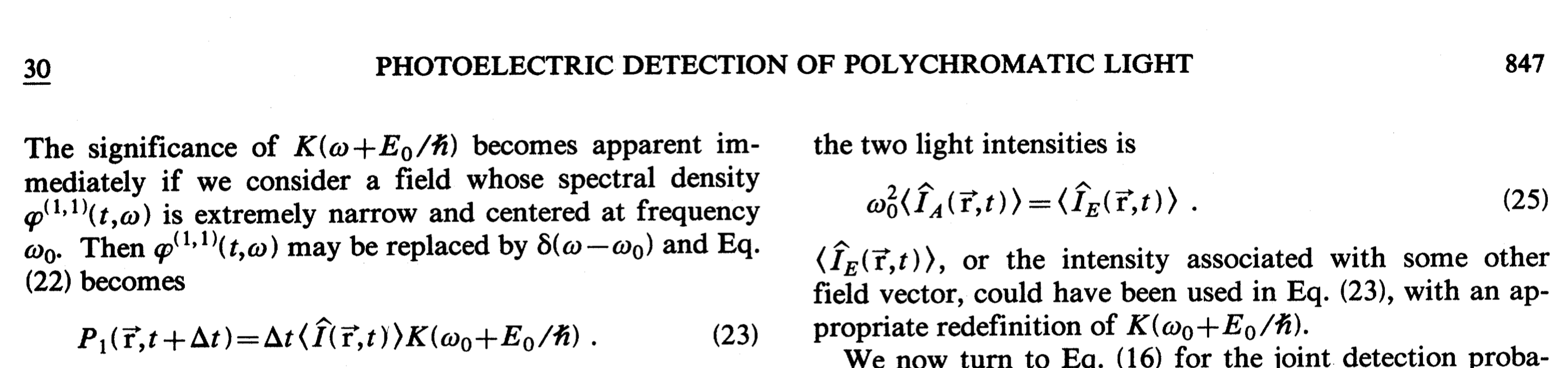
Mandel & Wolf address this issue by stating that they are related by $$ \omega_0^2 \hat{I}_A = \hat{I}_E $$ the same in the narrow-bandwidth approximation where the center-of-mass frequency is $\omega_0$.

If we do not invoke the narrow-bandwidth approximation, we get different predictions depending on the interaction we started. It should be possible to determine if the photocurrent depends on the field frequency to rule out the polar coupling!

2. Where would such a coupling come from? The overall action still has to be gauge-invariant!

Such a coupling usually appears when we couple the momentum of a charged particle with the field, i.e.,

$$ (p-eA)^2 = p^2 -pA -Ap +e^2A^2 .\tag{5} $$

3. Neither of these fields is "fundamental" in this case because you're not treating them as dynamical but as background fields here. But the "unphysical" vector potential appears as the central dynamical/fundamental field e.g. in the classical Lagrangian formulation of ED. What exactly is the "contradiction" you see here?

See my remark to your first question.

Update 2 (13.11.21)

Apparently, I was not clear about the photocurrent operator. Let me try to clarify.

In Quantum optics by Mandel & Wolf (p. 701), we have

which I believe misses a power of two over the $\omega_1$.

In Photodetection of polychromatic light by Kimble & Mandel, we find eq. (4):

These are not definitions but predictions. Depending on if we start with the minimal or polar coupling, eq. (1) or eq. (2), the photocurrent is either frequency-dependent or not!

In the quasi-monochromatic limit (narrow-bandwidth approximation) they are related by a multiplicative factor.

In principle, it should be possible to measure the (mean) photocurrent and see if there is any quadratic frequency dependence.

In practice, the frequency response of a photodiode (Google spectral sensitivity or responsitivity) might be much stronger compared to the frequency dependence between the photocurrent operators.

Answer 1

To show the two formulations are equivalent, note that in Coulomb gauge, $A^0=0$ so $E_i = \partial_i A_0 - \partial_t A_i = - \dot{A}_i$.

Then using $p_i = m \dot{x}_i$, and defining $H_A$ to be the Hamilonian written in terms of $A$ and $H_E$ the Hamilontian written in terms of $E$, we have \begin{eqnarray} H_A &=& -\frac{e}{m}p^i A_i \\ &=& -e \dot{x}^i A_i \\ &=& \frac{d}{dt}\left(e x^i A_i\right) + e x^i \dot{A}_i \\ &=& \frac{d}{dt}\left(e x^i A_i\right) - e x^i E_i \\ &=& H_E + \frac{dG}{dt} \end{eqnarray} where $G\equiv e x^i A_i$. In other words, the two Hamiltonians differ by a total time derivative of the "q" variables in the $x,A$ description. This total time derivative can be removed with a canonical transformation using standard techniques.

Adding a higher order non-minimal coupling like one that is $\sim A^2$ is of course possible, and you could formulate any gauge invariant coupling in terms of either $A$ or $E$ and $B$. The details will depend on the non-minimal coupling.

In terms of whether $E$ or $A$ is more fundamental, I would say that this is a matter of perspective and not really a physics question. On the one hand, $E$ is more directly related to observables in a lab and is gauge invariant. On the other hand, it is much easier to quantize electromagnetism in terms of the vector potential (I actually have never seen a quantization directly in terms of $E$ and $B$, although that doesn't mean it doesn't exist) and it is easier to understand subtle quantum effects like the Aaronhov-Bohm effect and magnetic monopoles in terms of $A$. In general, in field theory, there is an enormous degeneracy of equivalent descriptions related by field redefinitions, so one learns not to place too much weight in any one description in terms of fields and instead focus on observable quantities like the $S$-matrix that are invariant under such choices.

Note that if $\partial_t A_i = -E_i$, then you could equally say $E_i = -\int dt A_i$. Since $E_i$ is an observable, doesn't that make $A_i$ an observable? How can that be if $A_i$ is a gauge field? Well, actually, $A_i$ is an observable if you fix a gauge completely. The catch is that any expression you write with $A_i$ in that gauge will be wrong in any other gauge. So usually it's better to replace $A_i$ with $E_i$ in any expression you want to represent an observable, then you have a gauge invariant expression that's true in any gauge. This is similar to the trick in GR where you pick a coordinate system, derive a relationship that holds between quantities in that coordinate system, and then replace those quantities with tensors that are equal to the original quantities in that coordinate system, but which have nice transformation properties under changes of coordinates. The resulting tensor expression has to be true (since it reduces to the coordinate-dependent result you derived) but holds in any coordinate system (since, relations between tensors are coordinate-independent by design).