Misconception with Fourier Transforms in a Gaussian Light source?

Question

So for a Michaelson Interferometer , we know that the (complex) interferogram ($I=I(\Delta)$, $\Delta$=path difference between two mirrors) is related to the intensity profile of the light source $(I_S=I_S(\tilde{\nu}),\tilde{\nu}=1/\lambda$ denotes the wave number) by a Fourier Transform $$I(\Delta)=\frac{1}{2}\int I_S(\tilde{\nu})exp(-i2\pi\tilde{\nu}\Delta)d\tilde{\nu}, 0<\tilde{\nu}=\frac{1}{\lambda }<\infty \tag{1}$$ The detected intensity is then $Re(I(\Delta))$. [][1

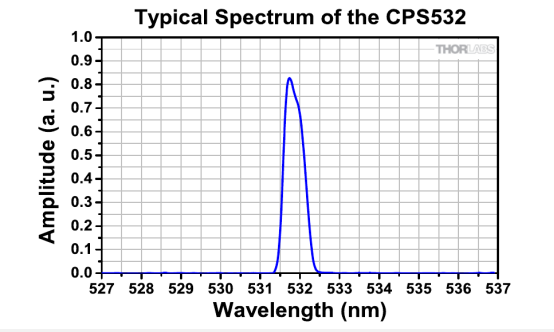
I am asked to predict the gaussian profile width ($\delta \Delta$) of the interferogram for the green laser below:

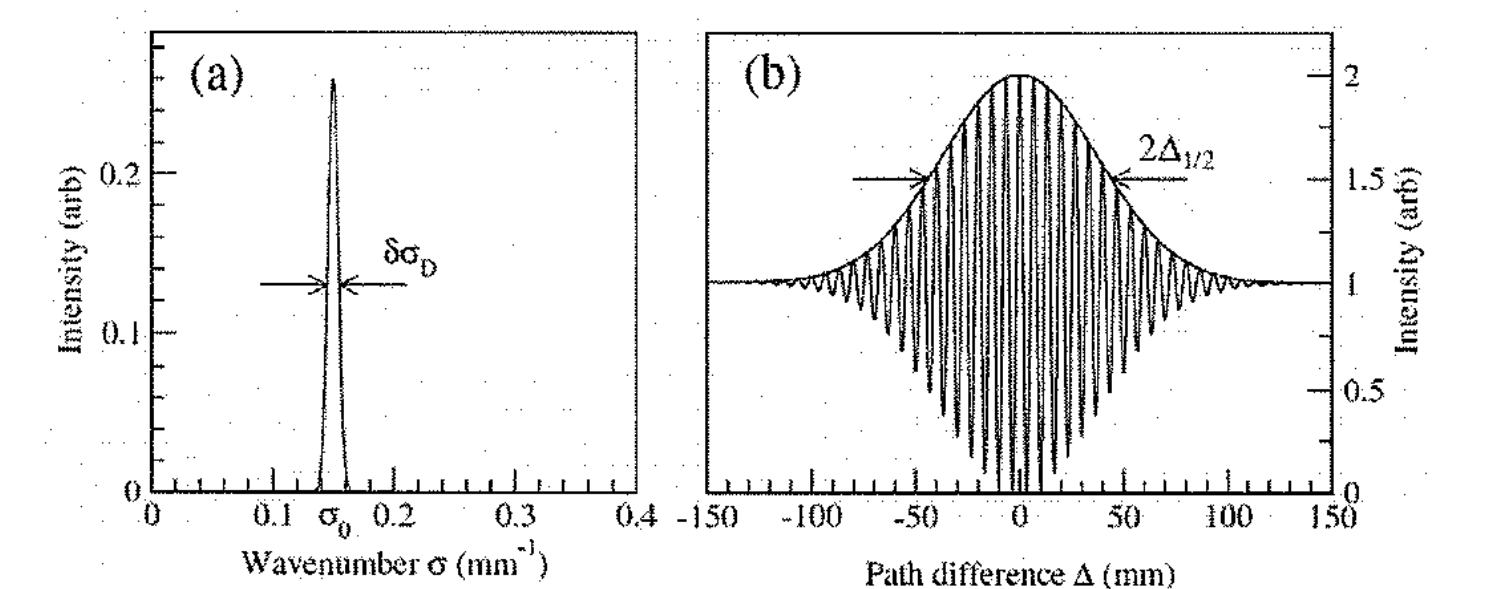
But the problem is that this spectrum is a Gaussian for the intensity in terms of the wavelength $I_S=I_S(\lambda)$ only, not in terms of the wavenumber $I_S(\tilde{\nu})$ so the x-axis is different? Which means diagrams (a), (b) ($\delta \tilde{\nu}=1/ \delta \Delta$)cannot be applied here because $I(\Delta)$ will no longer be a Gaussian (with beats in the profile) since the x-axis is now inverted $\lambda$? How do I find the Gaussian of the inteferogram $I(\Delta)$?

Answer 1

You are correct that $I_s(\nu)$ isn't Gaussian with respect to $\nu$ if $I_s(\lambda)$ is Gaussian with respect to $\lambda$. That said, $I_s(\nu)$ is approximately Gaussian if you have a sufficiently narrow bandwidth.

To see this, consider the Taylor series of expansion of $\lambda = 1/\nu$ around $\lambda_0 = 1/\nu_0$. We see that

$\lambda = \frac{1}{\nu_0} - \frac{1}{\nu_0^2} (\nu - \nu_0) + O((\nu - \nu_0)^2)$

or in other words,

$\Delta \lambda \approx - \frac{\Delta\nu}{\nu_0^2}$

where $\Delta \lambda = \lambda - 1/\nu_0 = \lambda - \lambda_0$ and $\Delta\nu = \nu - \nu_0$.

So when $\Delta\nu$ is small, we can write that

$I_s(\nu) = I_{s0}e^{-\Delta\lambda^2 / (2(\delta\lambda)^2)} \approx I_{s0}e^{-\Delta\nu^2 / (2(\delta\lambda)^2 \nu_0^4)}$

which is Gaussian in $\nu$.

Answer 2

You just have to do a variable substitution $\tilde{\nu} ->\lambda$ in your integral .

From $\tilde{\nu}=1/\lambda$ you get $$\frac{d\tilde{\nu}}{d\lambda} = -\frac{1}{\lambda^2}$$

i.e.

$$d\tilde{\nu} = -\frac{d\lambda}{\lambda^2}$$

and therewith

$$I_\lambda(\Delta)=\frac{1}{2\lambda^2}\int I_S(\lambda)exp(-i2\pi\Delta/\lambda)d\lambda,\quad 0<\lambda<\infty \tag{1}$$

Note that the minus sign has disappeared because the integration limits have been exchanged as well.

As for the example spectrum shown: this does actually not look anything like a Gaussian, as it is clearly not symmetrical.