How can we change the centre of mass of our own body?

Question

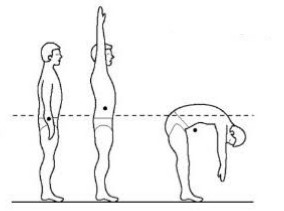
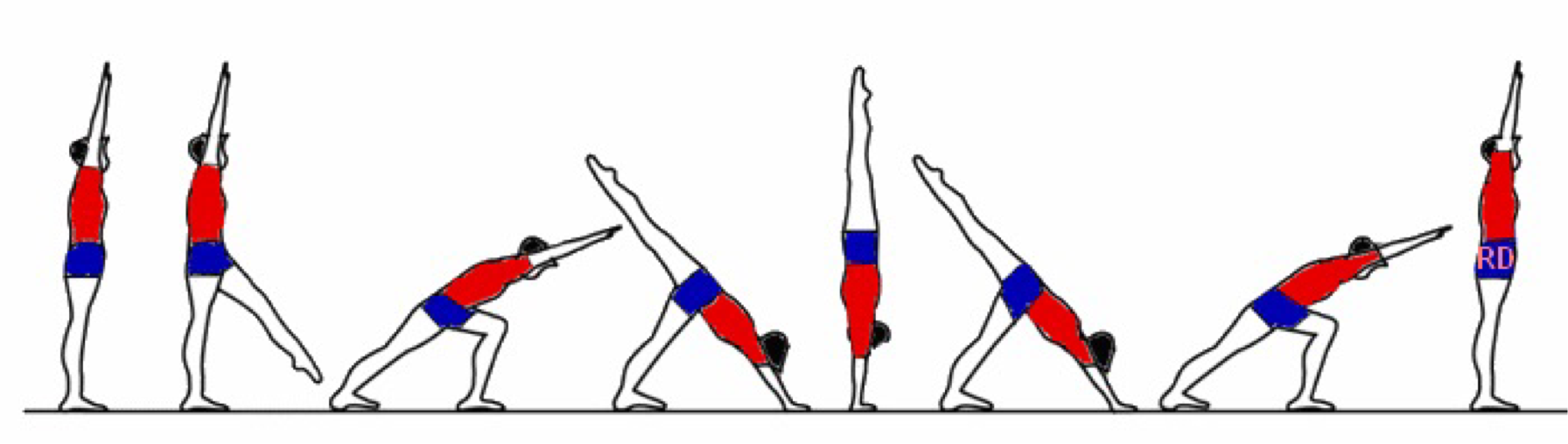
As per my knowledge the centre of mass of a system can only be changed by a net external force acting on the body.

Now imagine that we are in a spaceship (obviously in a pressurized cabin with zero gravity). By the videos that I have seen of astronauts in international space station and other space related videos or movies I know that we can control our body parts movement such as moving our hands and legs while floating in space, without any external aid. This movement of our body should change our centre of mass but How is that possible ?

Answer 1

If the astronaut is in a space ship far from any walls and they iterate their body through the positions (1), (2), and (3) in your figure the following will happen:

• Because there are no external forces acting on the astronaut's body (also a body in the physics sense) the astronaut's center of mass will not move relative to the walls of the space ship.
• However, because the shape of the astronauts body has changed their body parts will in fact move relative to their center of mass. For example, comparing body position (1) and (2), we can see that the astronauts head is closer to their center of mass in figure (2) compared to figure (1). So if the astronaut begins in position (1) and changes their position to figure (2) the astronaut's head will in fact move closer to their center of mass (which doesn't move relative to the spaceship) which means that the astronaut's head moves towards the bottom of the spaceship and away from the top.

Of course here by bottom I mean the part of the spaceship by the astronaut's feet and by top I mean the part of the spaceship by the astronaut's head.

Answer 2

The astronaut can only change the motion of their centre of mass if they push or pull against an external object or transfer some of their momentum to an external object.

If the astronaut is floating in the middle of the cabin out of reach of the walls then moving their arms and legs about slowly will not change the motion of their centre of mass. If they can move their arms quickly enough to transfer momentum to the surrounding air, they can in effect "swim" through the air (I think this would be difficult without using a paddle of some sort). Or they could take off a shoe and throw it away from them - by conservation of momentum, their own centre of mass will move in the opposite direction.

If they are within reach of a wall then they can pull themselves against the wall or push themselves off the wall (the astronaut in your diagram must have his feet fixed to wall in some way). By doing this they are transferring momentum to the spaceship. This will not change the motion of the combined centre of mass of the spaceship and all it contains, but it will change the motion of the astronaut relative to the spaceship.

Answer 3

Center of mass is point in physical body where relative mass distribution about it is in equilibrium, i.e. totals to zero. Say if we choose some axis $x$, and com in this axis $x_{com}$, then applies $$\sum_{i \in x \lt x_{com}} m_i ~~- \sum_{i \in x \gt x_{com}} m_i = 0$$. So com is rather a property of body mass distribution, in simplest case when $\rho = \text {const}$, then this is just a matter of geometrical center of shape. This is what astronauts,gymnasts,acrobats are able to change. If density in body is changing also, you need to integrate it over infinitesimal volumes in body, to get coordinates center of mass : $$ \mathbf {R} ={\frac {1}{M}}\iiint \limits _{Q}\rho (\mathbf {r} )\mathbf {r} dV, $$

Answer 4

you obtain the body center of mass by first partitioning the body to parts (see Fig.) for each parts you know the mass and the center of mass coordinates. you can now calculate the CM components with those equations (2D case).

$$x_{\text{cm}}=\frac {1}{M}\left(\sum_i m_i\,x_i\right)\tag 1$$ $$y_{\text{cm}}=\frac {1}{M}\left(\sum_i m_i\,y_i\right)\tag 2$$

where M is the total body mass $~M=\sum_i m_i~$

now if the position of the parts of the body $~x_i~,~y_i~$ change by apply external force on your feet, hence according to equation (1) and (2) your CM position is also change.

Answer 5

In your examples, the gravity as well as the normal force is acting. Thus you can change your centre of mass dramatically compared to the ground (for instance by lying down).

In outer space, far from everything, you can't lie down. If you do, you will simultaneously pull your legs up. In your example of bowing down, you would in outer space pull your legs upwards while bowing your torsoe downwards. The changes will cancel out and the centre of mass will remain stationary.