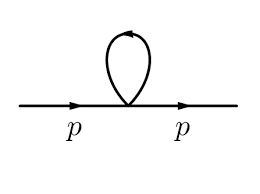
As I see you understand that these kind of diagrams, in massless theories, lead to so-called scaleless integrals, that is they do not depend on any Lorentz-invariant scale. These integrals always "vanish" in dimensional regularization. Indeed, these integrals can always be reduced to factors of
$$
I(d) = \int d^dk~(k^2)^{\alpha},
$$
where $\alpha$ is an arbitrary number. The properties of dimensional regularization include scaling of the measure, so we can conclude for arbitary $s$
$$
I(d) = \int d^d(s k)~(s^2 k^2)^{\alpha} = s^{d+2\alpha} I(d).
$$
Hence $I(d) = 0$, unless $d + 2\alpha = 0$, but since we want $I$ to be continuous $I = 0$. Another, surely more satisfying explanation is found in Analytical continuation in QFT.
But this is not really the whole story: Although scaleless integrals never produce finite contributions, the can produce UV and IR poles. Let us consider the integral ($d = 4-2\epsilon$)
$$
\int d^d k (k^2)^{-2} = - \int d^dk \int_0^{\infty} d\lambda \frac{1}{(k^2 - \lambda)^3} \propto \int_0^{\infty} \frac{d\lambda}{\lambda^{1+\epsilon}}.
$$
Note that $\int_0^{\infty} \frac{d\lambda}{\lambda^{1+\epsilon}}$ does not converge for any $\epsilon$.
As (more properly than here) explained in Analytical continuation in QFT, we can split up the integral
$$
\int_0^{\infty} \frac{d\lambda}{\lambda^{1+\epsilon}} = \int_0^1 \frac{d\lambda}{\lambda^{1+\epsilon}} + \int_1^{\infty} \frac{d\lambda}{\lambda^{1+\epsilon}} = \frac{1}{\epsilon_{\text{UV}}} - \frac{1}{\epsilon_{\text{IR}}},
$$
where we had to take $\epsilon = \epsilon_{\text{UV}} > 0$ in one integral and $\epsilon = \epsilon_{\text{IR}} < 0$ in the other. Dim.reg. does not distinguish between $\epsilon_{\text{UV}}$ and $\epsilon_{\text{IR}}$ so it gives zero. However these poles can still contribute to renormalization, in the case of $\epsilon_{\text{UV}}$, or infrared structure, in the case of $\epsilon_{\text{IR}}$. So they are indeed important, so it is kind of misleading to say that scaleless integrals are zero. More precisely one should say that the produce only pure UV and IR poles (that precisely cancel each other). However any QFT observable is defined with respect to some subtractive procedure to get rid of these divergences, and after this the contributions from these diagrams are truly zero.