Why do IR divergences only appear on external legs of a Feynman diagram in the discussion of Bremsstrahlung?

Question

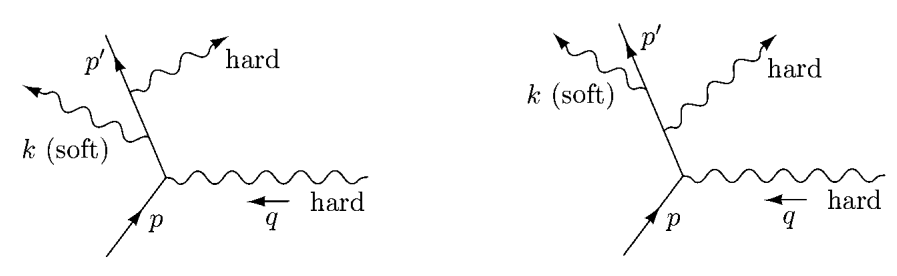
On p. 203 in section 6.5 of Peskin and Schroeder, the diagrams below are given as examples of when an infrared divergence occurs.

'Soft photons' are photons with energy below some cutoff that we impose to regulate infrared divergences. I understand that in order to have an infrared divergence in a Feynman diagram like these, one also needs an on-shell electron coming out of (or going into) the same vertex as the soft photon, since the electron propagator then becomes

$$\frac{1}{(p'+k)^2 - m^2} = \frac{1}{2p\cdot k}$$

which diverges for $k\rightarrow 0$. However the claim in the book is that only the right-hand diagram has on-shell electrons adjacent to the soft photon, which I don't understand. Scattering an electron off of an incoming hard photon can leave us with an on-shell electron, so why should adding another hard photon suddenly force some internal electron off-shell?

It's maybe important to mention that, from what I can tell from the context of this section, the incoming hard photon can be off-shell, since we have been considering electron scattering off of a very heavy target. So then it seems like the intermediate states can be whatever they want, and therefore on-shell in principle.

Answer 1

The outgoing legs are definitely on shell. After all, they travel far and get picked up by the detector.

Ignore the soft photon in the second diagram. Remember we are taking the $k\rightarrow 0$ limit. So we have a vertex where the outgoing hard photon, outgoing electron, and virtual electron meets, and two of them are known to be on shell. Now convince yourself that four-momentum conservation at the vertex forces the virtual electron to be off shell. No exception.

Answer 2

Elaborating on T.P. Ho's correct answer:

1. Given a massive external on-shell particle (an electron) with a non-zero time-like 4-momentum $p^{\prime}\neq 0$ (we can e.g. pick a rest frame where the $3$-momentum ${\bf p}^{\prime}={\bf 0}$ is zero), and

2. given a massless external on-shell particle (a photon) with a light-like 4-momentum $k^{\prime}$,

3. then the denominator of the virtual electron propagator is $$\begin{align} (p^{\prime}+k^{\prime})^2\pm m^2~=~&(p^{\prime 2}\pm m^2)+ k^{\prime 2} +2p^{\prime}\cdot k^{\prime}\cr ~=~&0+0+2p^{\prime}\cdot k^{\prime},\end{align}\tag{1} $$ using the $(\mp,\pm,\pm,\pm)$ Minkowski sign convention, respectively.

4. The virtual electron propagator (1) can be on-shell iff the inner product $$p^{\prime}\cdot k^{\prime}~=~0\tag{2}$$ vanishes.

5. Eq. (2) implies that the photon is soft $$k^{\prime}~=~0,\tag{3}$$ which is a frame-independent statement. (To prove eq. (3) use e.g. the rest frame for $p^{\prime}$.)