Is there an experimental way to observe magnetic quantum number $m_l$ values directly, the way electron spin was detected by Stern Gerlach experiment or proton's spin by nuclear magnetic resonance experiments? The Zeeman effect comes to mind, but in the Zeeman effect one cannot ignore the electron spin. In short, how can one experimentally or spectroscopically see that if $l=2$,, $m_l$ will be -2, -1, 0, +1, and +2?
$\begingroup$
$\endgroup$
7
-
$\begingroup$ A hint to the literature of fine-structure spectroscopists: an $\ell=2$ state is sometimes known as a “quintet” because of its splitting into five sublevels. See also singlet, doublet, triplet, etc. $\endgroup$– rob ♦Commented Oct 10, 2021 at 16:45
-
$\begingroup$ Let us take the example of the famous Na doublet. How does this directly indicate or hint at the $m_l$ value? This doublet is visible without an external magnetic field. Wikipedia on the hand indicates the $m_l$ should appear in the presence of magnetic field hence the name magnetic. $\endgroup$– ACRCommented Oct 10, 2021 at 16:52
-
$\begingroup$ @M.Farooq So just to confirm - you want an experiment that only splits the orbital magnetic components, but does not lift the electron's spin degeneracy? So you want something that only couples to the orbital angular momentum ... ? $\endgroup$– SuperCiociaCommented Oct 10, 2021 at 19:50
-
$\begingroup$ @SuperCiocia, Yes, you are right. $\endgroup$– ACRCommented Oct 10, 2021 at 20:06
-
$\begingroup$ I am going to guess that you cannot, since the electrons will always have spin. So your only hope is to find an atom where the Lande' factors for $L$ is much larger than that for $S$ so that the spin splitting is "hidden"... $\endgroup$– SuperCiociaCommented Oct 10, 2021 at 20:08
|
Show 2 more comments
2 Answers
$\begingroup$
$\endgroup$
3
A hint to the literature of fine-structure spectroscopists: an $ℓ=2$ state is sometimes known as a “quintet” because of its splitting into five sublevels. See also singlet, doublet, triplet, etc.
You suggest in a comment that we consider the famous sodium doublet, which is visible without any magnetic field. That doublet is a spin-orbit effect: the $3p$ first excited state in sodium can have $j=1/2$ or $j=3/2$, depending on the orientation of the electron spin relative to its orbit.
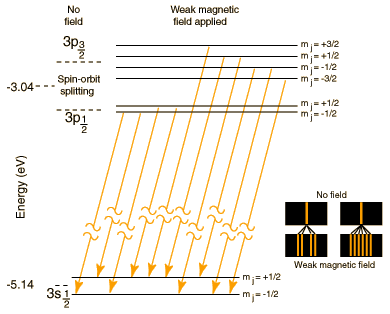(source)
In a magnetic field, the energy degeneracy among the various $m_j$ is broken, and the two lines in the sodium doublet split in ways that reveal their multiplicity. This diagram suggests that the $\frac12 \to \frac12$ transition subdivides into two doublets. The $\frac32\to\frac12$ transition divides into six pieces, rather than eight, because the transition from $m_j=-3/2$ to $m_j=+1/2$ would require the photon to carry away at least two units of angular momentum. (It’s not immediately obvious to me why the six transitions in the $\frac32\to\frac12$ transition should be equally spaced, as sketched, but I’m prepared to believe they are.)
Magnetic splitting was actually discovered by Zeeman in this sodium transition, but this transition is an example of the “anomalous Zeeman effect.” One description.
-
$\begingroup$ In response to clarifying comments on the question: the last link in the answer states that the “normal Zeeman effect” has an $m_\ell$ selection rule, contrasted with the $m_j$ selection rule for the anomalous effect. I don’t have a catalogue of Zeeman transitions handy to hunt for a better example, though. $\endgroup$– rob ♦Commented Oct 10, 2021 at 20:12
-
$\begingroup$ This question was asked by a student in SE Chemistry in an obscure way but it had intrigued me as well. Zeeman effect is indirectly allowing us to see $m_l$ because of the coupling with $m_s$, we have to talk about $m_j$. What about the Stark effect? Does it also allow us to see $m_l$ values? $\endgroup$– ACRCommented Oct 10, 2021 at 21:36
-
1$\begingroup$ The Stark effect seems to cause $s$-$p$ orbital hybridization, which means that $\ell$ is no longer a good quantum number. It seems, on some more reading and some dim recollections, that the Zeeman effect describes the weak-field regime where $m_j$ is a good quantum number but $m_\ell$ and $m_s$ are not. In the strong-field limit, $j$ goes away and you recover separate quantum numbers for $\ell$ and $s$, as first described by Paschen and Back. In an atom with even $Z$, Zeeman/Paschen-Back splitting of $s=0\to0$ transitions directly probes $m_\ell$. Candidate atoms include helium and cadmium. $\endgroup$– rob ♦Commented Oct 10, 2021 at 23:22
$\begingroup$
$\endgroup$
OP wants to observe orbital angular momentum directly without spin angular momentum being involved. The simplest way to do this is if the total spin is zero.
Atomic term symbols make the search easy. An atomic term symbol is of the form $^{2S+1}\!L_J$, where the symbols have their usual meaning. We want a term like this $^{1}\!L_J$, where $J>0$.
Looking at the periodic table here, we see there is no such ground-state atom. One can go to NIST's Atomic Spectra Database and find the levels for helium. There are many excited levels that satisfy our criteria for a term symbol.
Because helium's ground state is $^{1}\!S_0$, we could make an atomic beam out of it and send it straight through a Stern-Gerlach experiment with no deviation. A laser could then be used to excite whatever transition we picked and those excited atoms would deviate in the magnetic field purely due to orbital angular momentum. The lifetime of the excited state will need to be long.
I would not be surprised if an experiment similar to this has already been done.
