What and how are you measuring with $\frac{dy}{dx}$, $dx$, $\mathbf{\nabla} \cdot$ and $\mathbf{\nabla} \times$?

Question

In geometry, the gap between the mathematical model, be it axiomatic or algebraic, and the measurable real world is often non-existent. We have the intuition going from the mathematical model to world and know how to relate quantitative measurement with variables.

It is strange to find an engineering or physics student who does not find trivial the correspondence between the Pythagoras theorem and the measurement of the diagonal of a football field knowing its sides. (That includes finding the value using Pythagoras theorem and check the result by measuring, the units are embedded into the variables)

Precisely, however abstract the mathematics get one can translate the same problem to the real world meaning.

For that I say: The modeling of distances and angles into geometry and vice-versa is intuitive.

That said, I don't have any intuition of the mapping of calculus and the "real world," so even if I become proficient in calculus with $\epsilon$ $\delta$ or Differential Forms, I find myself struggling to apply that knowledge in physics.

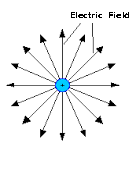
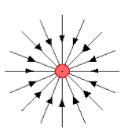
For instance, what are $\frac{dy}{dx}$, $dx$, $\mathbf{\nabla} \cdot \mathbf{F}$ or $\mathbf{\nabla} \times \mathbf{F}$ measure? Or more concretely what do I measure (what measurement) in the real word that correspond directly to the value I am getting from the formulas?

Measuring distances is often relative to a reference, X meters is X times a reference called meter is there and equivalent for the concepts above?

The common interpretation of curls as how the vector field "curls" does little to show how to measure.

Answer 1

Basic calculus is actually very intuitive, because it was invented by Newton & Leibnitz, and (for vector calculus) Heaviside, to model physical problems.

$\frac{dy}{dx}$ is simply the slope of one variable with respect to another. Surely you've encountered the tangent line to a curve? That's a pretty physical concept.

Divergence of a field is simply a measure of how much that field is emanating from a point (or a region), viz.

Positive divergence

Negative divergence

Zero divergence

Curl is a little harder to describe in a post, but here is a great video. https://youtu.be/UzW_jAJzlgI

Short answer is, the physical intuition is out there to be had, you just have to build yours. And it will enable you to read equations and understand what they are saying.

Answer 2

The formulas are representative of easily measurable physicals quantities .

Just take a look at the basic electric components like capacitors or inductors.

Applying a constant voltage to a capacitor is boring: there is zero current and nothing happens. However if you you change the voltage things become more interesting: now all of sudden we see electrons moving (i.e. there is current flowing). It turns out that the current is directly proportional to the rate of change of the voltage, i.e.

$$i = C\frac{dV}{dt}$$

So in this case $dV/dt$ measures directly the amount of current through the capacitor.

The same thing holds for an inductor, just the other way around: the voltage over an inductor is directly proportional to the change (or 1st derivative) of the current.

Things get even more interesting if you combine the two: a change in the capacitor voltage will create a current. That change in current through the inductor will create a voltage change for the capacitor which generates a current change etc., etc. etc. So now we have thing oscillating .

To answer the question directly

Or more concretely what do I measure (what measurement) in the real word that correspond directly to the value I am getting from the formulas?

If you want to measure the derivative of the capacitor voltage, just measure the current.

Answer 3

As others have noted, one can approximately measure derivatives by looking at changes over small amounts of time or space (or even changes in something else, as need be). But there's another way models containing derivatives can be tested with measurements. Suppose a physicist claims some differential equation characterizes a system's behaviour, and you solve that equation (analytically or numerically) subject to appropriate conditions. For example, you might get $x(t)$ from a constraint of the form $F(x,\,\dot{x},\,\ddot{x})=0$. If $x$ behaves as expected empirically, measurements have supported the model even if $\dot{x},\,\ddot{x}$ remain unmeasured.