This question is a prelude to another question I intend to ask.
The following is from MTW Box 10.1 E. Additivity for covariant differentiation:
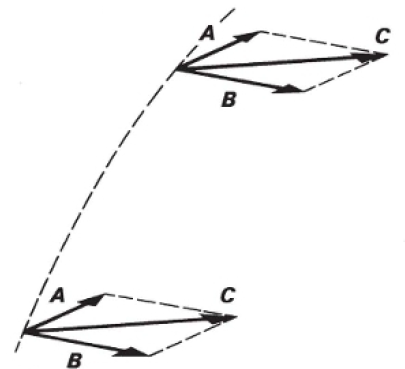
E. In the real physical world, be it Newtonian or relativistic, parallel transport of a triangle cannot break its legs apart: (1) $\mathbf{A},\mathbf{B},\mathbf{C}$ initially such that $\mathbf{A}+\mathbf{B}=\mathbf{C};$ (2) $\mathbf{A},\mathbf{B},\mathbf{C}$ each parallel transported with himself by freely falling (inertial) observer; (3) then $\mathbf{A}+\mathbf{B}=\mathbf{C}$ always. Any other result would violate the equivalence principle!
- Consequence of this (as seen by following through definition of covariant derivative, and by noting that any vector $\mathbf{u}$ can be regarded as the tangent vector to a freely falling world line): $$\mathbf{\nabla}_{\mathbf{u}}\left(\mathbf{v}+\mathbf{w}\right)=\mathbf{\nabla}_{\mathbf{u}}\mathbf{v}+\mathbf{\nabla}_{\mathbf{u}}\mathbf{w}$$ for any vector $\mathbf{u}$ and vector fields $\mathbf{v}$ and $\mathbf{w}.$
- Consequence of this, combined with symmetry of covariant derivative, and with additivity of the "closer of quadrilaterals" $\left[\mathbf{u},\mathbf{v}\right]:$ $$\mathbf{\nabla}_{\mathbf{u}+\mathbf{n}}\mathbf{v}=\mathbf{\nabla}_{\mathbf{u}}\mathbf{v}+\mathbf{\nabla}_{\mathbf{n}}\mathbf{v}.$$
The statement "noting that any vector $\mathbf{u}$ can be regarded as the tangent vector to a freely falling world line", seems problematic. Can't $\mathbf{u}$ be spacelike? In particular, in part E.2. we find $\mathbf{n}$ is used. In other places, Chapter 11, for example, $\mathbf{n}$ is called the geodesic separation; which is pretty clearly spacelike.
Is their "proof" incomplete, and the statement I described as problematic, in fact incorrect?