0
$\begingroup$

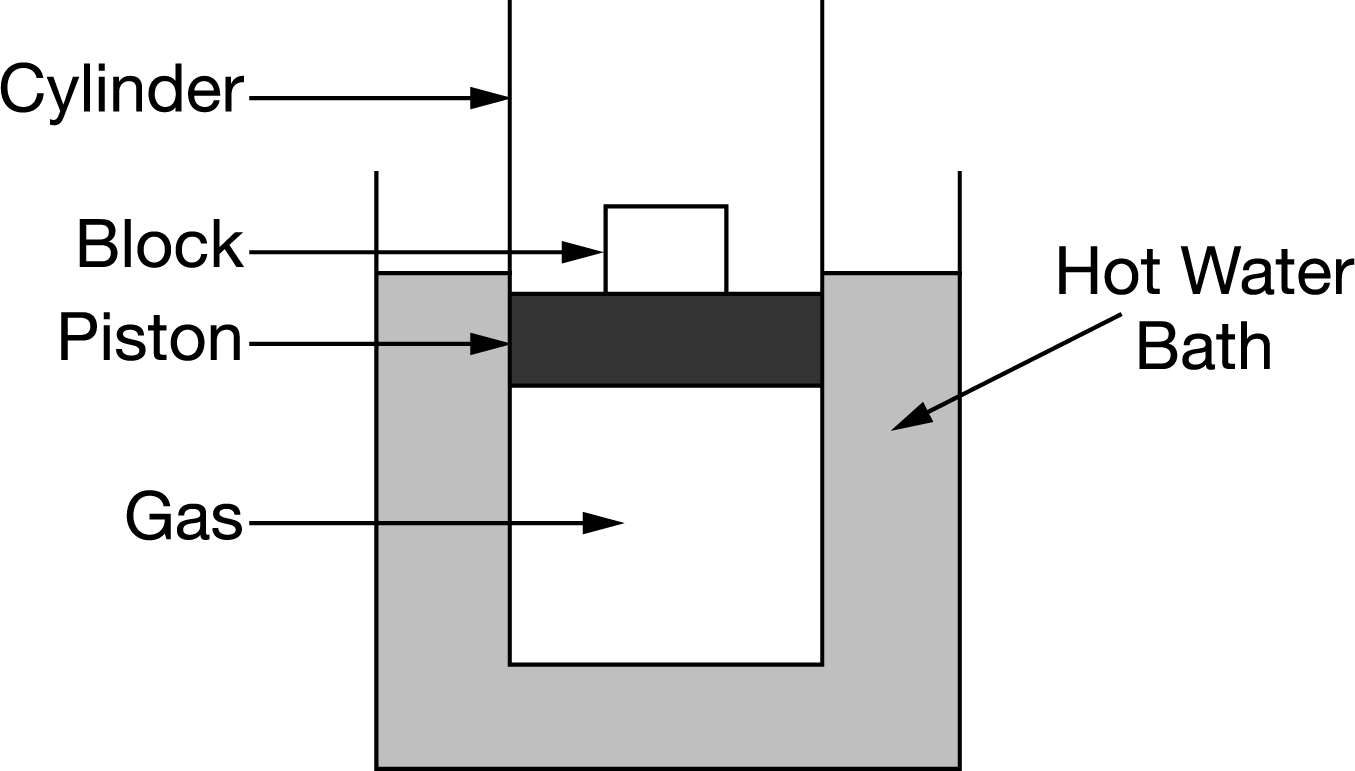
A gas is confined in a closed container with a movable piston. The container is kept in a hot water bath. Blocks of different known masses are placed on top of the piston one at a time, and the gas is allowed to come to equilibrium. The pressure and volume of the gas are recorded each time.

enter image description here

When a $200\ \mathrm g$ block is placed on the piston, the volume of the gas goes from $5×10^{-5}\ \mathrm{ m^3}$ to $4.7\times 10^{−5}\ \mathrm{m^3}$, while the pressure goes from $5.4\times 10^4\ \mathrm{Pa}$ to $5.7\times10^4\ \mathrm{Pa}$. If the surface area of the piston is $1\times 10^{−3}\ \mathrm{ m^2}$, the energy transferred to the gas due to the compression is most nearly:

Answer: $0.006\ \mathrm J$.

Is this the total work or is this specifically the work done by the piston?

Improve this question
$\endgroup$
8
  • $\begingroup$ What is the total work and how do these quantities related? $\endgroup$
    – Liberty
    Commented Oct 6, 2021 at 19:53
  • $\begingroup$ You obviously have some concept in mind. In your opinion, what is the difference between total work and work done by the piston? $\endgroup$
    – David White
    Commented Oct 6, 2021 at 20:02
  • $\begingroup$ If I thought about it by intuition I would conclude that they are the same, but when I estimate a pressure volume graph, then I get that the pistons work is very little compared to the total work. I estimated the total work to be 0.1665 J using triangle and rectangular areas. While the calculated work done by the piston is only 0.006 J. There has to be something I am missing. $\endgroup$
    – Liberty
    Commented Oct 6, 2021 at 21:07
  • $\begingroup$ Maybe they are referring to the net energy transferred to the gas, which also includes heat transferred from the gas to the bath. However, such process would be isothermal, so that then net would be zero. $\endgroup$
    – Chet Miller
    Commented Oct 6, 2021 at 23:34
  • $\begingroup$ @Liberty, it would help if you showed your calculations of piston work and total work. $\endgroup$
    – David White
    Commented Oct 7, 2021 at 0:26

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.