What is light cone? Explain to mathematicians who understand the Lorentz group but not light cone

Question

Mathematically the Lorentz group is precisely the $O(1,3)$ is the 4-vector rotation preserving the inner product of 4-vector under this metric $$ \eta_{\mu \nu}=(+1,-1,-1,-1). $$ There are four distinct sectors of this $O(1,3)$.

Say the 4-vector is $A$ and $B$, then for the Lorentz transformation $R_{n \nu} \in O(1,3)$ on the 4-vector, we have the following invariant inner product of 4-vectors under the Lorentz transformation: $$ A^\mu \eta_{\mu \nu} B^{\nu} ={A^\mu}' \eta_{\mu \nu} {B^\nu}'=A^\mu R^{T}_{\mu m} \eta_{m n} R_{n \nu} B^{\nu} $$

Lorentz group $O(1,3)$ explains the symmetry group of the spacetime at any fixed point.

Built in on this data of Lorentz group $O(1,3)$, how do we explain what is light cone to mathematicians?

Namely what is light cone? mathematically? How to explain to mathematicians who understand $O(1,3)$, but not light cone?

What exactly is this cone of light cone defined mathematically?

Answer 1

Given a four-vector $A^\mu$, define the “interval” associated with $A$ as

$$ \Delta s_A = \eta_{\mu\nu}A^\mu A^\nu = \left(A^0\right)^2 -\vec A{}^2 $$

We say that $A$ is

• “spacelike” if $\Delta s_A < 0$. An example is $(0, \vec A)$.
• “timelike” if $\Delta s_A > 0$. An example is $(A^0, \vec 0)$.
• “lightlike” if $\Delta s_A = 0$.

The “light cone” is the surface formed by all light-like four-vectors. The interval $\Delta s_A$ is preserved by Lorentz transformations.

Answer 2

Light cones can more generally be defined for a curved spacetime: Consider a $4$-dimensional Lorentzian manifold, that is, a $4$-dimensional smooth manifold $\mathcal{M}$ together with a metric $g$ with signature $(+,-,-,-)$. Then we can define for each point $p\in\mathcal{M}$ the "light-cone" $V_{p}\subset T_{p}\mathcal{M}$ to be the set of all "time-like vectors'', where we call a tangent vector at $p$ "time-like", if

$$g_{p}(v,v)>0.$$

In general relativity, one normally assumes that our spacetime manifold $\mathcal{M}$ is "time-orientable", which means that we assume that there exist a overall non-zero timelike smooth vector field on $\mathcal{M}$. Such a vector field $X\in\mathfrak{X}(\mathcal{M})$ allows us to define a past $\mathcal{J}^{-}(p)$ and a future $\mathcal{J}^{+}(p)$ with respect to some spacetime point $p\in\mathcal{M}$ by $$\mathcal{J}^{\pm}(p):=\{q\in\mathcal{M}\mid \exists\text{ path }\gamma:[0,1]\to\mathcal{M}:\gamma(0)=p,\gamma(1)=q\text{ and } g(X,\dot{\gamma})\gtrless 0\}.$$ Using this, we can split the light-cone $V_{p}$ of some point $p\in\mathcal{M}$ into a "future cone" $V^{+}_{p}$ and a "past cone" $V_{p}^{-}$, which contain only those elements of $V$ which are "future-pointing" or "past-pointing" respectively.

Answer 3

The light cone is definied to be the set of 4-vectors $(ct,x,y,z)$ satisfying $$c^2t^2 - x^2 - y^2 - z^2 = 0.$$ Or written in covariant notation $$\eta_{\mu\nu} x^\mu x^\nu = 0.$$

_{(image from Einstein for Everyone - Spacetime)}

Answer 4

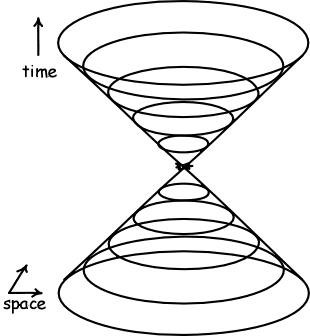
There are two related but different notions of "light cone" (or "null cone"): one in the spacetime [which Minkowski introduced in 1907/1908 as part of his "Space and Time", which introduced the "spacetime viewpoint" and the various terms we use today in relativity], and the other in the tangent space of a spacetime event.

Light Cone in the Tangent Space of an event in Spacetime

The Minkowski Metric on vectors in the tangent space divides the vectors into three types via the sign of the square-norm of the vector. In the (+---)-signature convention, we have timelike, null [or lightlike], and spacelike.... corresponding to positive, zero, or negative. It is said that the light cones encode the causal structure of the spacetime.

The light cone in the tangent space would be defined by the set of all of null vectors (the "lightlike directions"), which would be tangent vectors to geodesics in the spacetime traced out by light signals to or from an event. The tips of these vectors lie on a conical-shaped hypersurface. This cone can also be thought of as the asymptotic surface of the hyperboloids of (say) the future unit-timelike vectors [such a vector is called the 4-velocity, which is tangent to a timelike curve in spacetime that represents the worldline of a particle with nonzero mass]. The light cone therefore represents a finite limiting but unattainable speed for such particle worldlines.]

Some might consider the interior of the cone, which is determined by all of the timelike vectors. Sachs and Wu (in their "General Relativity and Cosmology" AMS 1977 https://web.archive.org/web/20170816045443/http://www.ams.org/journals/bull/1977-83-06/S0002-9904-1977-14394-2/S0002-9904-1977-14394-2.pdf and likely in their "General Relativity for Mathematicians") refer to this as a "solid cone".)

Light Cone in spacetime

The light cone of event O is the set of events reached by lightlike geodesics from O into the past and into the future, which describes the spacetime evolution of a flash of light. (This was Minkowski's use in his "Space and Time" presentation in 1907/1908.)

In some cases, it will look like the typical light cone as we move away from O. However, in some situations the light cone can develop caustics.

For more information, consult, e.g., "Gravitational Lensing from a Spacetime Perspective" by Volker Perlick https://arxiv.org/abs/1010.3416 and https://link.springer.com/content/pdf/10.12942%2Flrr-2004-9.pdf

The interior of the future light cone can essentially be thought of as the "chronological future" reachable by timelike geodesics from O, although there may be subtleties in tying down a more precise definition. (See "Techniques in Differential Topology in Relativity" (1972) by Roger Penrose https://doi.org/10.1137/1.9781611970609 )