Why do we consider the optical path length constant in a Cartesian Oval

Question

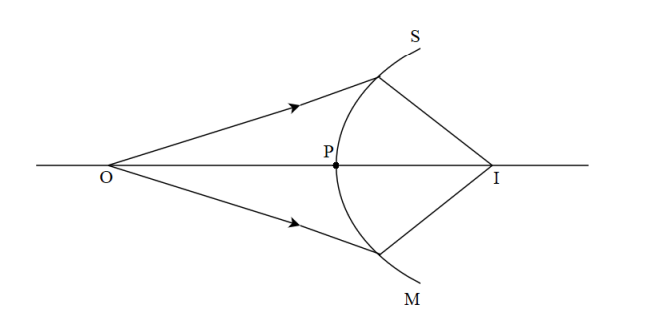
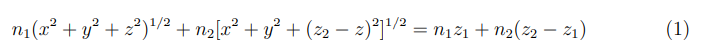I admit that this was from a homework question, but I'm having huge trouble wrapping my head around why the optical path length needs to be the same for a Lens that focuses every single ray emanating from a point to a single point:

Here, if we assume $O$ to be the origin, $P$ to be $(0,0,z_1)$ and $I$ to be $(0,0,z_2)$, the refractive indices of the two media being $n_1$ and $n_2$ respectively (from left to right), then, on equating the optical path lengths for any ray of light that is incident on that surface at $(x,y,z)$, we get the equation of the surface to be:

What I can't understand is why we are equating the optical path lengths, other than the phases of every ray of light reaching point $I$ being the same.

How does it satisfy Snell's law and end up resulting in every light ray reaching the exact same point $I$?

If this is a consequence of Fermat's Principle, please explain to me how it is so, because I'm not able to understand, and everywhere I look, this seems to be taken for granted as an obvious explanation.

If this is already available in another question anywhere, please link me to it and flag as dupe.

Answer 1

There are several but more or less equivalent ways one can express in one sentence all of geometrical optics. Given a piecewise differentiable scalar function $\nu(\mathcal {P})=\nu(x,y,z)$ define the ray vector $\hat {\mathbf{k}}(\mathbf{r})$ as the unit tangent to a path, and define the optical length as the integral $\mathcal{L}[\mathcal{P_2,P_1}] = \int _\mathcal{P_1}^\mathcal{P_2} \nu(x,y,z) \sqrt{dx^2+dy^2+dz^2}$ for an arbitrary path between the fixed points $\mathcal{P_1}$ and $\mathcal{P_2}$. Then:

1. Fermat's Theorem: for any first order infinitesimal variation of the path between the fixed points the variation of the optical length is also zero to a first order.

2. "Geometric" Huygens principle: Denote the infinitesimal length along a path by $d\ell = \sqrt{dx^2+dy^2+dz^2}$ then $d\mathcal{L} = \nu(x,y,z) d\ell$ and the rays are characterized by the vector equation $\nabla\mathcal {L}=\nu \hat {\mathbf{k}}$. Any (optical) ray, $\mathcal{P}=\mathbf{r}(\ell)$ can be equally well defined by its tangent vector $\hat {\mathbf{k}}(\mathbf{r}) = \frac{d\mathbf{r}}{d\ell}$ along the path and then these tangent vectors are the gradients to the "wavefronts", i.e., surfaces defined by $\mathcal {L}(x,y,z)= const$

3. The rays satisfy the vector equation $\hat{\mathbf{k}} \cdot \nabla \times \hat{\mathbf{k}} =0 $ or equivalently, $\nabla \times \nu\hat{\mathbf{k}}=0$

The "more or less" equivalence of these is "just" vector analysis, the physical content is in the associated verbiage.

The existence of surfaces such that $\mathcal {L}(x,y,z)= const$ and are orthogonal to the rays defined by the equation ${\mathbf{k}} \cdot \nabla \times \hat{\mathbf{k}} =0 $ is a generalization of what is called Malus's theorem for homogeneous medium. Snell's law is just the differential expression of $\delta \mathcal{L}=0$ constrained for variations at the interface between two homogeneous media.

The statement that "ideal focusing is just equal path lengths" is from given two wavefronts $\mathcal{L}=c_1$ and $\mathcal{L}=c_2$ the optical path length betwen them is given by any path that can connect these two surfaces via a ray that is cut orthogonally by these surfaces, that is $\Delta\mathcal{L}[2,1]=\int_1^2 \nu d\ell$ and this integral is the same for all ray paths connecting the two wavefronts. Of course, you can expect some mathematical difficulties as you shrink the wavefronts to a single point when trying to represent ideal focusing, and the proper handling of these singularities are outside realm of geometric optics.