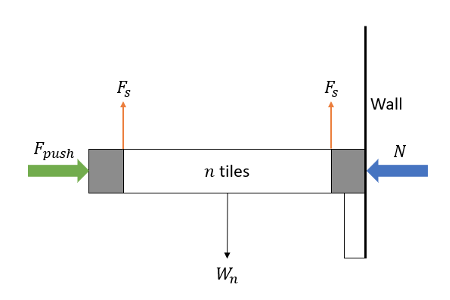
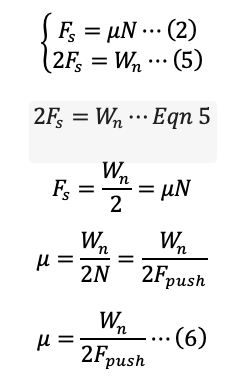0
$\begingroup$

I am currently working on the observation of the relationship between the weight of blocks and the inward force required to sustain the row of blocks. This is similar to the other posts from this site by esdoublef: Free body diagram on a rack of wooden blocks and silverrahul: Horizontal Rack of blocks revisited a.k.a. if it were on top of lava, which block would you choose to stand on?

enter image description here

In my study, I attempted to combine the coefficient of static friction equation and an equation I derived by myself. 2F=W(number of tiles).

Derivation of alternative COF formula

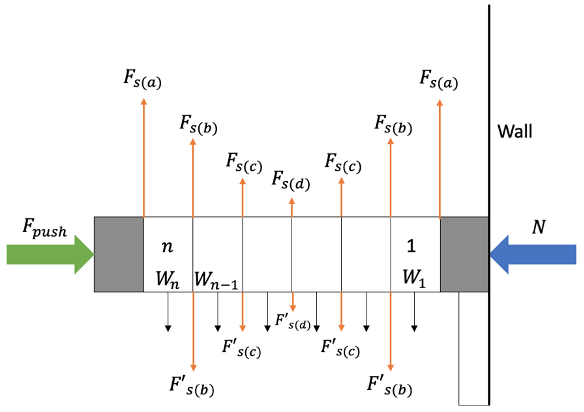
However, this seems to only work for the case in which there is one tile in the middle since there will be action-reaction pairs produced by the frictional forces if more tiles are involved as shown below. What should I do?

enter image description here

Improve this question
$\endgroup$
3
  • $\begingroup$ There is a lot of detail on this kind of thing in the other questions you mentioned, however your equation 6 seems valid for many tiles and gives the limiting coefficient of friction, i.e. if $\mu$ is less than this value, the structure would collapse. $\endgroup$
    – John Hunter
    Commented Jul 17, 2021 at 8:47
  • $\begingroup$ Does this mean that equation 6 is valid despite the answer proposed by esdoublef?<physics.stackexchange.com/a/629343/307029> $\endgroup$
    – Kenji Chng
    Commented Jul 18, 2021 at 7:02
  • $\begingroup$ Final comment, as we have been through this on the other posts: Eqn 6 is valid for the vertical forces if your $W_n$ stand s for the weight of the n blocks between the $F_s$. esdoublef's answer is ok too, and he/she starts to consider the horizontal components. $\endgroup$
    – John Hunter
    Commented Jul 18, 2021 at 10:01

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.