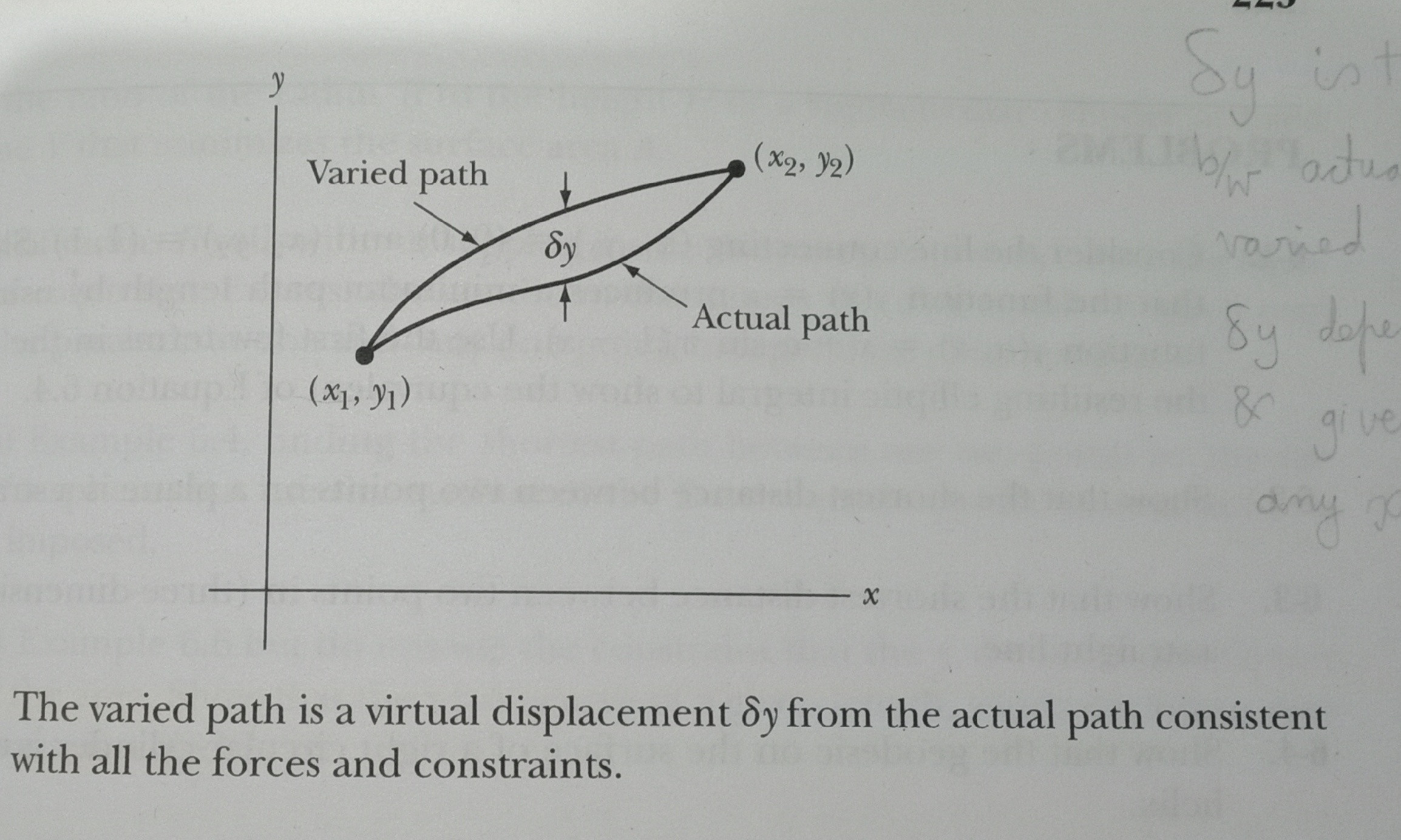
First of all, the first statement is wrong, the condition of being consistent with forces should be eliminated, retaining the consistency with constraints. Because if it is not eliminated, then the first statement is basically saying that the varied path is kinematically admissible, or in your phrase, possible path, which is wrong. So yes, the two statements contradict each other, but that is due to an incorrect definition of virtual displacement in the first statement. In the second statement, the reason why virtual displacements are not possible displacements, in general, is that virtual displacement is considered at a certain moment of time for a system with $dt=0$ while kinematically admissble/possible displacements have to take the change of the constraints with time into consideration. For instance, let suppose there is a particle being able to slide along a straight rigid rod rotating about a fixed point at either its ends. In this case, the virtual displacement vectors can only point along the rod, since by definition, we neglect the rotation of the rod, hence no angular velocity imposed on the particle by the rod. For possible displacements, we need to consider the rotation of the rod and add up the imposed angular velocity and any velocity of the particle on the road. Hence the possible displacement vectors will not point along the rod at the very least since rotation of the constraining rod is considered.