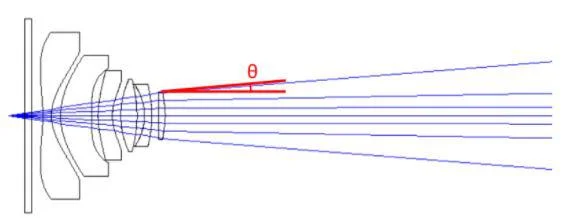
I want to find the angle after the last lens when the beam diffracts. How can I do that? Is it the field of view? But for the field of view formula it takes account the size of the sensor. I want to find the relation of this angle and the origin of the point source. So when I launch the beam from a different distance the angle will change.
If this is a camera with a lens, the angle $\alpha$ you are interested in is really the angle of view, which can be calculated as $$\alpha = arctan \left( \frac{d}{2f} \right) $$ You may have a look here for details about this equation https://en.m.wikipedia.org/wiki/Angle_of_view (for angle of view in photography you have to scroll down a bit). In this equation, $f$ is the focal length of your system and $d$ the physical dimension of the camera chip (width, height or diagonal, depending on what is of interest - your camera has different angles of view in the horizontal and vertical as long as the chip has different width and height). You could even calculate the angle in which a specific pixel is pointing by replacing $\frac{d}{2}$ by the distance of the repective pixel from the center of the chip.
The above equation assumes that the camera chip is in distance $f$ behind the principal plane of your lens, meaning you focus to infinity. If you focus to closer objects it might be necessary to increase the distance between camera and lens in order to get a sharp image. You need to use the real distance between lens and camera in the above equation then.
Actually you could test the correctness of the formula by taking an image of some object of known length (or you measure the object's length).
