1
$\begingroup$

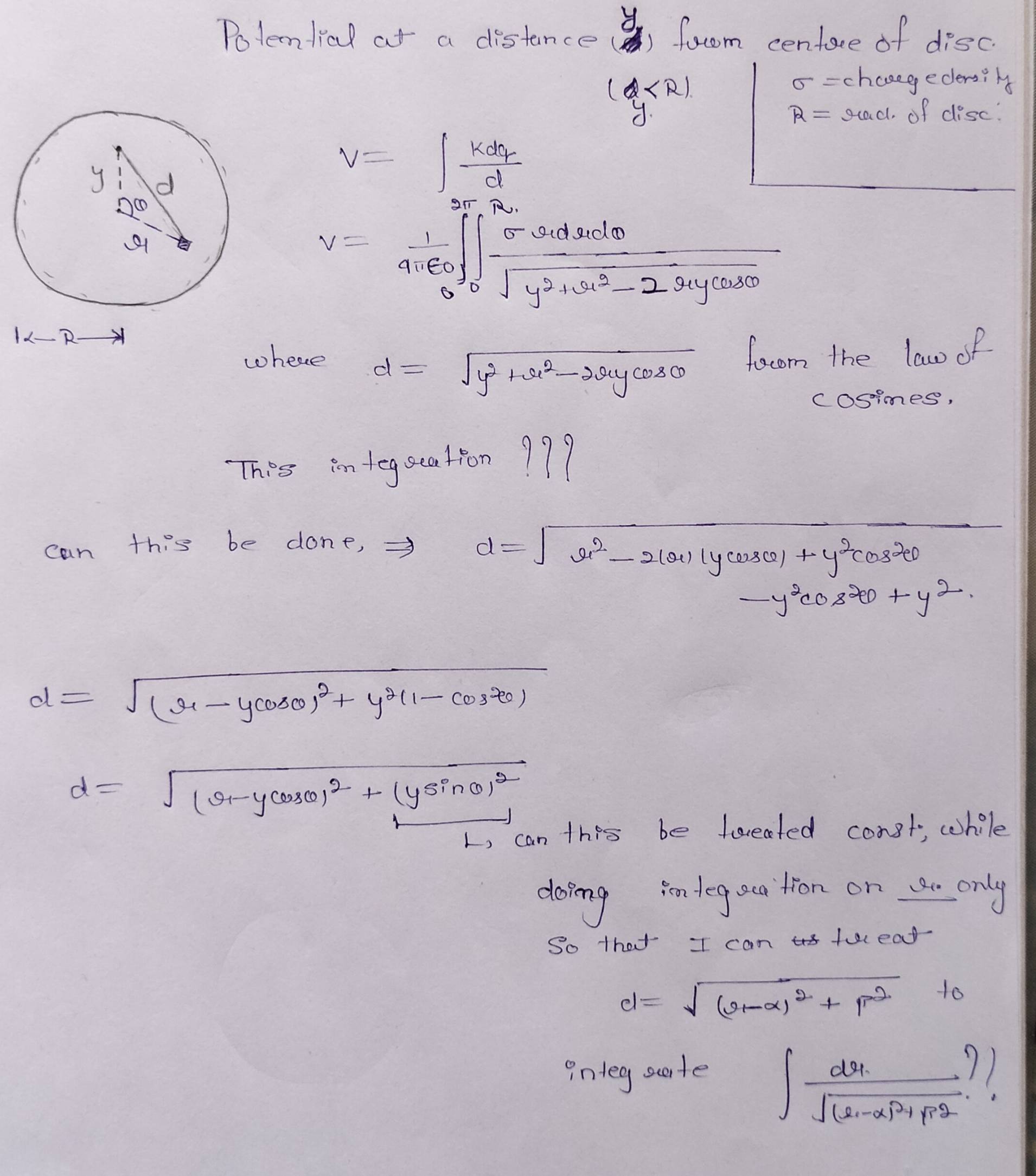
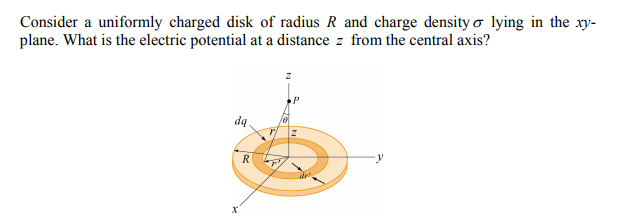
Here, I have attached my try work below. Though I am stuck in integration, but I would like some physics people to answer it.

Can I treat the $y\sin\theta$ factor to be constant while doing integration of $r$, as $y$ is also a fixed radial distance and later integrate $\theta$ while $r$ is done?

Edit: (The disc is placed in x-y plane itself.) And If a better method is available to do such potential calculations, please suggest it.

1

Improve this question
$\endgroup$
4
  • $\begingroup$ This cannot be done in closed form as it involves an elliptic integral. However, the special case where $y=R$ (which is finding the potential at the edge of the disk) can be done analytically, despite even Griffiths using computer numerical integration in his solution. It is extremely tedious but not difficult. However, I have trouble finding the paper on which I did it as it was quite a while ago. If I do manage to find it, I will post it as an answer. $\endgroup$
    – Vincent Thacker
    Commented Jun 25, 2021 at 17:28
  • $\begingroup$ Let me know if you need the closed-form treatment of the edge case. $\endgroup$
    – Vincent Thacker
    Commented Jun 25, 2021 at 17:37
  • $\begingroup$ Should we assume that the disk lies in the x-z plane with its center at the origin? $\endgroup$
    – R.W. Bird
    Commented Jun 25, 2021 at 20:09
  • $\begingroup$ @R.W. Bird No sir, sorry the question was unclear. The disc is placed in the x-y plane and potential is being calculated on a point 'on' the disc. $\endgroup$
    – SHIKHAR CHAMOLI
    Commented Jun 26, 2021 at 6:52

1 Answer 1

Reset to default
0
$\begingroup$

You can use the symmetry of the problem to simplify the integration.

Because $r$, $y$, and $d$ form a right triangle, $d = \sqrt{r^2 + y^2}$.

Because you are at the center of the circle, you can use rings instead of points and find the ring charge $dq$ = $f$($r$, $\text{d}r$) of a circular ring of radius $r$, thickness $\text{d}r$.

Plug that in for $\text{d}q$ in your first integral for $V$, reducing the problem to a simple integration over $r$.

(Equivalently, set up your double integral using $d = \sqrt{r^2 + y^2}$ and solve the $\theta$ portion first.)

You can check your work by making sure that at $y \gg R$, the potential looks like the potential for a point charge $Q$.

1

Improve this answer
$\endgroup$
5
  • $\begingroup$ @g s how do r,y,d form a right triangle, r is constant for a calculation that i understand, but r and theta are variable and theta can be anything from zero to 2pi. $\endgroup$
    – SHIKHAR CHAMOLI
    Commented Jun 25, 2021 at 8:24
  • $\begingroup$ [1 of 2] y is typically at right angles to the charged shape in this kind of question "a distance y (or r, x, s, etc) from the center of a charged disk (or circle, line segment, square, etc)". The question should have included a diagram or wording to be clear, but questions are often not very well framed, especially when textbooks have been asking students the same question for a hundred years. $\endgroup$
    – g s
    Commented Jun 25, 2021 at 15:31
  • $\begingroup$ More importantly, if the question wanted you to evaluate a physical quantity at any displacement $(y,\theta, \phi)$ from the center of the charged disk, it would have to ask for it. The wording of the question implies that the answer can be expressed as a function of y only, but if $(\theta, \phi)$ aren't constants it's clear that no such simplification will be possible. $\endgroup$
    – g s
    Commented Jun 25, 2021 at 15:35
  • $\begingroup$ Were you trying to find the potential at a point on the charged disk itself? That's doable, and expressible as a function of y only, but I doubt that's what was intended. I'll add a picture of how this common question ought to be expressed in my answer above. $\endgroup$
    – g s
    Commented Jun 25, 2021 at 15:52
  • $\begingroup$ Yes sir @g s I was trying to find potential on the charged dics itself. $\endgroup$
    – SHIKHAR CHAMOLI
    Commented Jun 26, 2021 at 6:53

Not the answer you're looking for? Browse other questions tagged or ask your own question.