I know the ground state of hydrogen is unaffected by the Stark effect to first order. And I also know that the 1st excited state is split from 4 degenerate states to 2 distinct, and 1 degenerate state like this:
But I don't quite understand why. I imagine it is something to with (anti)symmetry of the wavefunctions and selection rules.
Can anyone explain?
What happens, essentially, is that the S and P wavefunctions get mixed to produce eigenstates that have shifted centres. This means the atom gets an induced electric dipole moment, whose interaction with the external field either lowers or raises the eigenenergy.
More specifically, consider the wavefunctions of the states $|200\rangle$ and $|210\rangle$:
The first is spherically symmetric, while the second has two lobes where the wavefunction has different signs.
If the field is strong (i.e. stronger than the fine interaction, which separates these two levels, but not strong enough that levels with other $n$ get involved) then the eigenstates will be even mixtures of these, but with different phases, as your diagram indicates.
This should really be done properly, but for an intuitive picture adding equal colours amplifies them while adding opposite colours cancels them, and vice versa for substraction. If you do that, you get something like this:
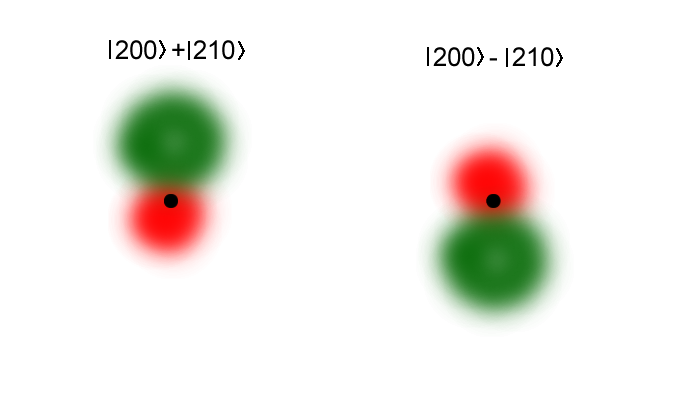Note in particular that the electronic centre of charge has moved from the origin, which means the states have nonzero dipole moments. With the electric field pointing downwards, the state to the left has a lower energy and the one to the right is raised.
Ordinarily these superpositions would "slosh" back and forth between one and the other, as the fine-structure difference in energy between them caused the P state to accumulate phase (as $e^{-iE_{21}t/\hbar}|210\rangle$) slightly faster than the S state. With the field present and pointing downwards, the state to the left stays where it is because the field keeps pulling the electron back. The state to the right is forced to its high-energy position by the requirement that it be orthogonal to the first.
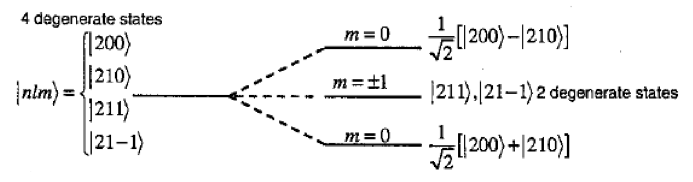
To do this a bit more formally, you just need to state the problem a bit more specifically. Saying that the field is not strong enough to mix subspaces with different $n$ amounts to saying that you are interested in the hamiltonian's structure in the subspace $\mathcal{H}=\text{span}\{|200\rangle,|210\rangle,|211\rangle,|21-1\rangle\}$. The hamiltonian is then fully described by its matrix elements $$ \langle 2l'm'|\hat H|2lm\rangle=E_{2l}\delta_{l'l}\delta_{m'm}+\int \mathrm{d}\mathbf{r} \ \psi^\ast_{2l'm'}(\mathbf{r})E_0 z\ \psi_{2lm}(\mathbf{r}). $$ These integrals you should be able to find yourself, but there are selection rules (which amount to the parity properties of the above integral) that require most elements to be zero. The hamiltonian is thus reduced to the form $$\hat H = \begin{pmatrix} E_{20} &E_0 d&0&0\\ E_0 d& E_{21} &0&0\\ 0&0& E_{21}&0\\ 0&0&0& E_{21} \end{pmatrix} $$ with the basis ordering as above and where $d=\int \mathrm{d}\mathbf{r}\ \psi^\ast_{200}(\mathbf{r}) z\ \psi_{210}(\mathbf{r})$ can be assumed real.
Having done that, saying that the field is strong w.r.t the fine interaction means enforcing the condition that $E_0 d\gg|E_{21}-E_{20}|$, i.e. working in the approximation that $E_{21}\approx E_{20}$. In this approximation the hamiltonian in the subspace $\text{span}\{|200\rangle,|211\rangle\}$ is (up to a change of origin in energy) of the form $\begin{pmatrix}0&1\\1&0\end{pmatrix}$ and the eigenstates are as above. (If the field is not quite as strong then there will be small off-diagonal terms, and the mixing will not be quite as good. For the details, diagonalize! It's relatively easy as it's a simple two-level problem.)
This is really all the formalism one can put into it without overdoing it. To do the problem more rigorously you should expand the physical boundaries of the problem: if the field is weak, consider the effects of fine and even hyperfine structure; if the field is strong then consider mixing of different principal subspaces, and work to higher than first order. Both directions mean quite a bit of work and they do alter the results you quote. (They have to!)
