Work done by the weight when an object moves down

Question

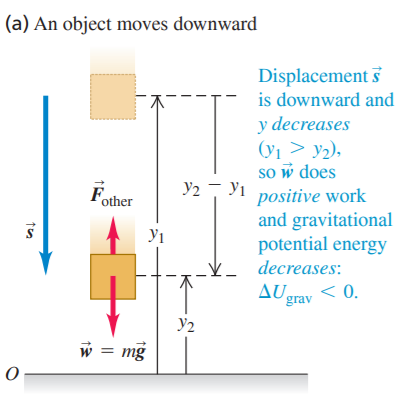
From University Physics with Modern Physics by Young and Freedman, they say:

We want to find the work done by the weight when the object moves downwards from a height $y_1$ above the origin to a lower height $y_2$. The weight and displacement are in the same direction, so the work $W_{\text{grav}}$ done on the object by its weight is positive: $$ W_{\text{grav}} = Fs = w(y_1 - y_2) = mgy_1 - mgy_2 $$

This expression also gives the correct work when the object moves upward and $y_2$ is greater than $y_1$. In that case the quantity $ (y_1 - y_2)$ is negative, and $W_{\text{grav}}$ is negative because the weight and displacement are opposite in direction.

I am confused about this because they have taken displacement, $s$, as $y_1 - y_2$. I've always learnt that displacement $s = y_2 - y_1$ i.e. Final minus initial, not initial minus final.

Is this because they have taken downwards direction as positive? And because down is positive, $w$ is positive, and to make $s$ positive you do $y_1 - y_2$ not $y_2 - y_1$? Am I correct? I've been thinking about this, and this interpretation seems to make sense, but what they've done $s = y_1 - y_2$ is bothering me because for a very long time I've learnt that displacement is final minus initial never the other way around.

Could you also do this?

For the same scenario, take up as positive, then $F = -w = -mg$ and $ W = Fs = -mg(y_2-y_1)$?

Answer 1

what you are thinking is partially correct. In simple terms, work done= force(dot product)displacement. So treating both as vectors, in case 1:- vector of force i.e. mg points downwards which is evident as gravity acts downwards and the displacement vector also acts downwards, now you can explain this with your own thought process that displacement is D(final) - D(initial), where taking D from ground to upwards is =+ve displacement thus displacement here comes to be y1-y2 in upwards direction. Thus work done is -mg.(y1-y2).cos180 as both vectors are in opposite directions and we have taken downwards as negative. Now u know that cos180 is -1 therefore work done is +ve and mgy1-mgy2. Similarly in case 2 the direction of both displacement and gravity vectors interchange and you can check the result for yourself. I hope u have understood if not please revert back.