0
$\begingroup$

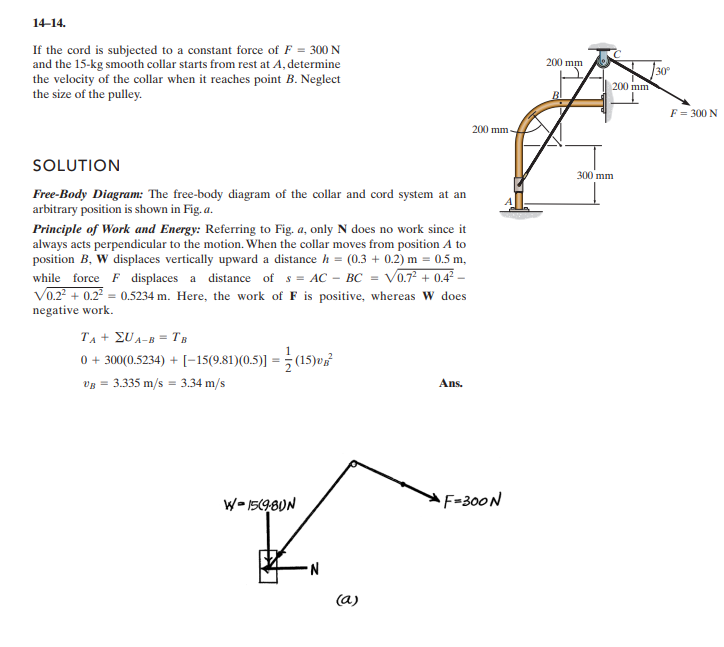
I'm having trouble understanding why work done by a force is calculated in the way as described in the following problem.

Solution of problem

I kind of get why the work done by W is just $mgh$:

$ work = U_{1-2} = \int \vec F \cdot d\vec r = \int_{\vec{r_1}}^{\vec{r_2}}(-W\vec j) \cdot (dx \vec i + dy \vec j + dz \vec k) = \int_{y_1}^{y_2}-Wdy = -W(y_2-y_1)$

So no matter the path, work done by gravity will only depend on the vertical displacement.

I assume that the work done by the cord is calculated the way it is due to a similar explanation, but the understanding sadly eludes me. Does anyone have a satisfying explanation for this?

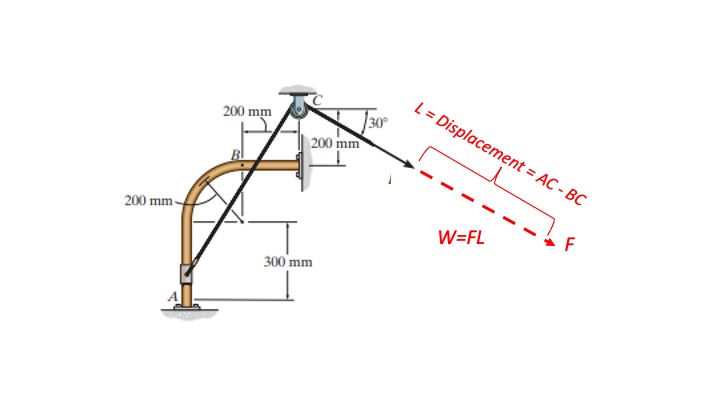
Here is my "proof" for the straight part and how it is dependent on the change in length of the rope from the pulley to the point of contact with the collar.

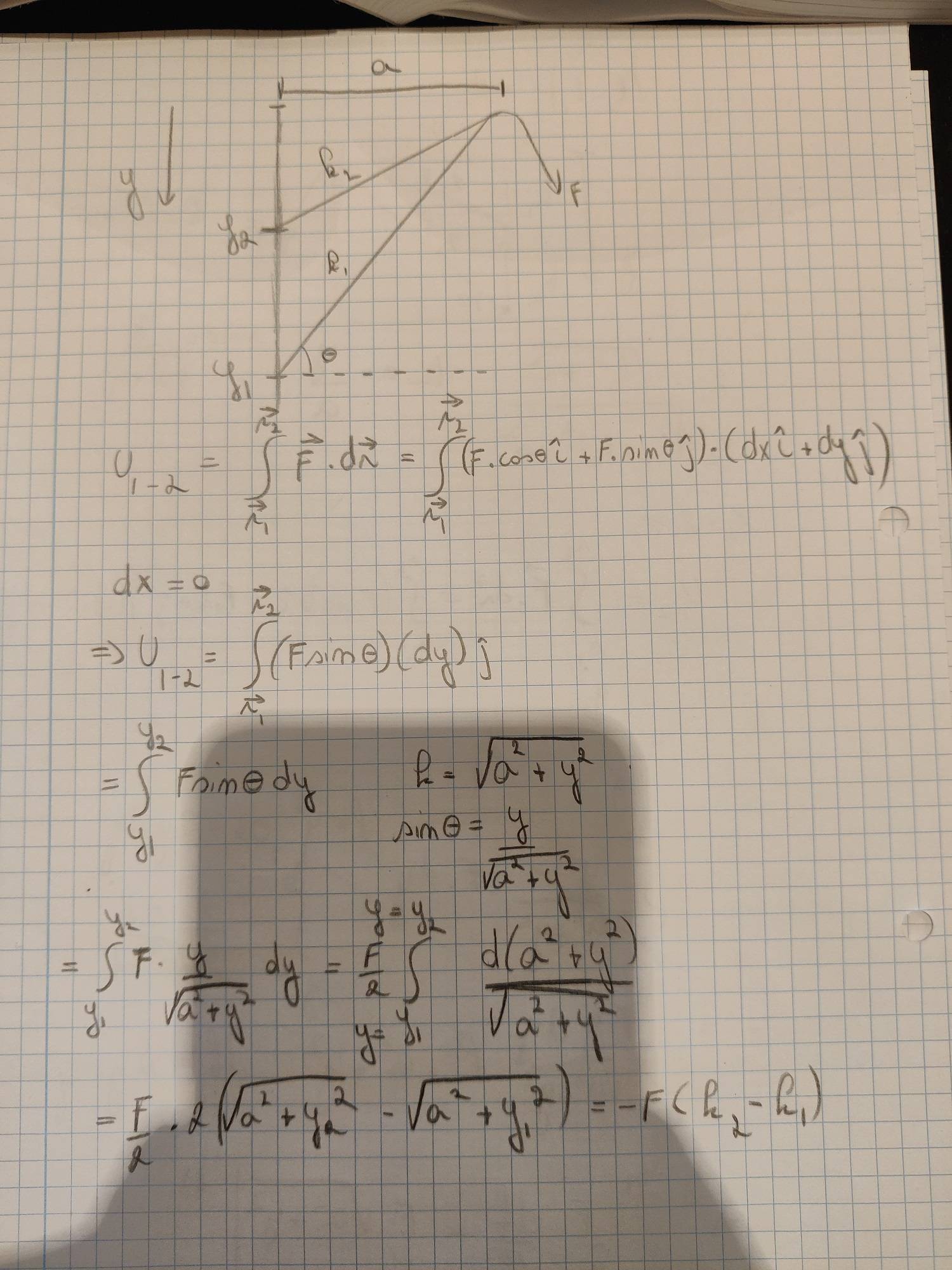
I don't get why the textbook and seemingly everyone else just assumes this as a given without much proof or explanation behind it. Is there an intuitive explanation for it?

Any insights would be greatly appreciated, thank you.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

Since the force on the cord is stated to be constant, then the work by the cord is simply pulling force times the displacement at the point of application of the force, or $W_{cord}=FL$ as shown below.

That work is positive, whereas the work done by gravity is negative. Then per the principle of work and energy (a.k.a. the work-energy theorem), the net work done by the cord and gravity equals the change in kinetic energy of the collar.

Hope this helps.

enter image description here

Improve this answer
$\endgroup$
9
  • $\begingroup$ So we are calculating the work done by the force on a single point in the rope? And this work is equal to the work done at the contact point of the rope with the collar? $\endgroup$
    – ijmert Ulens
    Commented Oct 31, 2023 at 16:59
  • $\begingroup$ Not sure I follow you. The work is the force times the distance it pulls. That distance is the length of the rope that passes C while pulling the rope. Do you understand what I'm saying? $\endgroup$
    – Bob D
    Commented Oct 31, 2023 at 17:04
  • $\begingroup$ Well work always has to act on something right? If you were to calculate the work done by the rope on the collar you'd have to take the integral of the dot product of the force and the displacement (possibly by taking the projection of the force along the displacement with trigonometry). In your explanation I'd think you are calculating the work done by the force on one particular point of the rope (every point of the rope has the same displacement). Because the work done on the rope by the force is the same at every point in the rope, it must also be the work done by the rope on the collar. $\endgroup$
    – ijmert Ulens
    Commented Oct 31, 2023 at 17:29
  • $\begingroup$ since the line of action of the force is in the same direction as the displacement of the rope it is puling on he angle between is zero and the dot product simply becomes Fl.I'm afraid I can't make it any clearer than that. $\endgroup$
    – Bob D
    Commented Oct 31, 2023 at 17:51
  • $\begingroup$ I've added a diagram. $\endgroup$
    – Bob D
    Commented Oct 31, 2023 at 18:19

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.