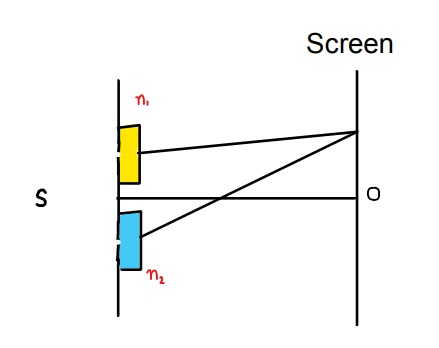
Suppose there are two slabs of refractive indices $n_1$ and $n_2$ kept in front of the sources of light with wavelength λ as shown, how do we calculate the distance of the first bright fringe from the centre O? I really don't understand how to approach. And why is it that only a fixed number of fringes get shifted by the slabs and not all?
Overtime, one could forget the meaning of the phrase 'number of fringes shifted'.
As @José Andrade said in comment elsewhere, imagine a slab of thickness zero mm introduced, this will make no difference, but slowly as we increase the thickness of the slab, the pattern shifts toward either left or right.
If this shift is by an integral multiple of fringe width (we'll call it β), then the new pattern aligns perfectly with the old pattern ass seen in fig 2 of my drawing. So, the first bright fringe is formed at a distance β from the centre of the screen O.
But if the shift is not an integral multiple of β, then the new pattern won't align with the original pattern as seen in fig 3. Hence in this case, the first bright spot will be formed at a distance of {shift}*β from O. Here, {.} indicates fractional part. After this, the question would be easy (I hope)
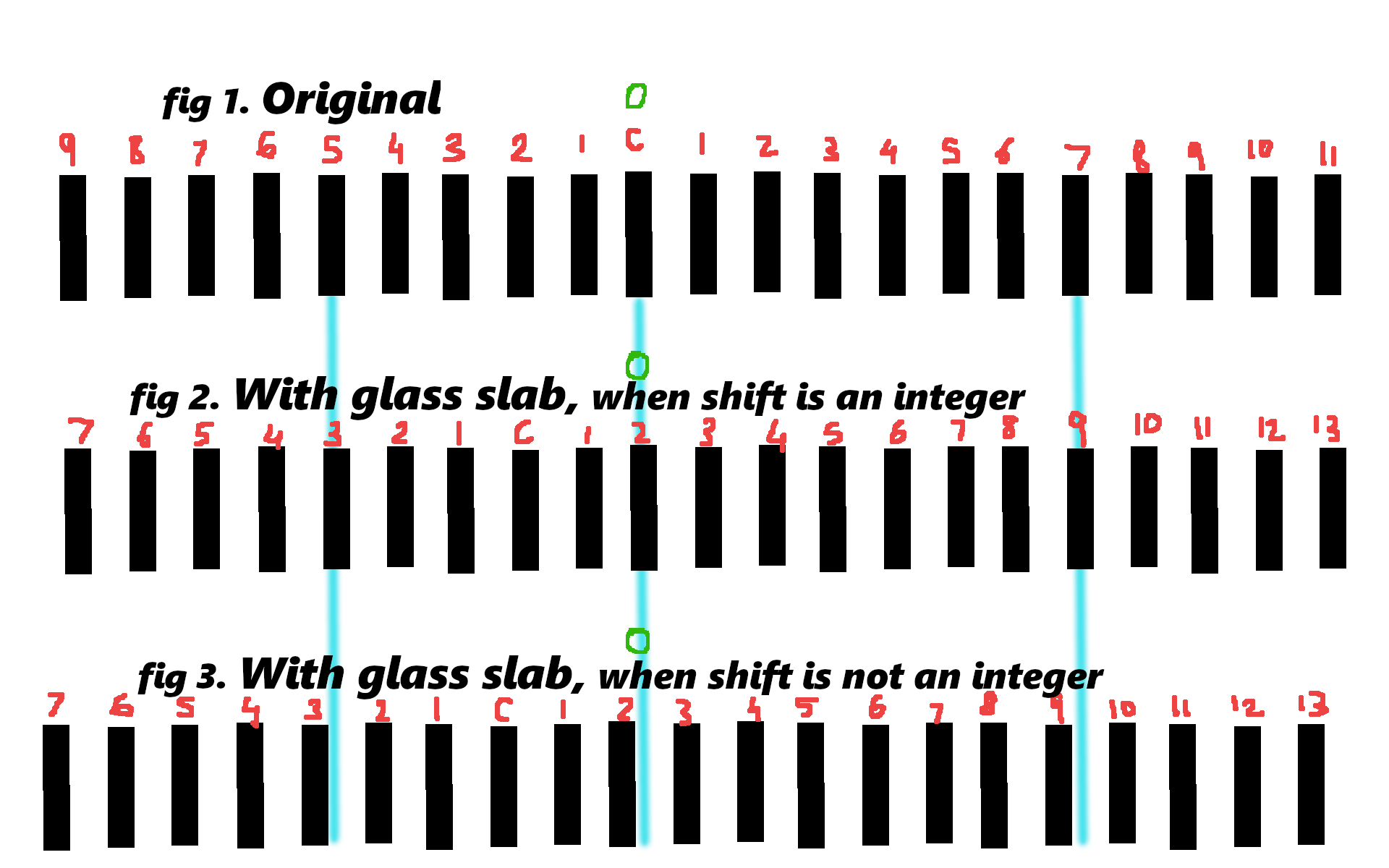Note that the green O in the image represents the centre of the screen.
I put up this question because it bothered me a lot, but after re-examining the concept of introducing a glass slab in the double slit experiment and some helpful comments by the above mentioned person, I finally understood this. This puzzled few of the people I know, although it might be very easy to many. I felt that drawing a diagram and a little explanation could help someone.
