The current I is flowing upward in the wire in this figure. The direction of the magnetic filed due to the current can be determined by the right hand rule.
Can we determine the north and the south of the magnetic field produced by the current I by using a hand rule?
There is no North or South pole in the field around wire. You must understand that the field itself has no poles. Field just consists of field-lines. What we call pole is usually the place when field-lines collide with the physical source of the field (eg. earth, magnet).
But in fact these lines continue even through the source and make elipsoids.
And if you take any field-line of the field, you'll be hardly capable to tell, where the north is.
The concept of magnetic poles only makes sense with respect to permanent magnets/dipoles/solenoids, where the field lines point from one end of the object to the other. In the case of the magnetic field produced by a current, the field lines are in circular alignment around the wire. Hence, there are no endpoints.
You're right with the right hand rule. It's accepted because it agreed with the observations. Placing a magnetic needle (compass) in the influence of the (theorized) magnetic field lines, the compass deflects in the direction of the field indicating the curl. The direction how we twist our fingers show the direction of field. Since we've theorized that the lines of force start at the north pole and end at south pole, of course it can be (it already is) determined by the rule...
So, for a curling magnetic field, there's no specific NS poles. It's curled along the direction of lines.
The concept of magnetic poles is only defined for localized magnetic systems, which include permanent magnets (or equivalently their surface currents) and induction coils.
The reason for this is that the north/south pole description of a magnet is, mathematically, a description of the magnetic (dipole) moment of the system, where the dipole approximation to the field is only valid "away" from the system. If the wire is infinite, you can't be "far" from it.
For loop, surface, or volume currents, respectively, the magnetic moment is defined as $$ \mathbf{m} =\frac{1}{2}\int_C\mathbf{r}\times I\,d\mathbf{l} =\frac{1}{2}\int_S\mathbf{r}\times \mathbf{K}\,dS =\frac{1}{2}\int_V\mathbf{r}\times\mathbf{J}\,dV. $$ If your loop is an infinite wire, the magnetic moment is infinite and its direction depends on where you place your origin. Both of these tell you that this is an incorrect description of your system.
For a simple solenoid, there is a simple rule to get the north and south poles, which is best explained graphically:
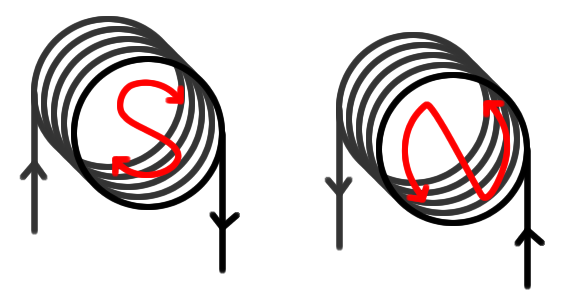
Magnetic field lines come out of the north pole, loop around, and go into the south pole (and you can see that there is no analogue of this for a single long wire!).
Also, as far as this is concerned, solenoids and permanent magnets are much the same. This is because the multiple current-carrying coils of the solenoid look very much like the surface spin currents on a permanent magnet, which are the ones that create its magnetic field. (Where they do differ is in the values of the $\mathbf{H}$ field inside the magnet.)
