Just in case anyone else stumbles on this question, the right-hand rule is really a family of rules that have a specific handedness. The standard first form of this rule is:
If $A \times B = C$ then our standard orientation of 3D space is: take your right hand and point in the direction of $A$ with your index finger. Now orient your hand to point along the direction of $B$ with your middle finger while still pointing at $A$ with your index finger. Then $C$ points along your thumb, if stuck straight-out.
This has a very useful direct interpretation when you have a test charge which is a positive ion with velocity $v$ where the Lorentz force is $q~v \times B = F$, your first finger is the velocity vector, your second finger is the magnetic field, and the resulting force points alongside your thumb.
Of course with an electron you then have to factor its negative charge into this to find an opposite force, or you have to use your left hand which is like using your right hand but with an intrinsic minus sign built-in.
Another application of this rule is in torque $\tau = r\times F.$
But there are two more useful facts to connect this to the rest of the family of rules:
The magnetic field lines of a line-current curl around that line, and
Two parallel currents feel an attractive Lorentz force; two anti-parallel currents (parallel but flowing in opposite directions) feel a repulsive Lorentz force.
Now if you point your thumb along a line of current, your palm (more specifically, the metacarpal bones) puts your fingers (phalange bones) out at an offset and they only curl a certain way around the thumb, counterclockwise if looking at the thumb head-on. So as per (1) the field is either parallel or opposite to the way your hand is curling, and per (2) it's, in fact, parallel. (Proof: Remembering that your left hand carries an intrinsic minus sign, place your left middle finger on top of your right index finger in the opposite direction, to cancel out this minus sign: then when pointing your left index finger parallel to your right thumb, to symbolize a charge moving in the same direction as the line charge, you should see your left thumb point towards your right palm, indicating an attractive force.)
So the second form of this rule is:
Point your right hand's thumb along a line of normal current; the magnetic field curls around it in the same way that your fingers curl about your thumb.
Finally, we have the polarity of magnets, which requires a definition:
- The North pole of a bar magnet is the side that the field lines are coming out of.
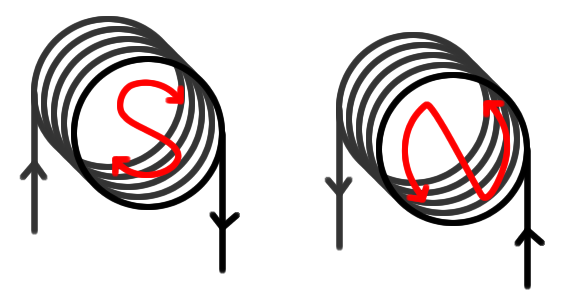
Imagine applying the second form of the right-hand rule to a very large solenoid. In other words imagine placing your right thumb along a big cylinder.
If the current is going clockwise about the cylinder, your fingers will dangle inside, meaning that the field is curling from the outside of the cylinder into its middle; thus your palm is on the South pole of the solenoid. But if the current is going counterclockwise about the cylinder then your fingers will dangle outside, and the field will be curl from the inside of the cylinder out of it: this is the North pole of the solenoid.
Now here's the genius part: think of a solenoid with its North pole pointing up, and curl your right fingers around it in the direction of that counterclockwise current: your right thumb should be pointing straight up, too! That leads to a third form:
Curl your right hand's fingers around a solenoid in the direction of normal current flow; your thumb points in the direction of the North pole and field lines are coming out of your thumb, they arc over the back of your hand, towards the butt (pinky-side) of your hand. Similarly, if you know the North pole of a magnet, point along it with your right thumb and the curl of your fingers describes the currents that are causing that magnetic field.
The thumb more-technically points in the direction of the "magnetic moment" of the current loops that we're talking about, which is more properly defined by torque: a current loop in a magnetic field $B$ feels a torque $\mu\times B$ where $\mu$ is its magnetic moment. This tends to energetically favor the alignment of the two magnetic fields with each other, though since magnetic fields do not do work on their own, it often instead causes Larmor precession. On Earth the magnetic fields point vaguely towards the geographic North Pole, so energy minimization says that if you leave a magnet undisturbed it will point with its "north pole" (magnetic moment) aligned towards Earth's geographic North Pole, hence the name.
(I'm mentioning this because if you really think about it, the fact that the magnetic fields are pointing towards the Earth's geographic-North Pole means that they must enter the Earth at that point, which makes it actually a magnetic-south pole; in other words Earth's magnetic moment points from its North Pole towards its South Pole. In other words, opposite sides attract and like sides repel and all that.)