Consider disc of uniform mass density for simplicity.
Factors on which rotation will depend on frictionless surface:
- Torque about Centre of Mass [COM]
- Direction of Force
There can be $3$ possible cases to apply the Force: Tangentially, Normally & Along a chord of disc
Observe the situations shown in the image carefully.
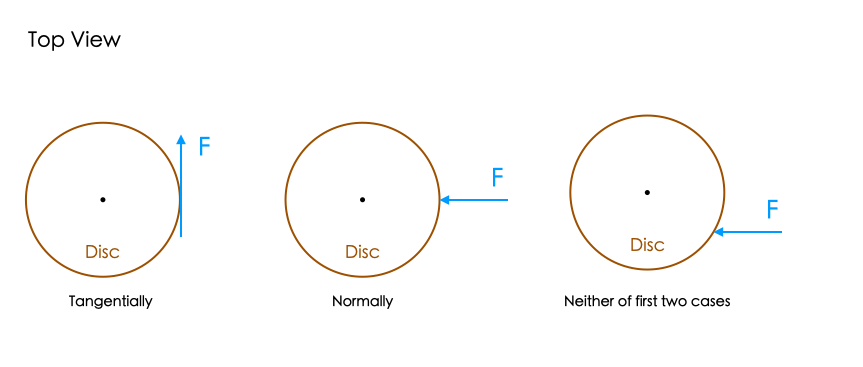
Case I: Tangentially
Considering there the rim has enough friction, when a force is applied to the rim, there is a torque acting about the COM. This torque gives the disc an angular acceleration to rotate.
Case II: Normally
When force is applied normally, which means the line of force passes through the COM, the torque about COM is zero. Therefore, we conclude that no angular acceleration hence, no rotation.
Case III: Along a chord (other than a diameter) of disc
There will be rotation in this case because the line of force do not pass through the COM producing a non-zero torque about the COM. Consequently, producing angular acceleration.
In all cases translation will happen because:
$$F = m.a_{com}$$
where,
$a_{com}$ - linear acceleration

